Mathematics
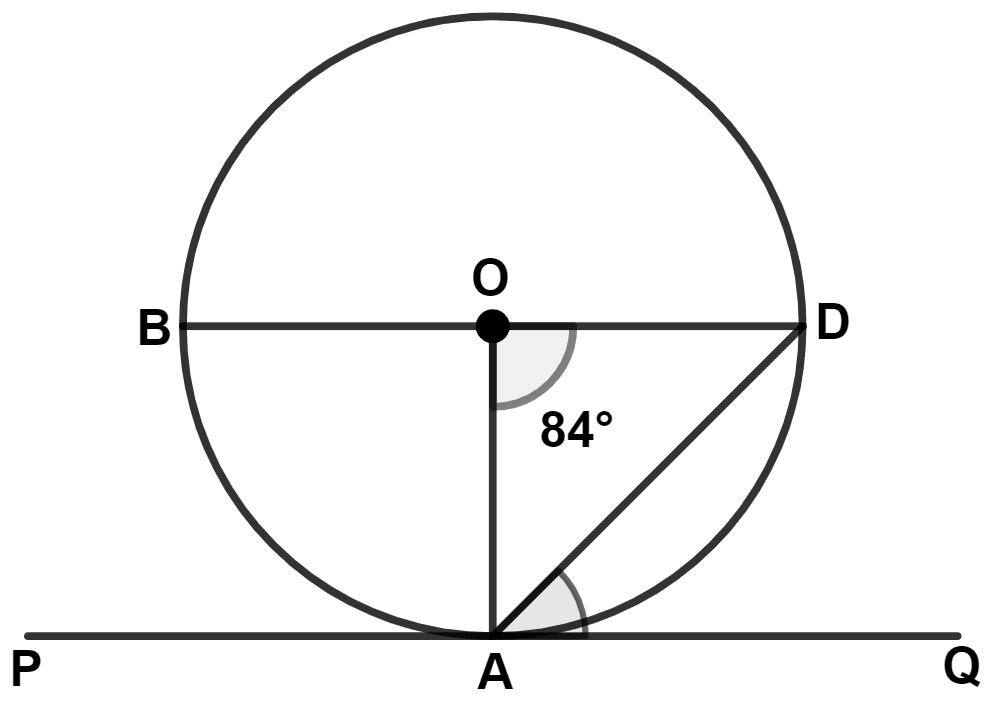
In the given figure O is center, PQ is tangent at point A. BD is diameter and ∠AOD = 84° then angle QAD is :
32°
84°
48°
42°
Circles
2 Likes
Answer
In △OAD,
OA = OD (Radius of same circle)
We know that,
Angles opposite to equal sides are equal.
∴ ∠A = ∠D = x (let)
⇒ ∠O + ∠A + ∠D = 180° (By angle sum property of triangle)
⇒ 84° + x + x = 180°
⇒ 2x = 180° - 84°
⇒ 2x = 96°
⇒ x = = 48°.
From figure,
∠OAD = ∠A = 48°
We know that,
Tangent at any point of a circle and the radius through this point are perpendicular to each other.
∴ ∠OAQ = 90°
From figure,
∠DAQ = ∠OAQ - ∠OAD = 90° - 48° = 42°.
Hence, Option 4 is the correct option.
Answered By
1 Like
Related Questions
In the given figure, AB is tangent to the circle with center O. If OCB is a straight line segment, the angle BAC is :
40°
55°
35°
20°
Two mutually perpendicular tangents are drawn to a circle with radius units. The shortest distance between the two points of contact is :
R units
units
units
2R units
For the three circles with centers A, B and C and radii 5 cm, 2 cm and 6 cm respectively.
Assertion (A) : To find the perimeter of the triangle ABC, add the radii of given three circles.
Reason (R) : The required perimeter is the product of sum of radii by 2.
A is true, R is true
A is true, R is false
A is false, R is true
A is false, R is false
In the given figure, O is center of the circle and PQ is a tangent. If angle OAB = x; the measure of angle ABP; in terms of x, is :
x
180° - 2x
90° + x
90° - x
