Mathematics
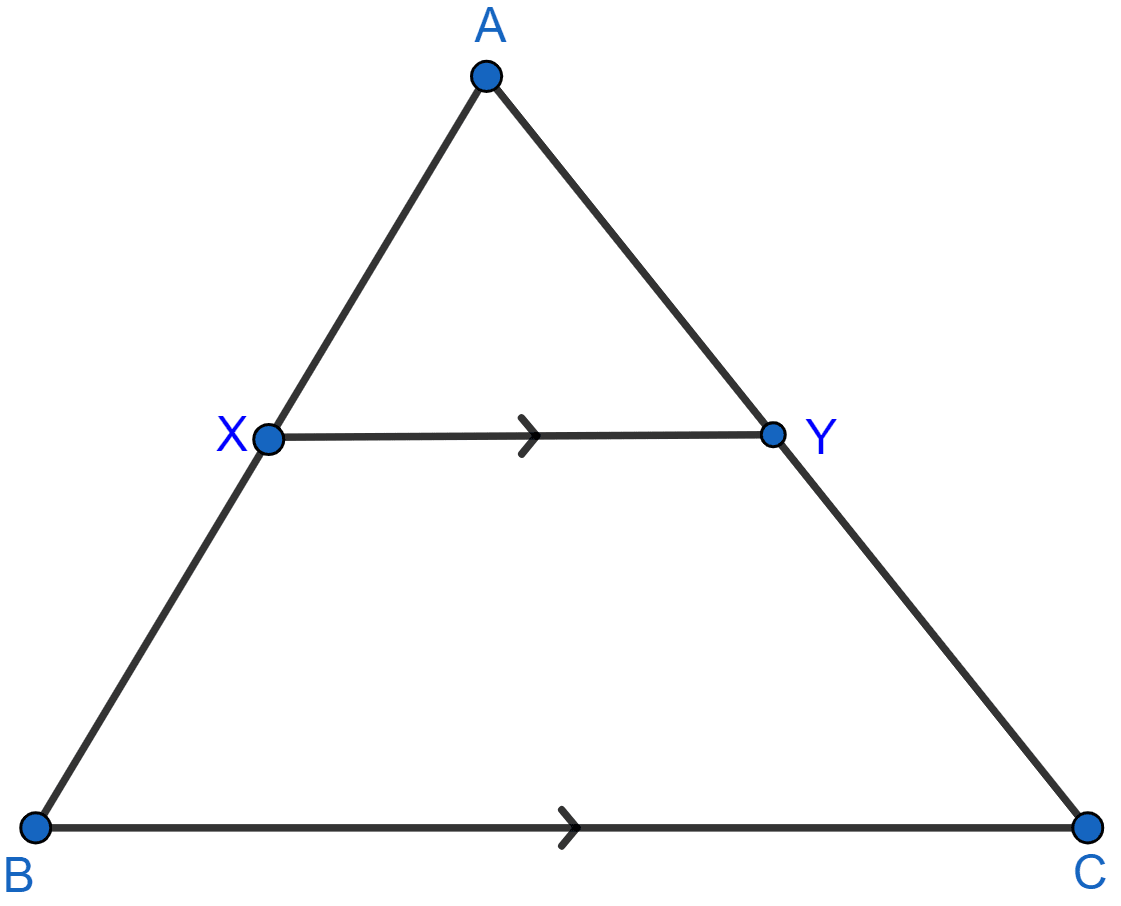
In the given figure, AX : XB = 3 : 5.
Find :
(i) the length of BC, if the length of XY is 18 cm.
(ii) the ratio between the areas of trapezium XBCY and triangle ABC.
Similarity
14 Likes
Answer
(i) Given, AX : XB = 3 : 5
Let AX = 3a and XB = 5a.
From figure,
AB = AX + XB = 3a + 5a = 8a.
(i) In ΔAXY and ΔABC,
As XY || BC, corresponding angles are equal.
∠AXY = ∠ABC and ∠AYX = ∠ACB
∴ ∆AXY ~ ∆ABC [By AA]
Since, corresponding sides of similar triangle are proportional to each other.
Hence, BC = 48 cm.
(ii) We know that,
The ratio of the areas of two similar triangles is equal to the ratio of squares of their corresponding sides.
Let area of ∆AXY = 9b and area of ∆ABC = 64b.
From figure,
Area of trapezium XBCY = Area of ∆ABC - Area of ∆AXY
= 64b - 9b = 55b.
Hence, ratio of area of trapezium XBCY and triangle ABC = 55 : 64.
Answered By
8 Likes
Related Questions
A line PQ is drawn parallel to the base BC of ΔABC which meets sides AB and AC at points P and Q respectively. If AP = PB; find the value of :
(i)
(ii)
The perimeters of two similar triangles are 30 cm and 24 cm. If one side of the first triangle is 12 cm, determine the corresponding side of the second triangle.
ABC is a triangle. PQ is a line segment intersecting AB in P and AC in Q such that PQ || BC and divides triangle ABC into two parts equal in area. Find the value of ratio BP : AB.
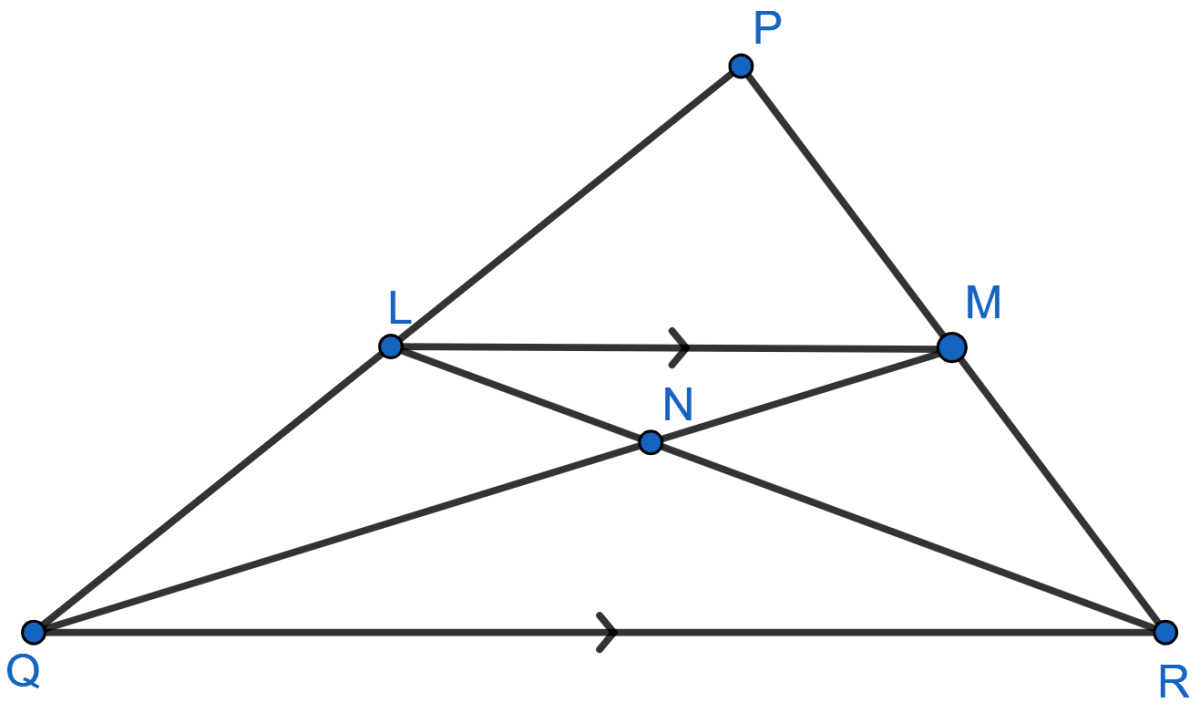
In the given triangle PQR, LM is parallel to QR and PM : MR = 3 : 4.
Calculate the value of ratio:
(i)
(ii)
(iii)