Mathematics
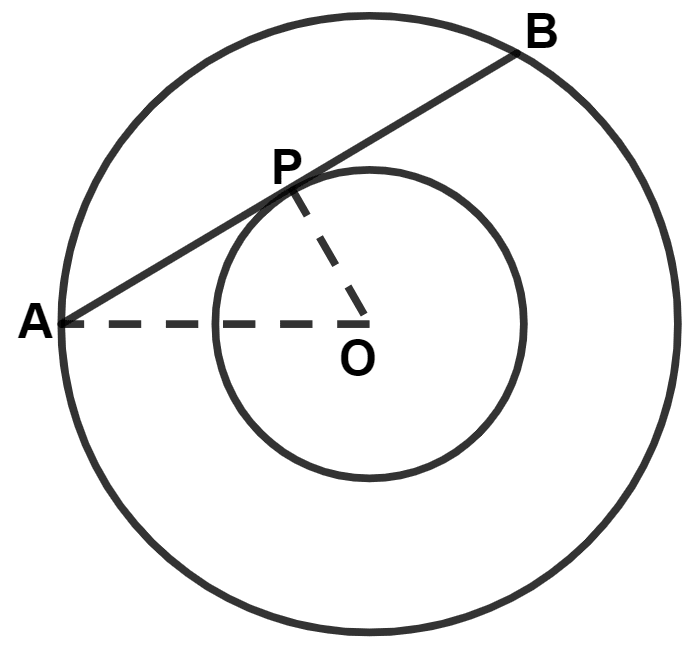
In the given figure, APB is tangent to the inner circle and also a chord of outer circle. Both the circles are concentric. If OA = 10 cm and OP = 6 cm, the length of AB is :
16 cm
10 cm
14 cm
20 cm
Circles
4 Likes
Answer
We know that,
Tangent at any point of a circle and the radius through this point are perpendicular to each other.
∴ OP ⊥ AP
In right angle triangle OAP,
By pythagoras theorem,
⇒ OA2 = OP2 + AP2
⇒ 102 = 62 + AP2
⇒ 100 = 36 + AP2
⇒ AP2 = 100 - 36
⇒ AP2 = 64
⇒ AP = = 8 cm.
Since, AB is the chord to the bigger circle, with center O.
We know that,
Perpendicular from center to the chord, bisects it.
∴ PB = AP = 8 cm.
AB = AP + PB = 8 + 8 = 16 cm.
Hence, Option 1 is the correct option.
Answered By
3 Likes
Related Questions
In the given figure, PA, PB and QR are tangents to a circle. If perimeter of the △PQR = 18 cm, the length of tangent PA is :
18 cm
27 cm
9 cm
none of these

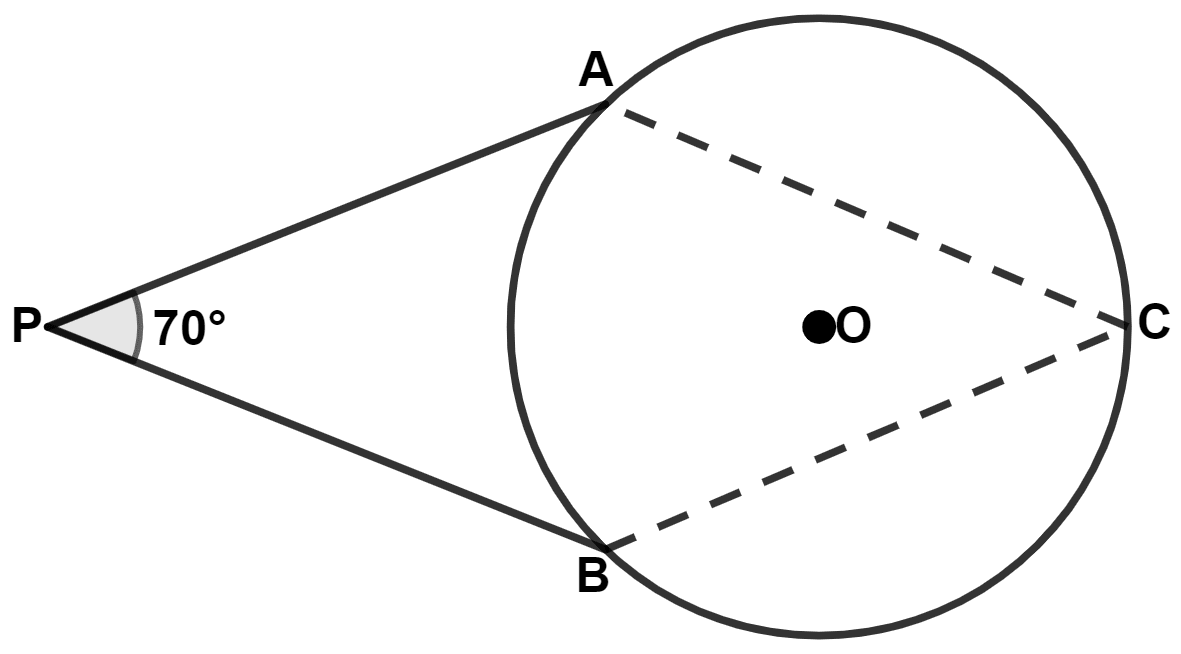
PA and PB are tangents to a circle with center O. If angle BPA = 70°, the angle ACB is :
70°
105°
140°
55°
A, B and C are three circles which touch each other as shown. Using the information, given in the diagram, we find the length AB as :
6 cm
17 cm
(289 - 9 - 2) cm
11 cm

BC is a tangent to the circle with center O. OD is radius of the circle. If ∠DOC = 100°, ∠B is equal to :
50°
60°
40°
70°