Mathematics
In the given figure, AE is the diameter of the circle. Write down the numerical value of ∠ABC + ∠CDE. Give reasons for your answer.
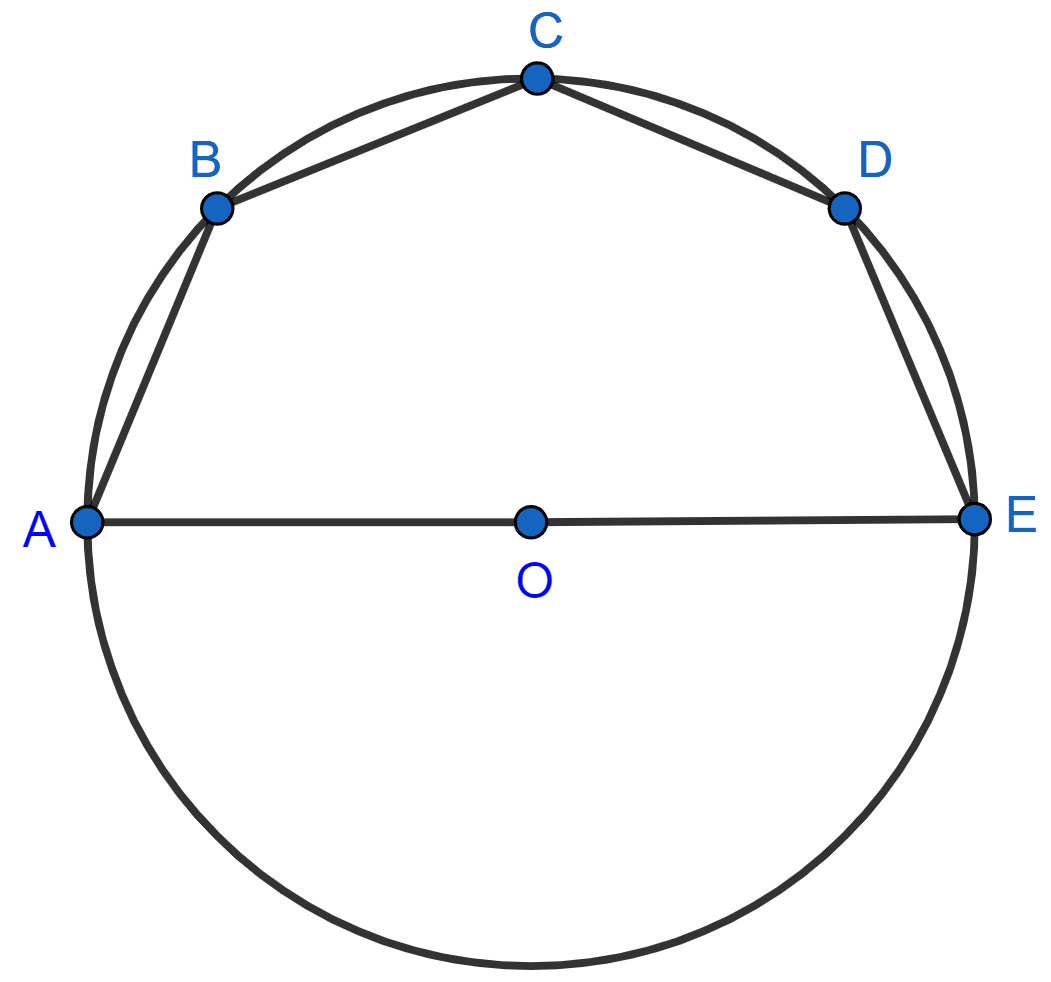
Circles
27 Likes
Answer
Join OA, OB, OC, OD.
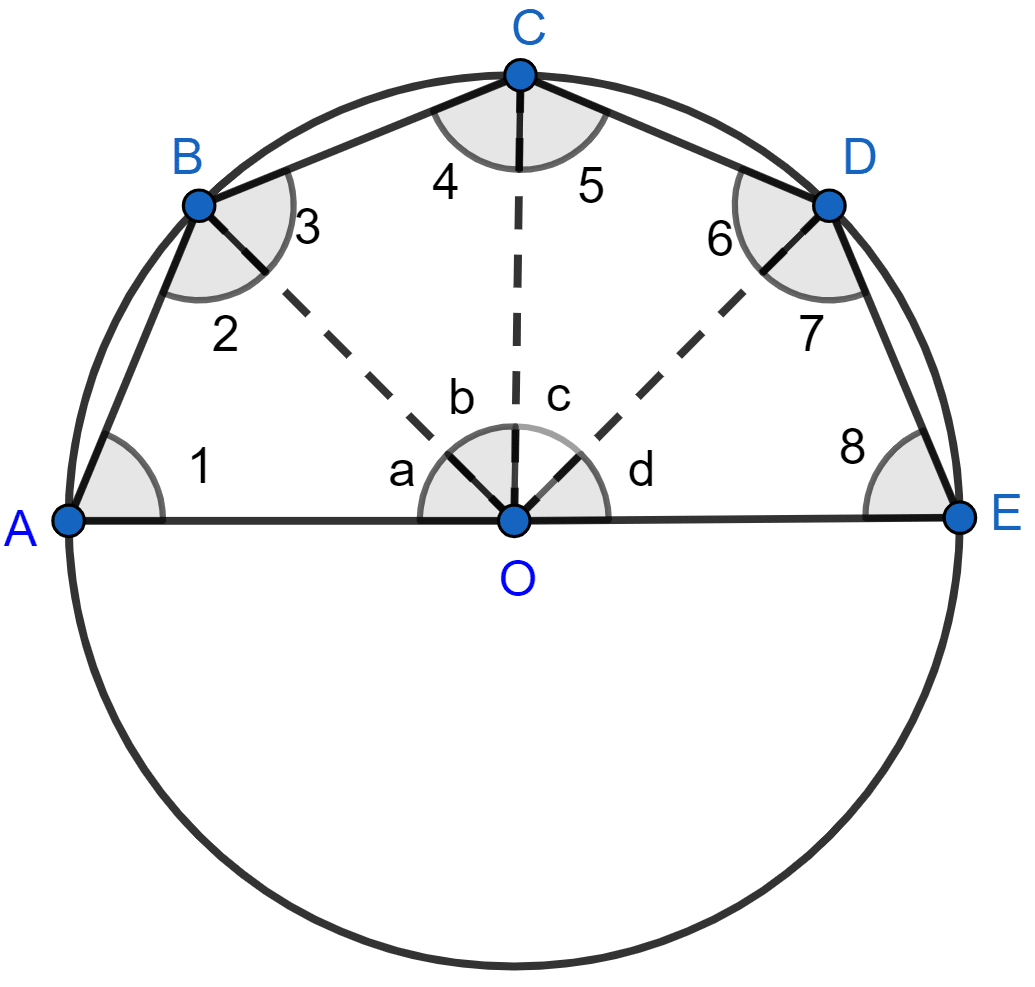
In △OAB,
OA = OB [Radius of same circle]
∠1 = ∠2.
In △OBC,
OB = OC [Radius of same circle]
∠3 = ∠4.
In △OCD,
OC = OD [Radius of same circle]
∠5 = ∠6.
In △ODE,
OD = OE [Radius of same circle]
∠7 = ∠8.
In △OAB,
⇒ ∠1 + ∠2 + ∠a = 180° [By angle sum property of triangle] ……….(1)
In △OBC,
⇒ ∠3 + ∠4 + ∠b = 180° [By angle sum property of triangle] ……….(2)
In △OCD,
⇒ ∠5 + ∠6 + ∠c = 180° [By angle sum property of triangle] ……….(3)
In △ODE,
⇒ ∠7 + ∠8 + ∠d = 180° [By angle sum property of triangle] ………(4)
Adding (1), (2), (3) and (4) we get,
⇒ ∠1 + ∠2 + ∠a + ∠3 + ∠4 + ∠b + ∠5 + ∠6 + ∠c + ∠7 + ∠8 + ∠d + = 180° + 180° + 180° + 180°
⇒ ∠2 + ∠2 + ∠a + ∠3 + ∠3 + ∠b + ∠6 + ∠6 + ∠c + ∠7 + ∠7 + ∠d + = 720°
⇒ 2∠2 + 2∠3 + 2∠6 + 2∠7 + ∠a + ∠b + ∠c + ∠d = 720°
⇒ 2[∠2 + ∠3] + 2[∠6 + ∠7] + 180° = 720° [As ∠a + ∠b + ∠c + ∠d = 180°]
⇒ 2∠ABC + 2∠CDE = 540°
⇒ ∠ABC + ∠CDE = 270°.
Hence, ∠ABC + ∠CDE = 270°.
Answered By
20 Likes
Related Questions
Calculate the angles x, y and z if :
In the given figure, AC is the diameter of the circle with center O. CD and BE are parallel. Angle ∠AOB = 80° and ∠ACE = 10°. Calculate :
(i) Angle BEC,
(ii) Angle BCD,
(iii) Angle CED.

In the given figure, AOC is a diameter and AC is parallel to ED. If ∠CBE = 64°, calculate ∠DEC.

Use the given figure to find :
(i) ∠BAD
(ii) ∠DQB.
