Mathematics
In the given figure, AC is a transverse common tangent to two circles with centres P and Q and of radii 6 cm and 3 cm respectively. Given that AB = 8 cm, calculate PQ.
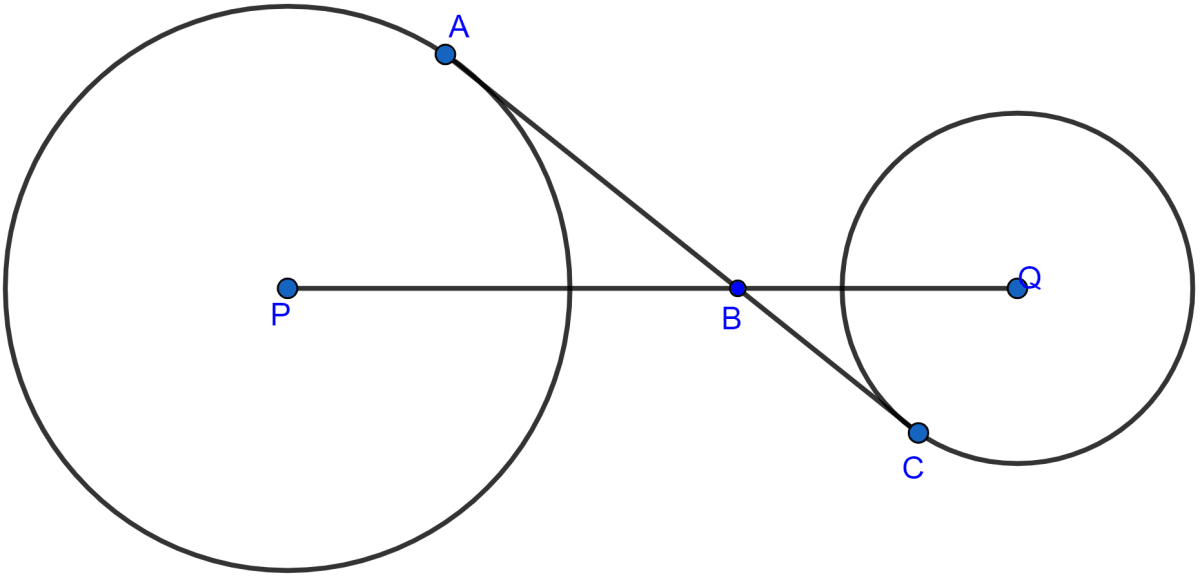
Circles
31 Likes
Answer
Join AP and CQ.
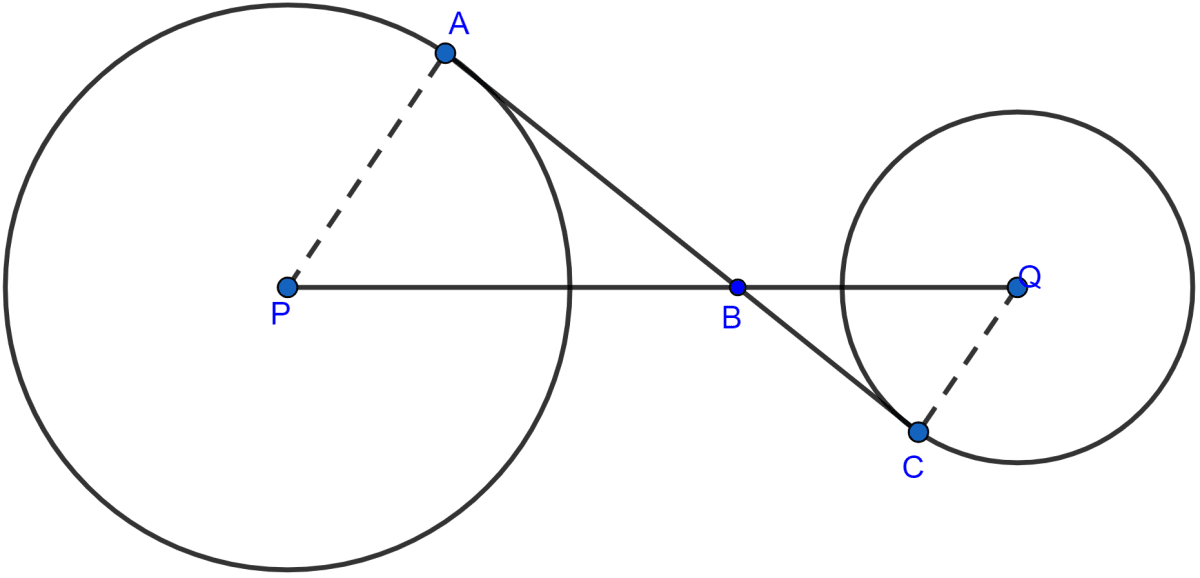
AB ⊥ AP (∵ tangent at a point and radius through the point are perpendicular to each other.)
In right angled triangle PAB.
Considering △PAB and △BCQ,
∠A = ∠C (Each are equal to 90°)
∠ABP = ∠CBQ (Vertically opposite angles are equal)
△PAB ~ △BCQ by AA axiom.
Since triangles are similar hence, the ratio of their corresponding sides are equal.
From figure,
PQ = PB + BQ = 10 + 5 = 15 cm.
Hence, the length of PQ = 15 cm.
Answered By
20 Likes
Related Questions
The length of the direct common tangent to two circles of radii 12 cm and 4 cm is 15 cm. Calculate the distance between their centres.
Calculate the length of a direct common tangent to two circles of radii 3 cm and 8 cm with their centres 13 cm apart.
Two circles with centres A, B are of radii 6 cm and 3 cm respectively. If AB = 15 cm, find the length of a transverse common tangent to these circles.
In the figure (i) given below, PA and PB are tangents at the points A and B respectively of a circle with centre O. Q and R are points on the circle If ∠APB = 70°, find
(i) ∠AOB
(ii) ∠AQB
(iii) ∠ARB
