Mathematics
In the given figure, AB and XY are diameters of a circle with center O. If ∠APX = 30°, find :
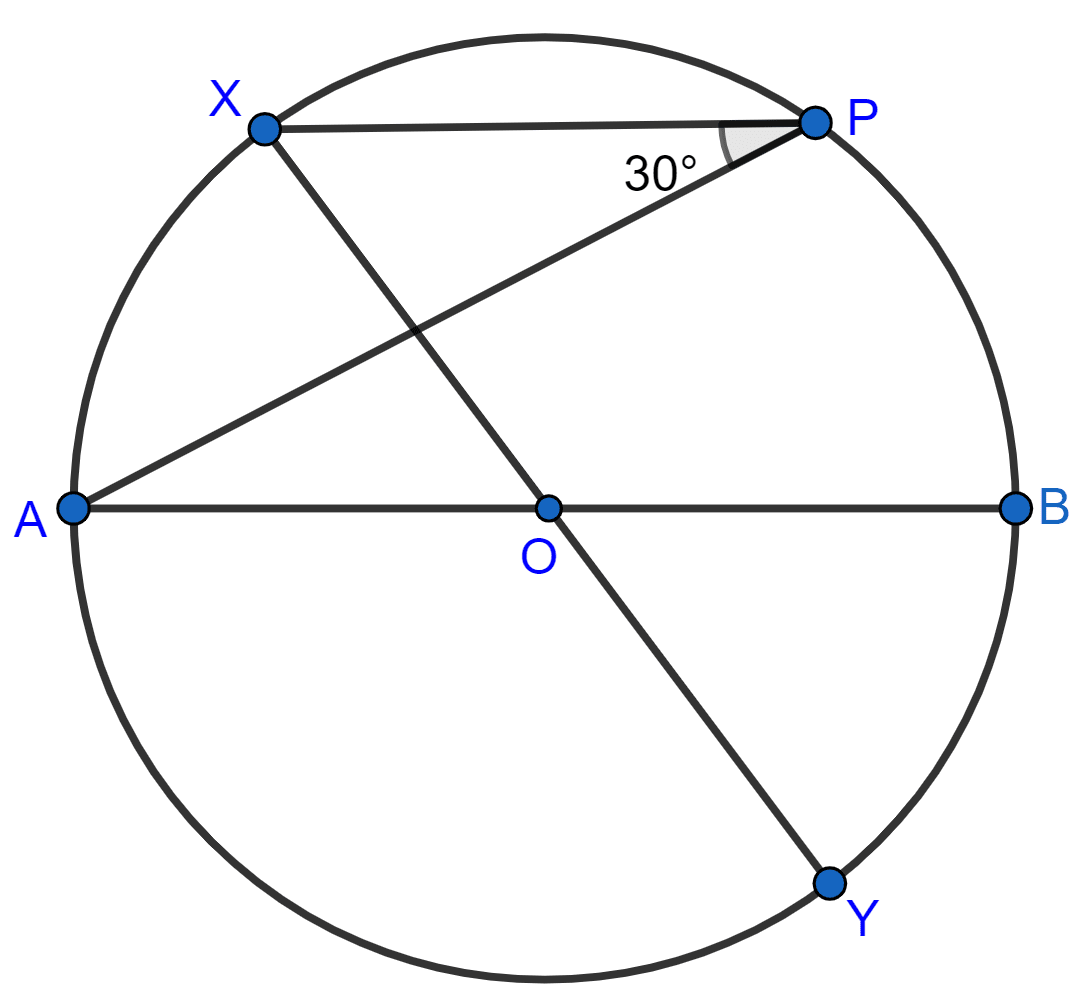
(i) ∠AOX
(ii) ∠APY
(iii) ∠BPY
(iv) ∠OAX
Circles
6 Likes
Answer
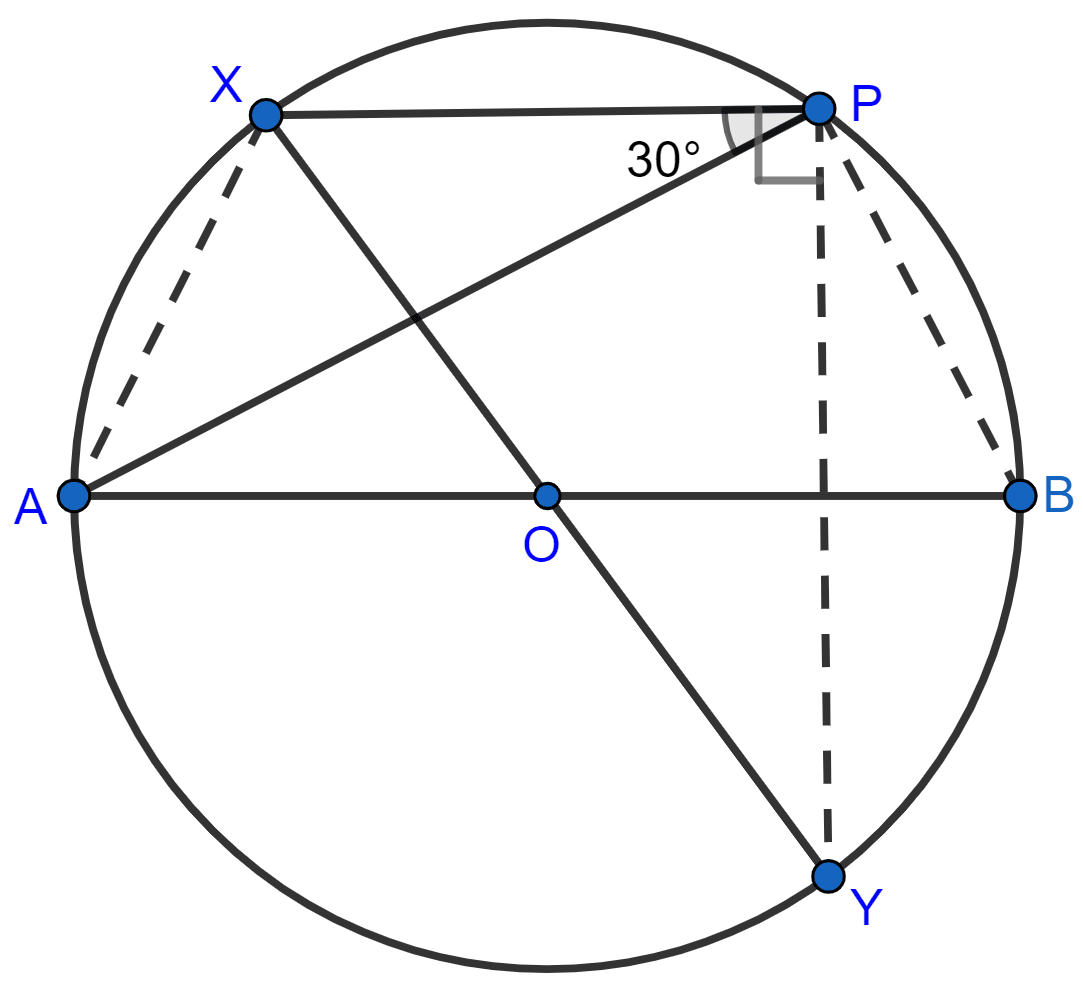
(i) Arc AX subtends ∠AOX at the center and ∠APX at the remaining part of the circle.
⇒ ∠AOX = 2∠APX (∵ angle subtended on center is twice the angle subtended on the remaining part of the circle.)
⇒ ∠AOX = 2 × 30° = 60°.
Hence, the value of ∠AOX = 60°.
(ii) From figure,
∠XPY = 90° [∵ angle in semicircle = 90°]
∴ ∠APY = ∠XPY - ∠APX = 90° - 30° = 60°.
Hence, the value of ∠APY = 60°.
(iii) From figure,
∠APB = 90° [∵ angle in semicircle = 90°]
∴ ∠BPY = ∠APB - ∠APY = 90° - 60° = 30°.
Hence, the value of ∠BPY = 30°.
(iv) In △AOX,
OA = OX (Radius of same circle)
∴ ∠OAX = ∠OXA
Since, sum of angles of a triangle = 180°
∴ ∠AOX + ∠OAX + ∠OXA = 180°
⇒ 60° + ∠OAX + ∠OAX = 180°
⇒ 2∠OAX = 120°
⇒ ∠OAX =
⇒ ∠OAX = 60°.
Hence, the value of ∠OAX = 60°.
Answered By
4 Likes
Related Questions
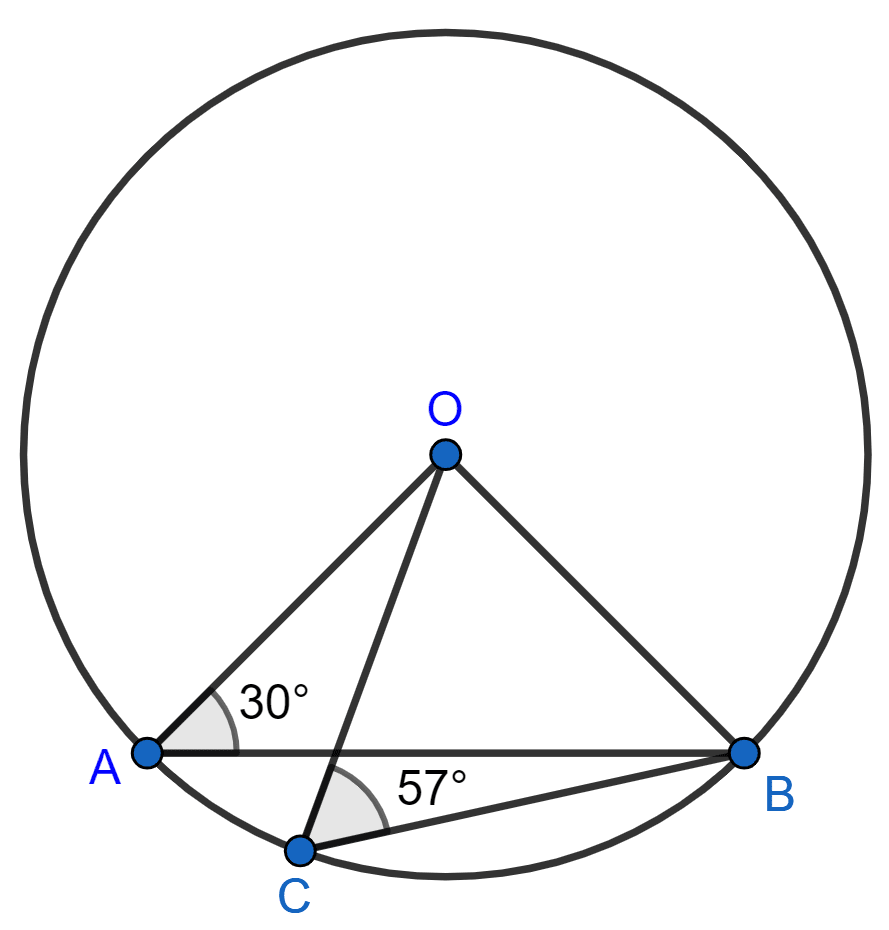
In the given figure, ∠OAB = 30° and ∠OCB = 57°, find ∠BOC and ∠AOC.
In the given figure, O is the center of the circle. If chord AB = chord AC, OP ⊥ AB and OQ ⊥ AC; show that : PB = QC.
In the adjoining figure; AB = AD, BD = CD and ∠DBC = 2∠ABD. Prove that : ABCD is a cyclic quadrilateral.

AB is a diameter of a circle with centre O. Chord CD is equal to radius OC. AC and BD produced intersect at P. Prove that : ∠APB = 60°.
