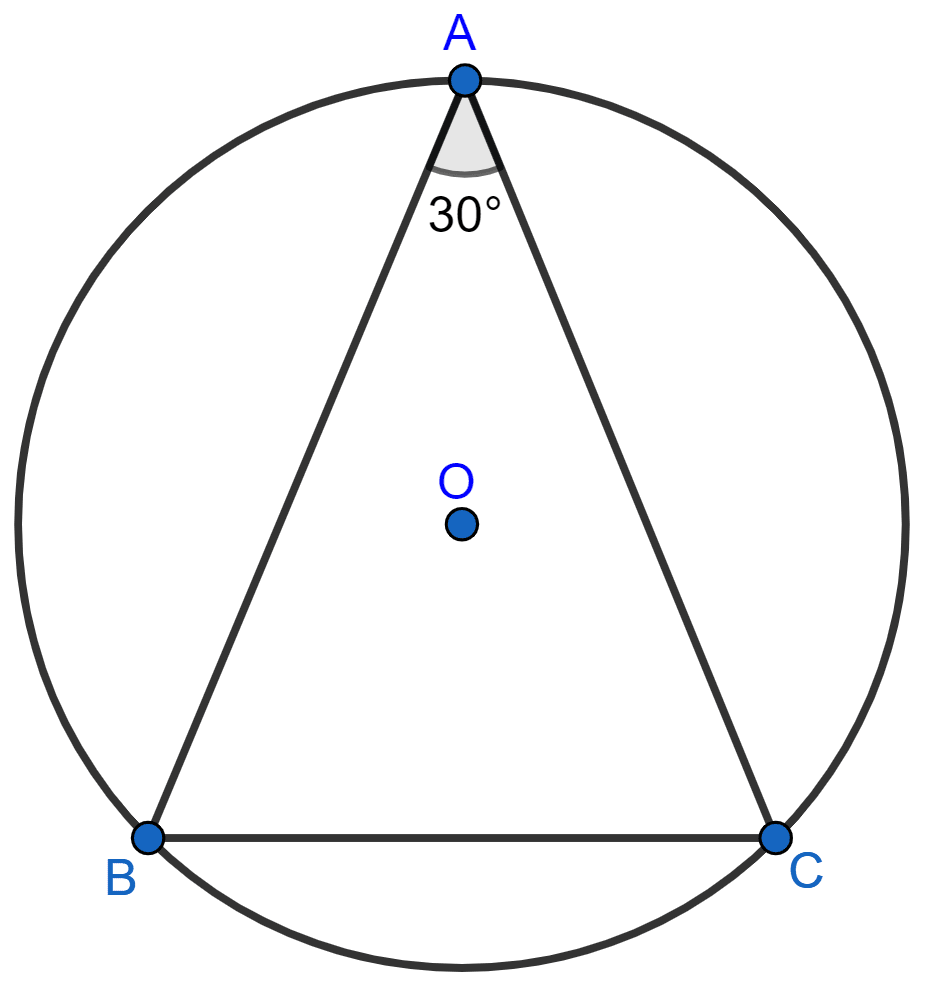
Mathematics
Answer
As angles in same segment are equal.
∴ ∠ABD = ∠ACD = 30°.
From figure,
∠ADB = 90° [As angle in semi-circle is a right angle.]
In △ADB,
⇒ ∠ABD + ∠ADB + ∠BAD = 180° [By angle sum property of triangle]
⇒ 30° + 90° + x = 180°
⇒ 120° + x = 180°
⇒ x = 180° - 120° = 60°.
Hence, the value of x = 60°.
Related Questions
In the given figure, AB is the side of regular pentagon and BC is the side of regular hexagon. Angle BAC is :
132°
66°
90°
120°
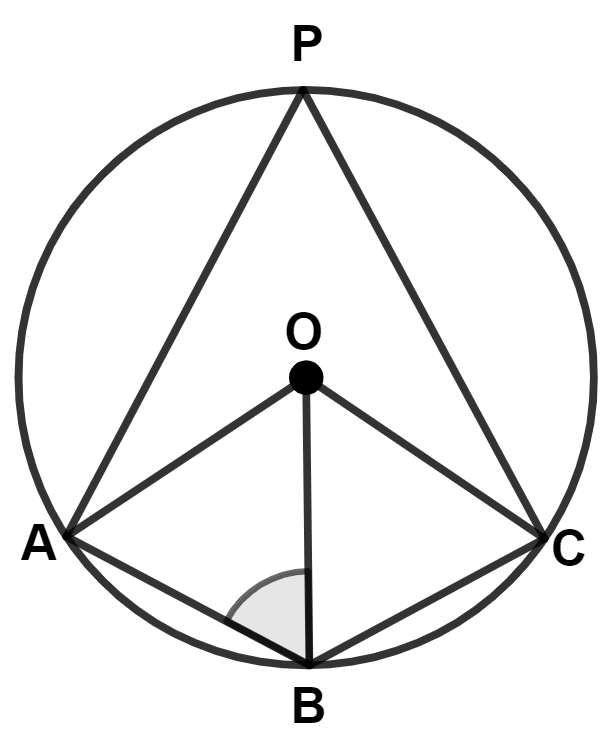
In the given figure, O is center of the circle. Chord BC = chord CD and angle A = 80°. Angle BOC is :
120°
80°
100°
160°

Prove that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base.
In the given figure, ABC is a triangle in which ∠BAC = 30°. Show that BC is equal to the radius of the circumcircle of the triangle ABC, whose centre is O.