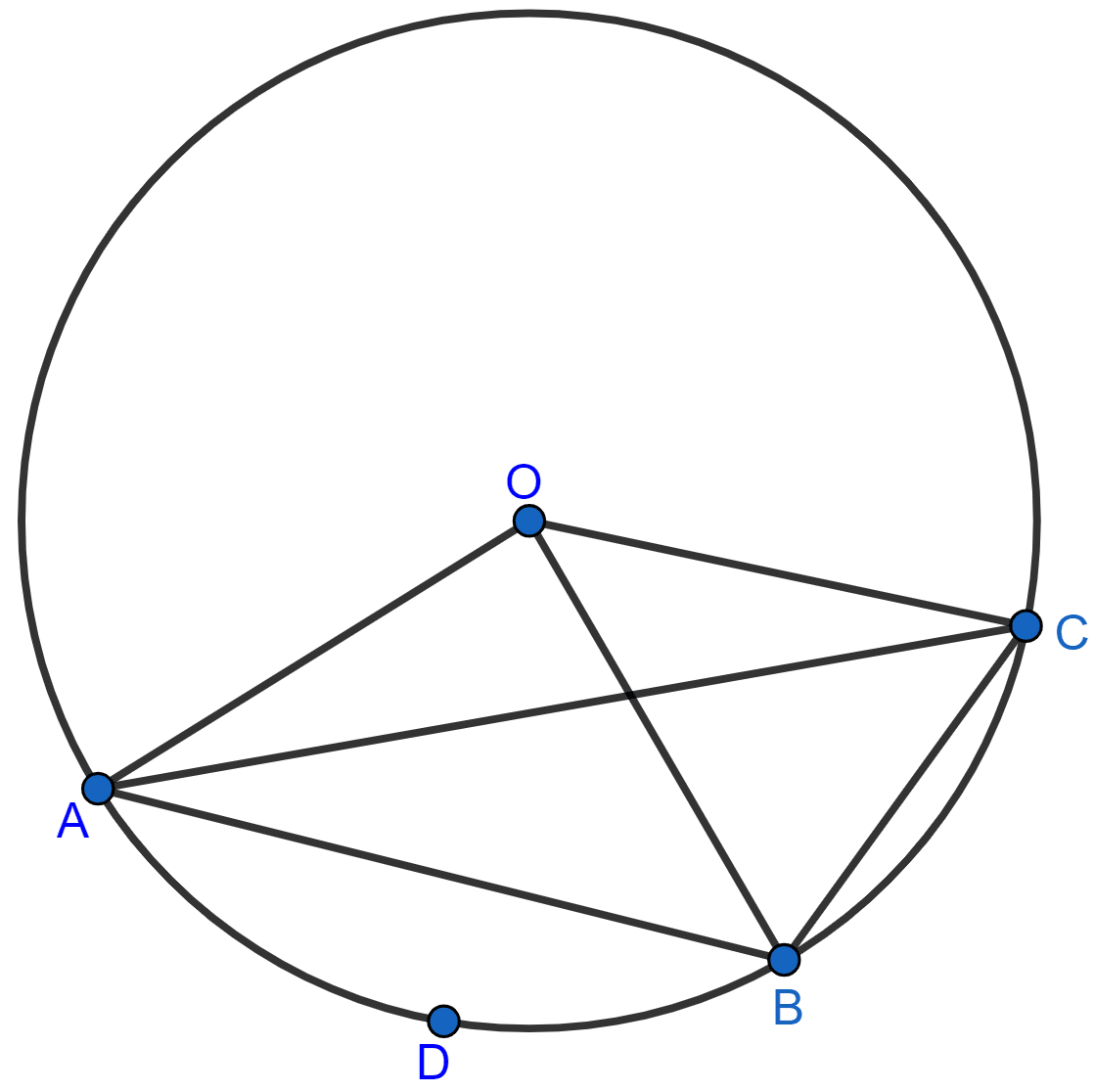
In the figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC. If angle AOB = 108°, find :

(i) ∠CAB,

(ii) ∠ADB.

Join AD and BD.

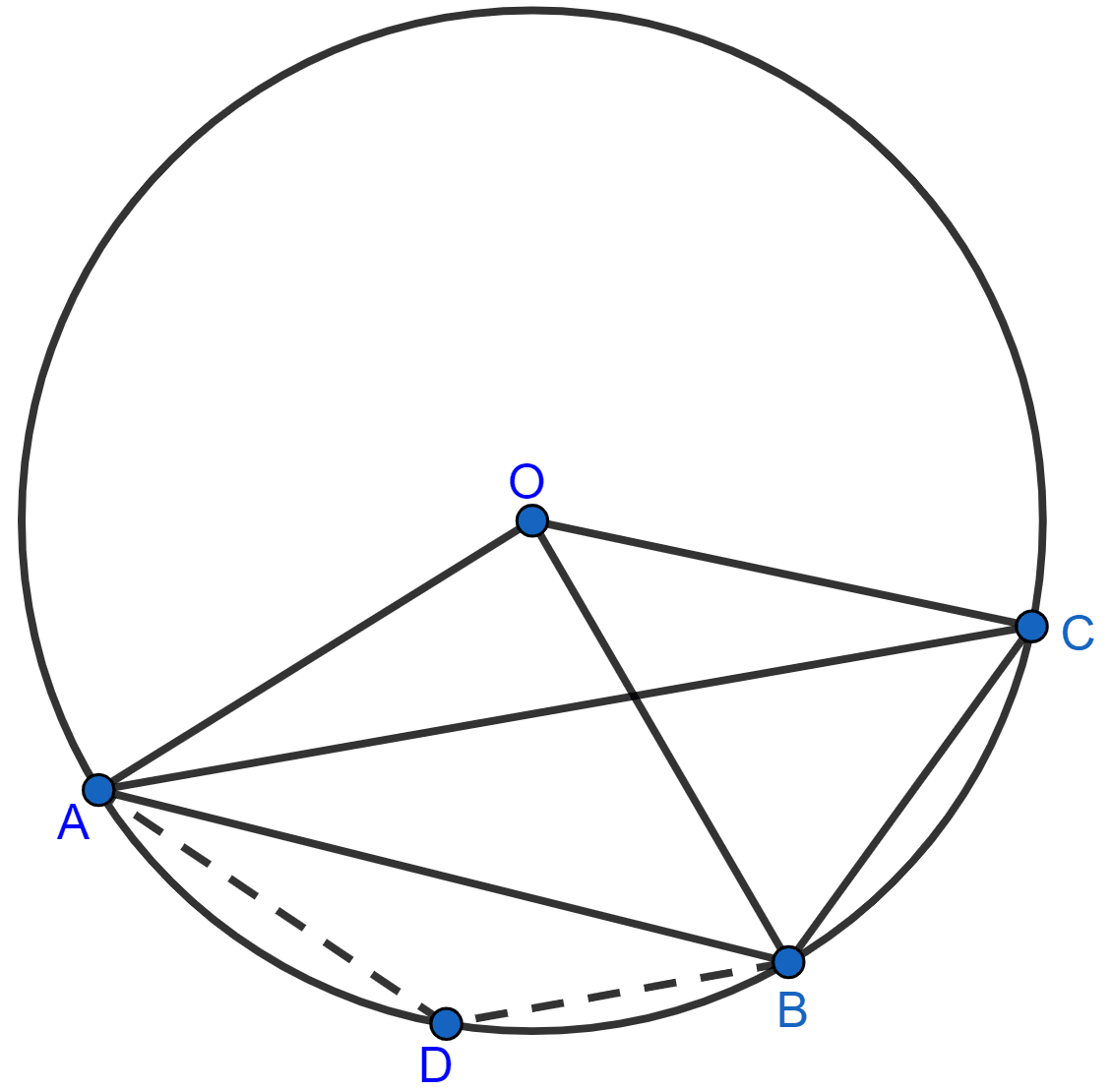
(i) Given,

⇒ arc AB = 2 arc BC

⇒ ∠AOB = 2∠BOC

⇒ ∠BOC = $\dfrac{1}{2}$∠AOB = $\dfrac{1}{2} \times 108°$ = 54°.

We know that,

Angle which an arc subtends at the center is double that which it subtends at any point on the remaining part of the circumference.

∴ ∠BOC = 2∠CAB

⇒ ∠CAB = $\dfrac{1}{2}$∠BOC = $\dfrac{1}{2} \times 54°$ = 27°.

Hence, ∠CAB = 27°.

(ii) Arc AB subtends ∠AOB at the centre and ∠ACB at the remaining part of the circle.

∴ ∠AOB = 2∠ACB

∠ACB = $\dfrac{1}{2}$∠AOB = $\dfrac{1}{2} \times 108°$ = 54°.

In cyclic quadrilateral ADBC,

⇒ ∠ADB + ∠ACB = 180° [As sum of opposite angles in cyclic quadrilateral = 180°]

⇒ ∠ADB + 54° = 180°

⇒ ∠ADB = 180° - 54° = 126°.

Hence, ∠ADB = 126°.