Mathematics
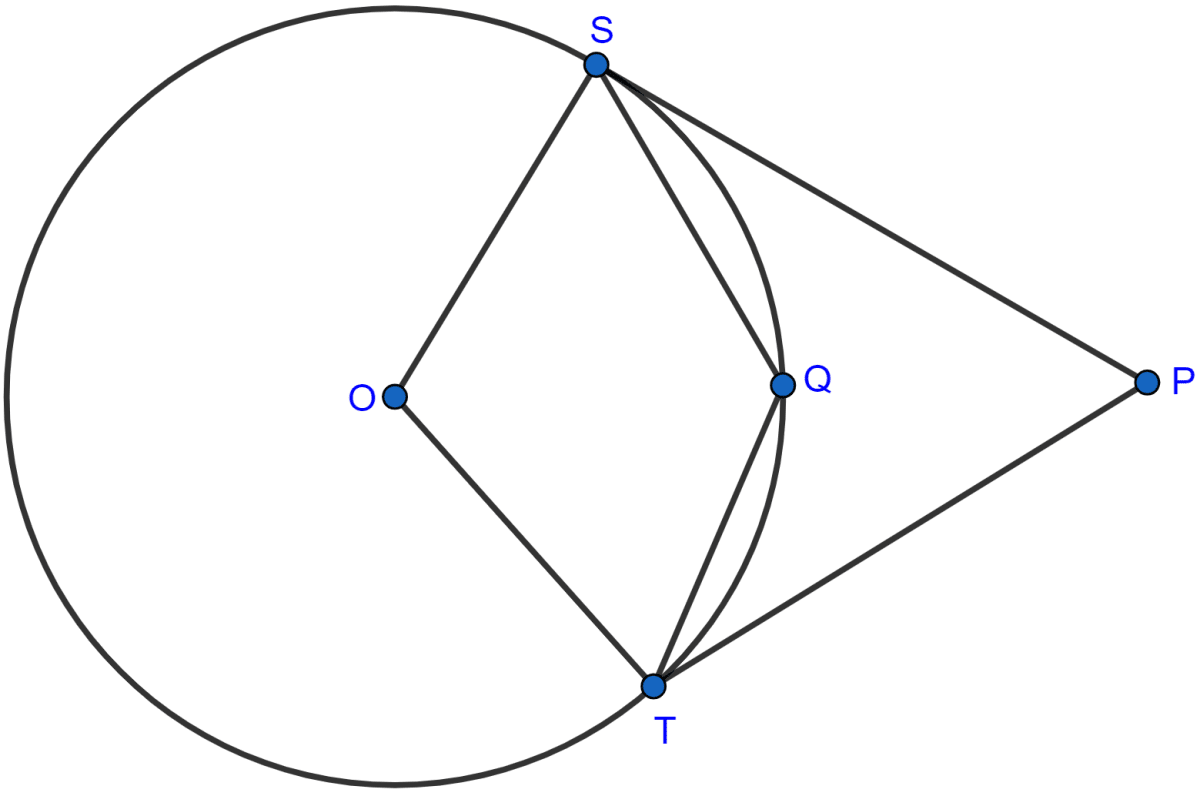
In the figure (ii) given below, O is the centre of the circle. PS and PT are tangents and ∠SPT = 84°. Calculate the sizes of the angles TOS and TQS.
Circles
13 Likes
Answer
Since PS and PT are tangents on the circle. So,
∠OSP = ∠OTP = 90°.
Since, sum of angles in a quadrilateral = 360
∠TOS + ∠OTP + ∠SPT + ∠OSP = 360°
∠TOS + 90° + 84° + 90° = 360°
∠TOS + 264° = 360°
∠TOS = 360° - 264°
∠TOS = 96°.
Reflex ∠TOS = 360° - ∠TOS = 360° - 96° = 264°.
Arc ST subtends reflex ∠TOS at the centre and ∠TQS at the remaining part of the circle.
∴ Reflex ∠TOS = 2∠TQS
∠TQS = x Reflex ∠TOS = x 264° = 132°.
Hence, the value of ∠TOS = 96° and ∠TQS = 132°.
Answered By
9 Likes
Related Questions
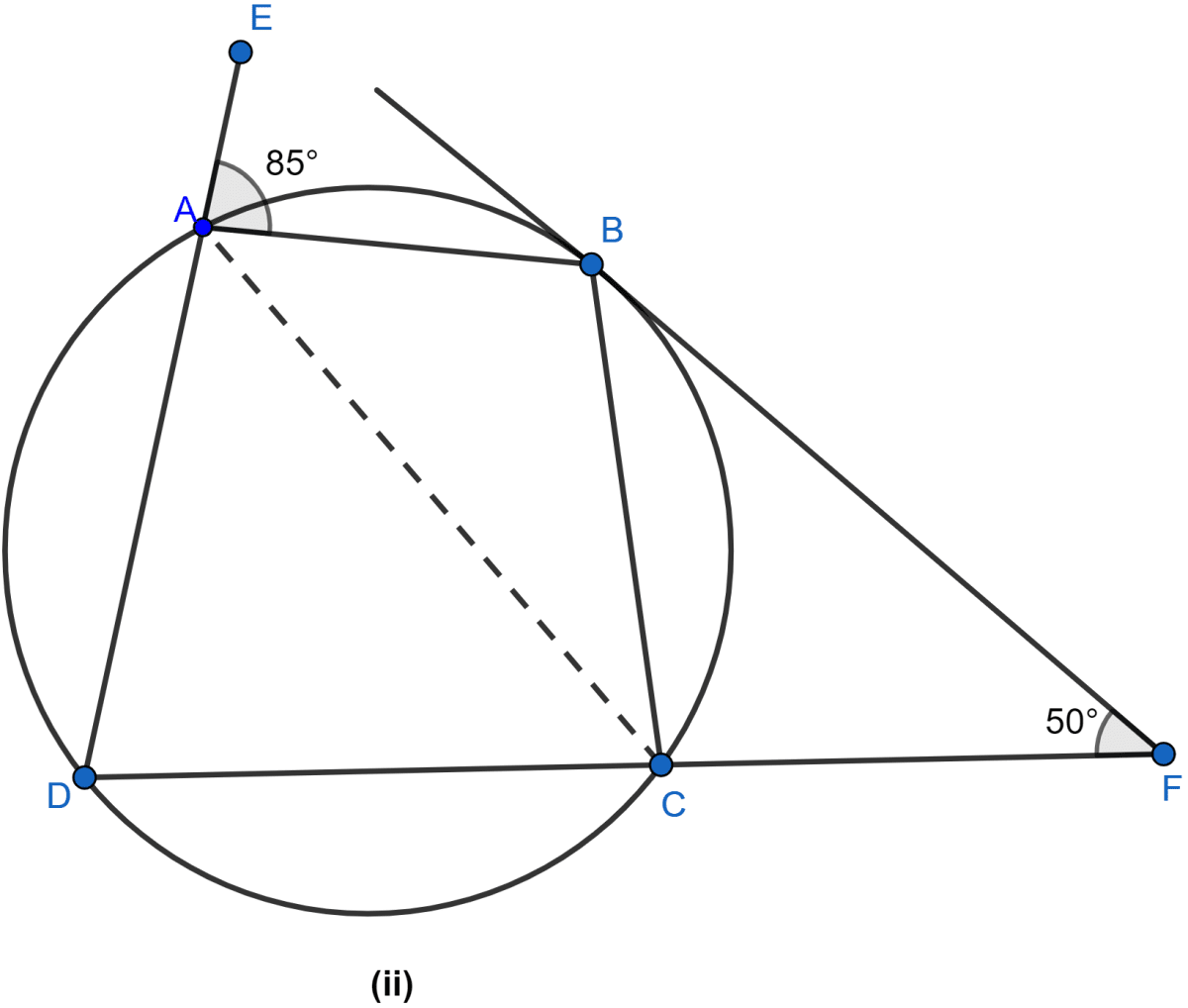
In the figure (ii) given below, ABCD is a cyclic quadrilateral. The tangent to the circle at B meets DC produced at F. If ∠EAB = 85° and ∠BFC = 50°, find ∠CAB.
In the figure (i) given below, O is the centre of the circle and SP is a tangent. If ∠SRT = 65°, find the values of x, y and z.

In the adjoining figure, O is the centre of the circle. Tangents to the circle at A and B meet at C. If ∠ACO = 30°, find
(i) ∠BCO
(ii) ∠AOB
(iii) ∠APB

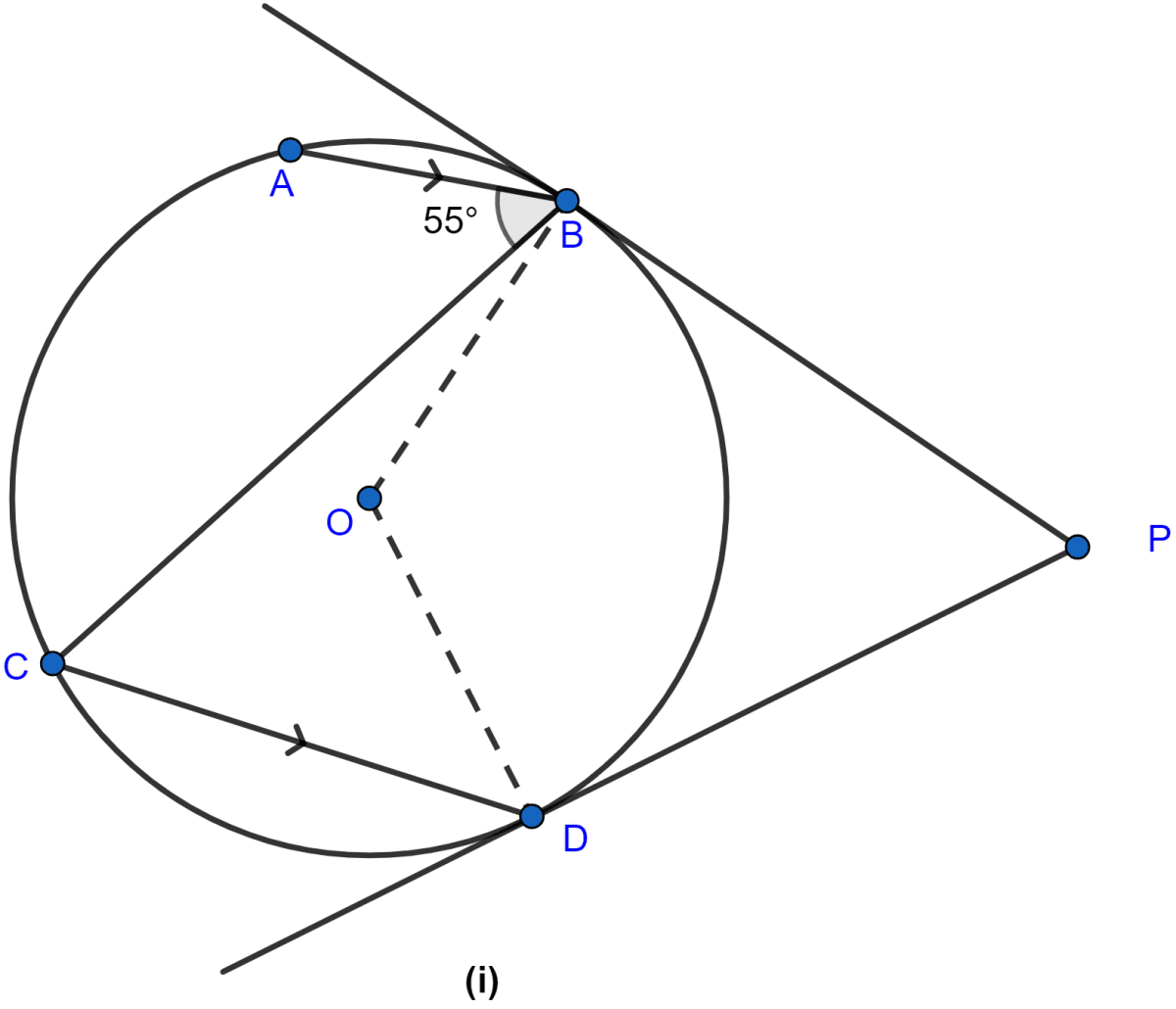
In the figure (i) given below, O is the centre of the circle. The tangents at B and D meet at P. If AB is parallel to CD and ∠ABC = 55°, find
(i) ∠BOD
(ii) ∠BPD.