Mathematics
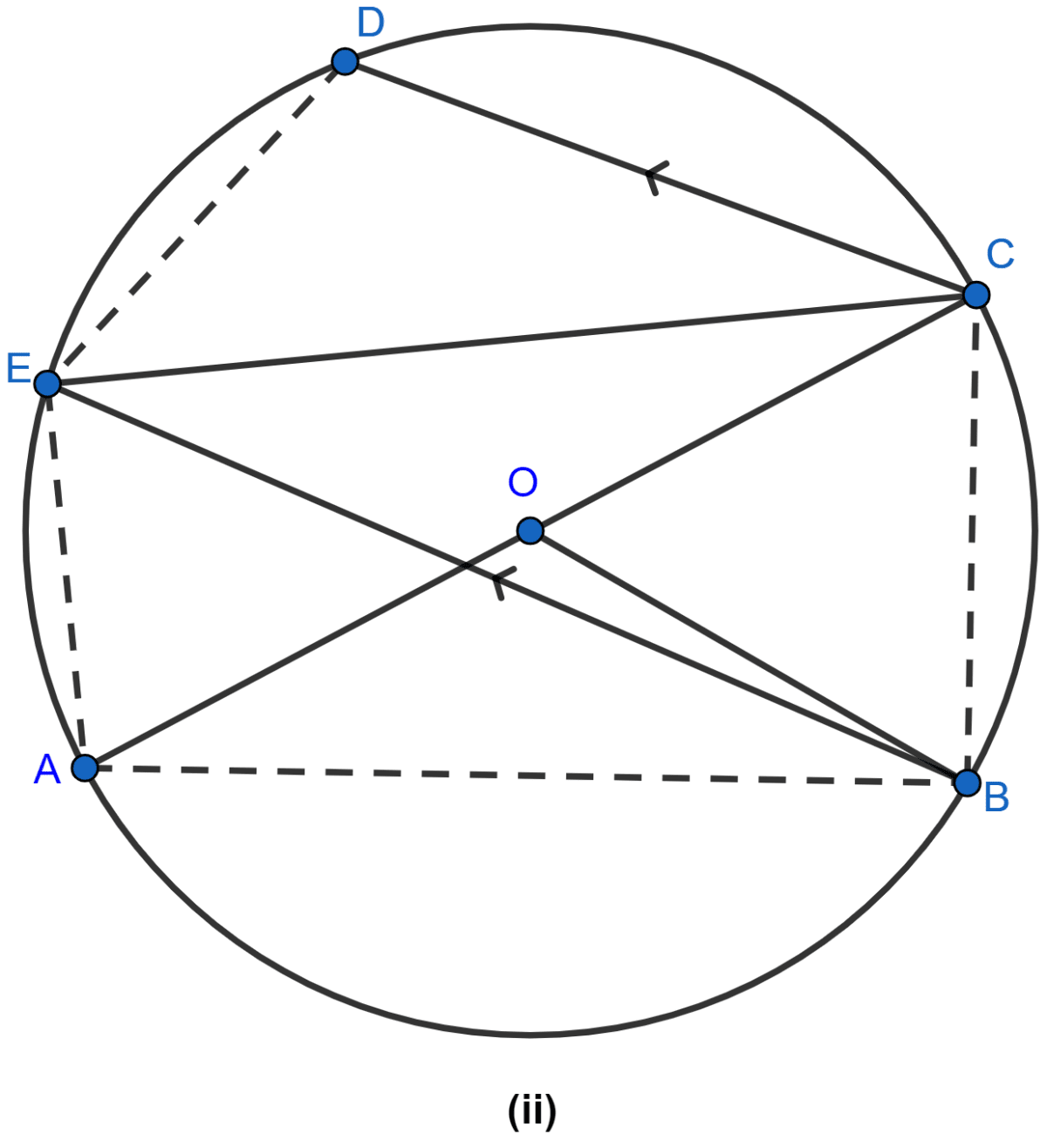
In the figure (ii) given below, AC is a diameter of the circle with centre O. If CD || BE, ∠AOB = 130° and ∠ACE = 20°, find :
(i) ∠BEC
(ii) ∠ACB
(iii) ∠BCD
(iv) ∠CED.
Circles
13 Likes
Answer
∠AOB = 130°.
∠AOB + ∠BOC = 180° (∵ these angles form linear pair.)
130° + ∠BOC = 180°
∠BOC = 180° - 130° = 50°.
(i) Arc BC subtends ∠BOC at the centre and ∠BEC at the remaining part of the circle.
∠BOC = 2∠BEC (∵ angle subtended on centre is twice the angle subtended on the remaining part of the circle).
⇒ 50° = 2∠BEC
⇒ ∠BEC =
Hence, the value of ∠BEC = 25°.
(ii) Arc AB subtends ∠AOB at the centre and ∠ACB at the remaining part of the circle.
⇒ ∠AOB = 2∠ACB (∵ angle subtended on centre is twice the angle subtended on the remaining part of the circle).
⇒ 130° = 2∠ACB
⇒ ∠ACB =
Hence, the value of ∠ACB = 65°.
(iii) Given, CD || EB,
∠ECD = ∠CEB = 25°. (∵ alternate angles are equal.)
From figure,
∠BCD = ∠ACB + ∠ACE + ∠ECD = 65° + 20° + 25° = 110°.
Hence, the value of ∠BCD = 110°.
(iv) EBCD is a cyclic quadrilateral hence the sum of opposite interior angles = 180°.
∴ ∠BED + ∠BCD = 180°
⇒ ∠BEC + ∠CED + ∠BCD = 180°
⇒ 25° + ∠CED + 110° = 180°
⇒ ∠CED + 135° = 180°
⇒ ∠CED = 180° - 135° = 45°.
Hence, the value of ∠CED = 45°.
Answered By
10 Likes
Related Questions
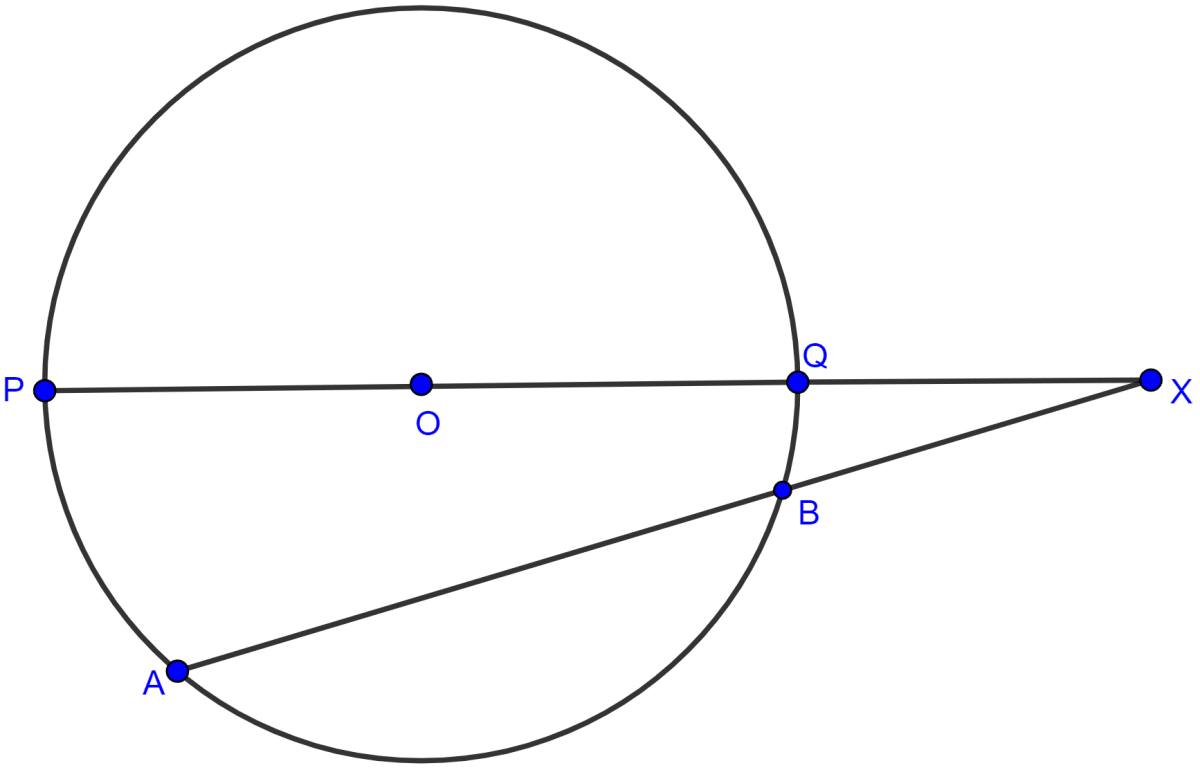
In the adjoining figure, chord AB and diameter PQ of a circle with centre O meet at X. If BX = 5 cm, OX = 10 cm and the radius of the circle is 6 cm, compute the length of AB. Also find the length of tangent drawn from X to the circle.
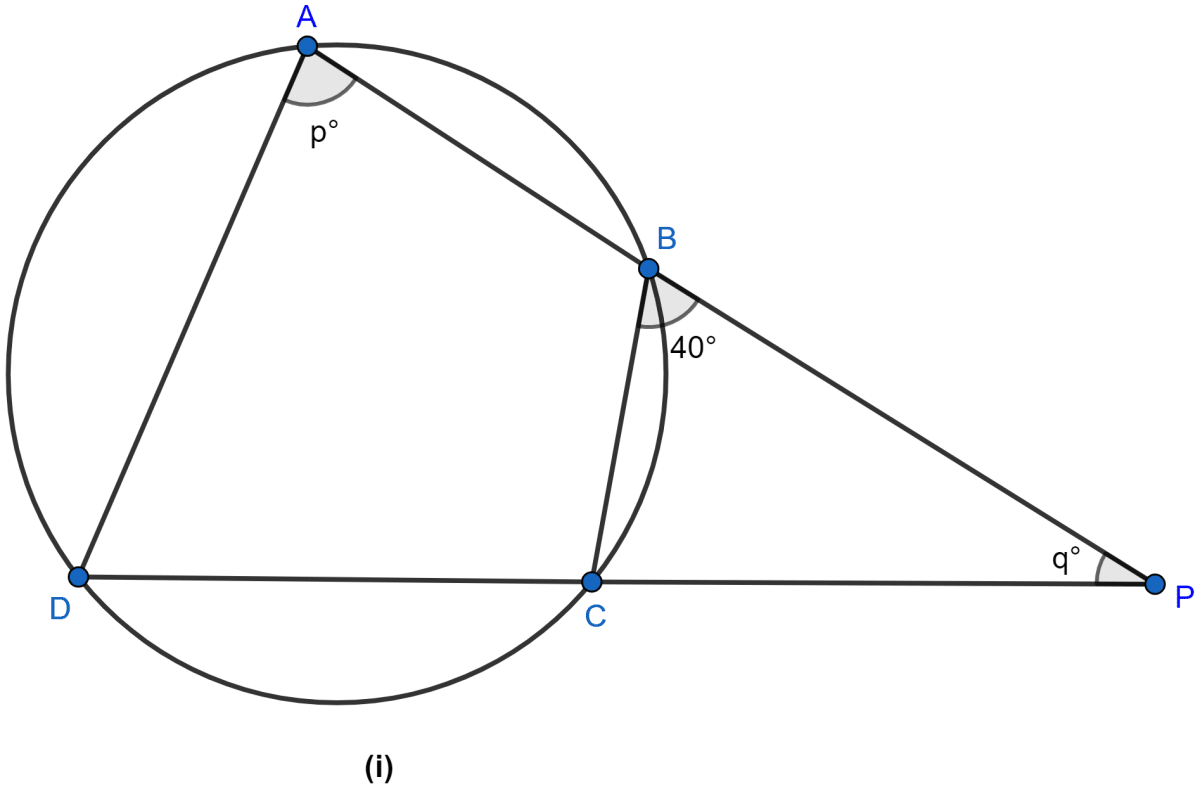
In the figure (i) given below, ∠CBP = 40°, ∠CPB = q° and ∠DAB = p°. Obtain an equation connecting p and q. If AC and BD meet at Q so that ∠AQD = 2q° and the points C, P, B and Q are concyclic, find the values of p and q.
In the figure (i) given below, chords AB, BC and CD of a circle with center O are equal. If ∠BCD = 120°, find
(i) ∠BDC
(ii) ∠BEC
(iii) ∠AEB
(iv) ∠AOB.
Hence, prove that △OAB is equilateral.

In the figure (i) given below, AB and XY are diameters of a circle with centre O. If ∠APX = 30°, find
(i) ∠AOX
(ii) ∠APY
(iii) ∠BPY
(iv) ∠OAX.
