Mathematics
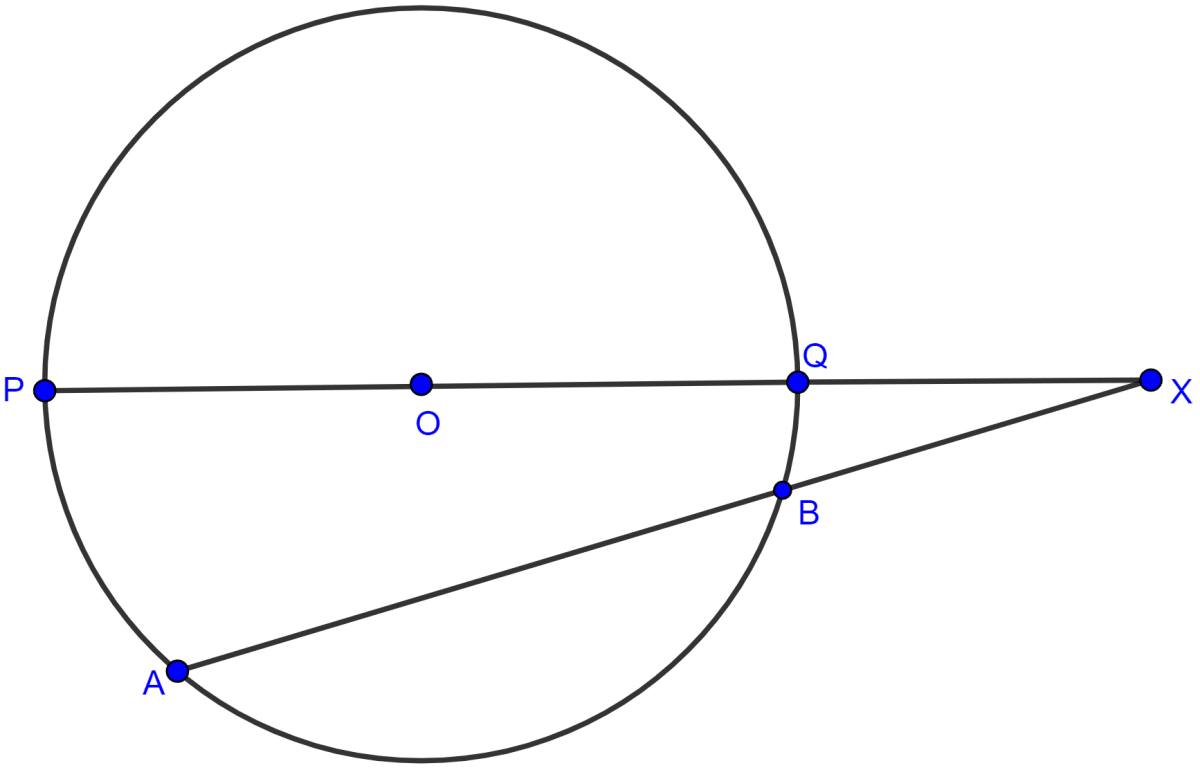
In the adjoining figure, chord AB and diameter PQ of a circle with centre O meet at X. If BX = 5 cm, OX = 10 cm and the radius of the circle is 6 cm, compute the length of AB. Also find the length of tangent drawn from X to the circle.
Circles
3 Likes
Answer
From figure,
OP = OQ = radius of circle = 6 cm.
XP = XO + OP = 10 + 6 = 16 cm.
XQ = XO - OQ = 10 - 6 = 4 cm.
We know that,
If two chords of a circle intersect externally, then the products of the length of segments are equal.
From figure,
XA XB = XP XQ
5 XA = 16 4
XA = = 12.8 cm.
AB = XA - XB = 12.8 - 5 = 7.8 cm
Let XT be the tangent to the circle as shown in the figure below:
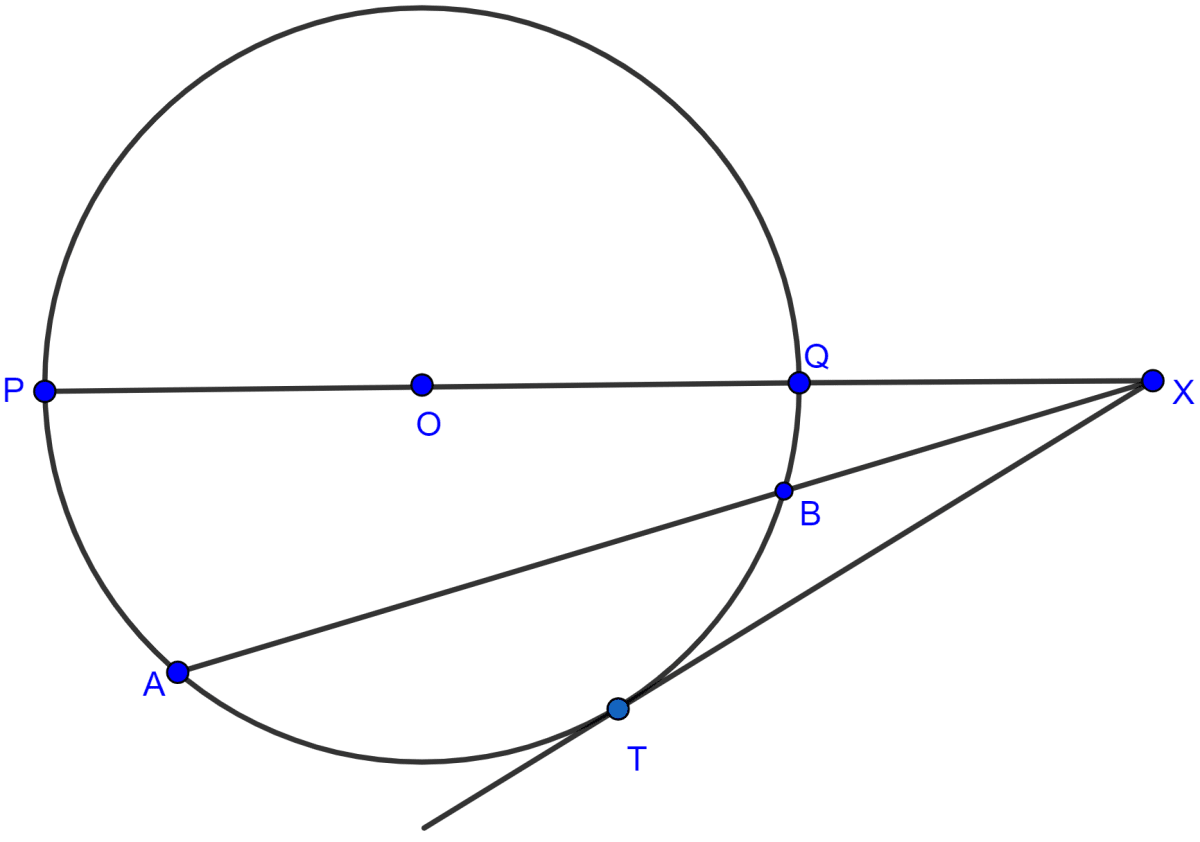
We know that if a chord and a tangent intersect externally, then the product of the lengths of the segments of the chord is equal to the square of the length of the tangent from the point of contact to the point of intersection.
∴ XP.XQ = XT2 …..(i)
XT2 = 16 x 4 = 64
XT = = 8 cm.
Hence, the length of AB = 7.8 cm and length of tangent = 8 cm.
Answered By
1 Like
Related Questions
In the figure (i) given below, chord AB and diameter CD of a circle with centre O meet at P. PT is tangent to the circle at T. If AP = 16 cm, AB = 12 cm and DP = 2 cm, find the length of PT and the radius of the circle.

In the figure (ii) given below, chord AB and diameter CD of a circle meet at P. If AB = 8 cm, BP = 6 cm and PD = 4 cm, find the radius of the circle. Also find the length of the tangent drawn from P to the circle.

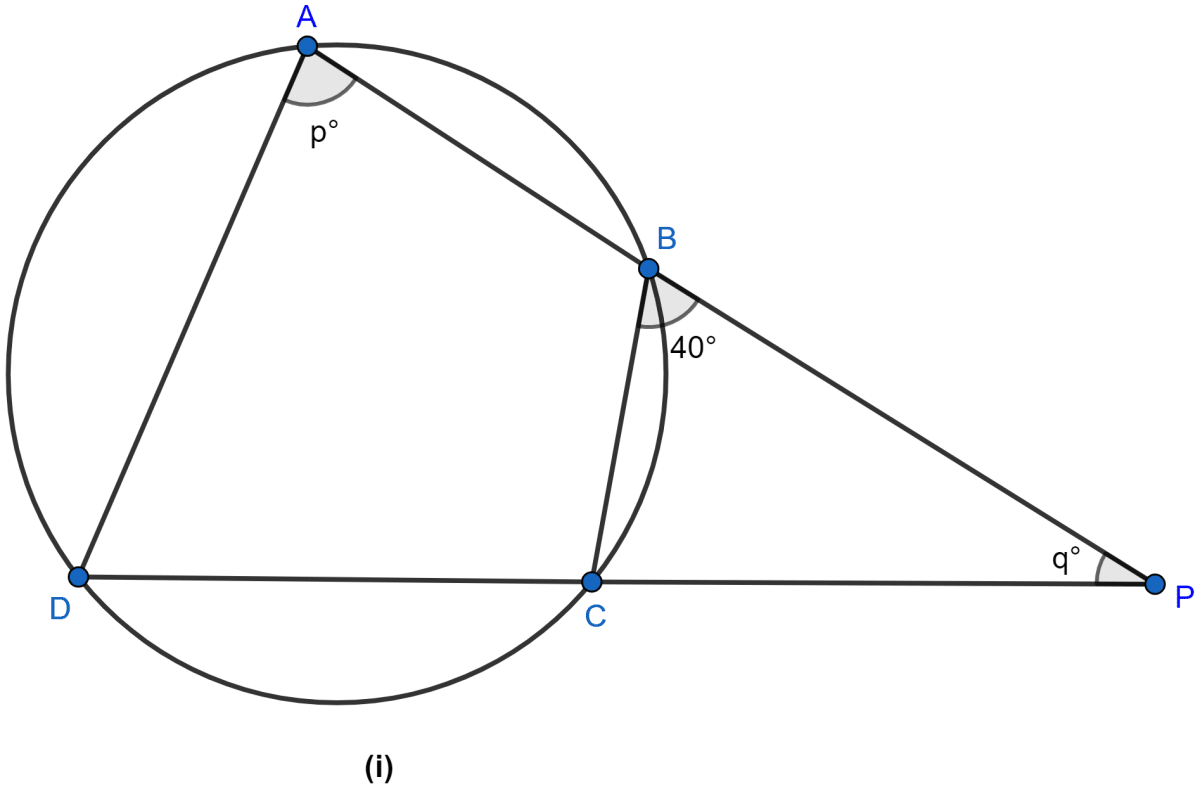
In the figure (i) given below, ∠CBP = 40°, ∠CPB = q° and ∠DAB = p°. Obtain an equation connecting p and q. If AC and BD meet at Q so that ∠AQD = 2q° and the points C, P, B and Q are concyclic, find the values of p and q.
In the figure (ii) given below, AC is a diameter of the circle with centre O. If CD || BE, ∠AOB = 130° and ∠ACE = 20°, find :
(i) ∠BEC
(ii) ∠ACB
(iii) ∠BCD
(iv) ∠CED.