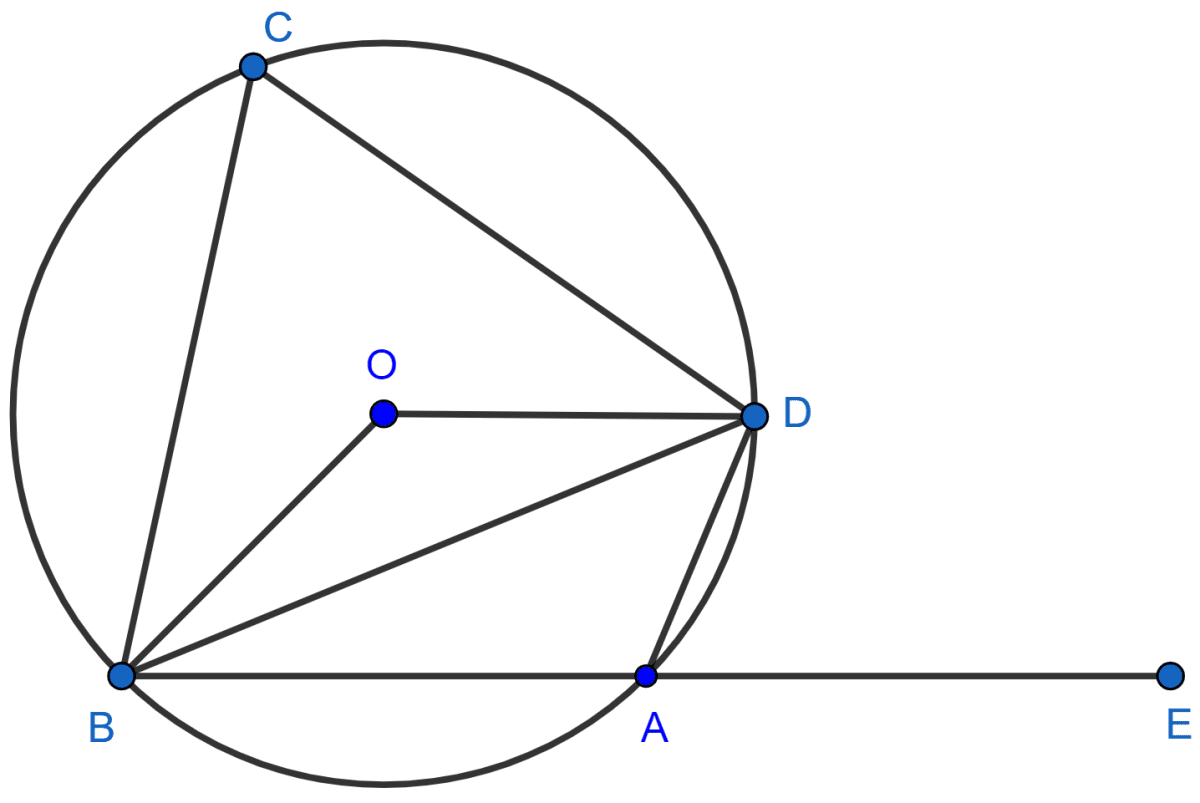
In the figure given, O is the centre of the circle. ∠DAE = 70°. Find giving suitable reasons, the measure of

(i) ∠BCD

(ii) ∠BOD

(iii) ∠OBD

(i) Given,

∠DAE = 70°

⇒ ∠DAE + ∠BAD = 180° [Linear pairs]

⇒ 70° + ∠BAD = 180°

⇒ ∠BAD = 180° - 70° = 110°.

We know that,

Sum of opposite angles in a cyclic quadrilateral = 180°

⇒ ∠BCD + ∠BAD = 180°

⇒ ∠BCD + 110° = 180°

⇒ ∠BCD = 180° - 110° = 70°.

Hence, ∠BCD = 70°.

(ii) We know that,

Angle which an arc subtends at the centre is double that which it subtends at any point on the remaining part of the circumference.

⇒ ∠BOD = 2∠BCD = 2 × 70° = 140°.

Hence, ∠BOD = 140°.

(iii) In △OBD,

OB = OD [Radius of same circle]

∠OBD = ∠ODB = x.

⇒ ∠OBD + ∠ODB + ∠BOD = 180°

⇒ x + x + 140° = 180°

⇒ 2x = 180° - 140°

⇒ 2x = 40°

⇒ x = $\dfrac{40°}{2}$ = 20°.

∴ ∠OBD = 20°.

Hence, ∠OBD = 20°.