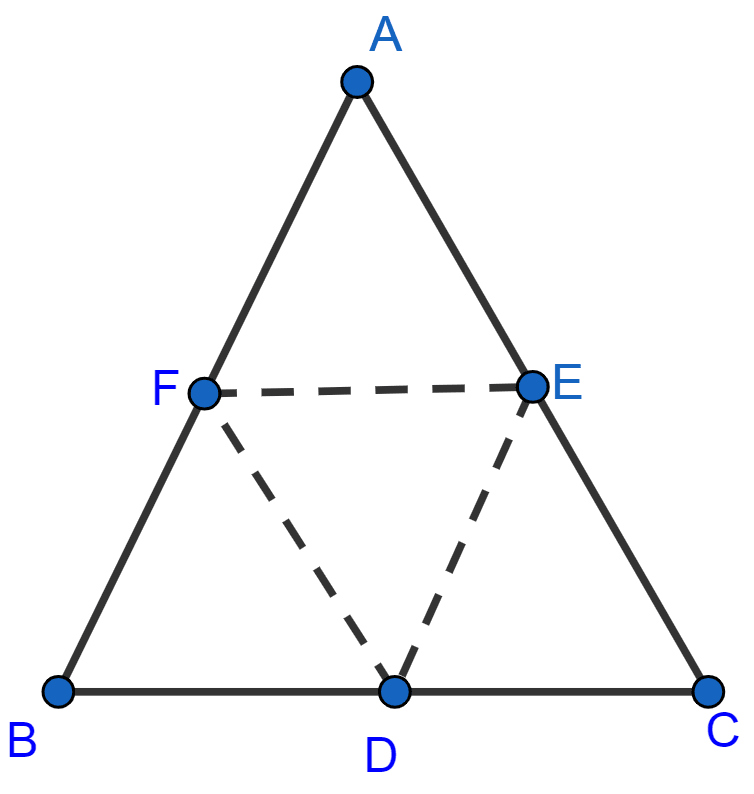
In the figure given below, D, E and F are mid-points of the sides BC, CA and AB respectively of △ ABC. If AB = 6 cm, BC = 4.8 cm and CA = 5.6 cm, find the perimeter of

(i) the trapezium of FBCE

(ii) the triangle DEF.

(i) Since F is midpoint of AB and E is midpoint of AC,

∴ FE is parallel to BC and FE = $\dfrac{1}{2}$BC = $\dfrac{1}{2}(4.8)$ = 2.4 cm (By midpoint theorem)

FB = $\dfrac{1}{2}$AB = $\dfrac{1}{2}(6)$ = 3 cm

EC = $\dfrac{1}{2}$AC = $\dfrac{1}{2}(5.6)$ = 2.8 cm.

Perimeter of trapezium FBCE = FE + EC + BC + FB = 2.4 + 2.8 + 4.8 + 3 = 13 cm.

Hence, perimeter of trapezium FBCE = 13 cm.

(ii) Since F is midpoint of AB and E is midpoint of AC,

∴ FE is parallel to BC and FE = $\dfrac{1}{2}$BC = $\dfrac{1}{2}(4.8)$ = 2.4 cm (By midpoint theorem)

Since F is midpoint of AB and D is midpoint of BC,

∴ FD is parallel to AC and FD = $\dfrac{1}{2}$AC = $\dfrac{1}{2}(5.6)$ = 2.8 cm (By midpoint theorem)

Since E is midpoint of AC and D is midpoint of BC,

∴ ED is parallel to AB and ED = $\dfrac{1}{2}$AB = $\dfrac{1}{2}(6)$ = 3 cm (By midpoint theorem)

Perimeter of △DEF = FE + FD + ED = 2.4 + 2.8 + 3 = 8.2 cm

Hence, perimeter of △DEF = 8.2 cm.