Mathematics
Prove that four triangles formed by joining in pairs, the mid-points of the sides of a triangle are congruent to each other.
Mid-point Theorem
33 Likes
Answer
From figure,
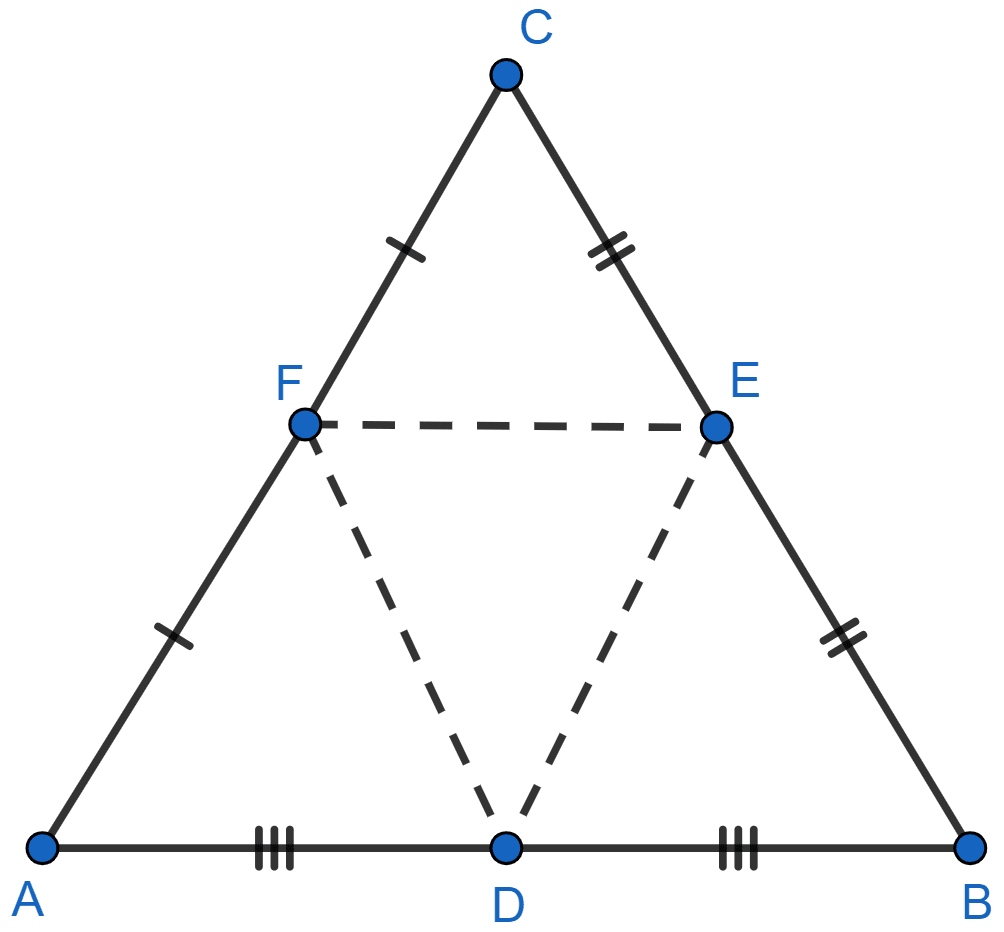
In △ABC,
D, E and F are mid-points of AB, BC and CA respectively.
Now join DE, EF and FD.
To prove :
△ADF ≅ △DBE ≅ △ECF ≅ △DEF
In △ABC,
D and E are midpoints of AB and BC
∴ DE || AC or,
DE || FC …….(i)
or DE || AF ………(ii)
D and F are midpoints of AB and AC
∴ DF || BC or,
DF || EC …….(iii)
or DF || BE ……..(iv)
F and E are midpoints of AC and BC
∴ FE || AB or,
FE || AD …….(v)
or FE || DB (vi)
From (i) and (iii) we get,
DE || FC and DF || EC.
∴ DECF is a parallelogram.
We know that,
Diagonal FE divides the parallelogram DECF in two congruent triangles DEF and CEF.
∴ △DEF ≅ △ECF …….(1)
From (ii) and (v) we get,
DE || AF and FE || AD.
∴ ADEF is a parallelogram.
We know that,
Diagonal FD divides the parallelogram in two congruent triangles DEF and AFD.
∴ △DEF ≅ △AFD …….(2)
From (iv) and (vi) we get,
DF || BE and FE || DB.
∴ DBEF is a parallelogram.
We know that,
Diagonal DE divides the parallelogram in two congruent triangles DEF and DBE.
∴ △DEF ≅ △DBE …….(3)
Using equations 1, 2 and 3 we get,
△ADF ≅ △DBE ≅ △ECF ≅ △DEF.
Hence, proved that four triangles formed by joining in pairs, the mid-points of the sides of a triangle are congruent to each other.
Answered By
21 Likes
Related Questions
In the figure given below, D and E are mid-points of the sides AB and AC respectively. If BC = 5.6 cm and ∠B = 72°, compute
(i) DE
(ii) ∠ADE

In the figure given below, D and E are mid-points of AB, BC respectively and DF || BC. Prove that DBEF is a parallelogram. Calculate AC if AF = 2.6 cm.
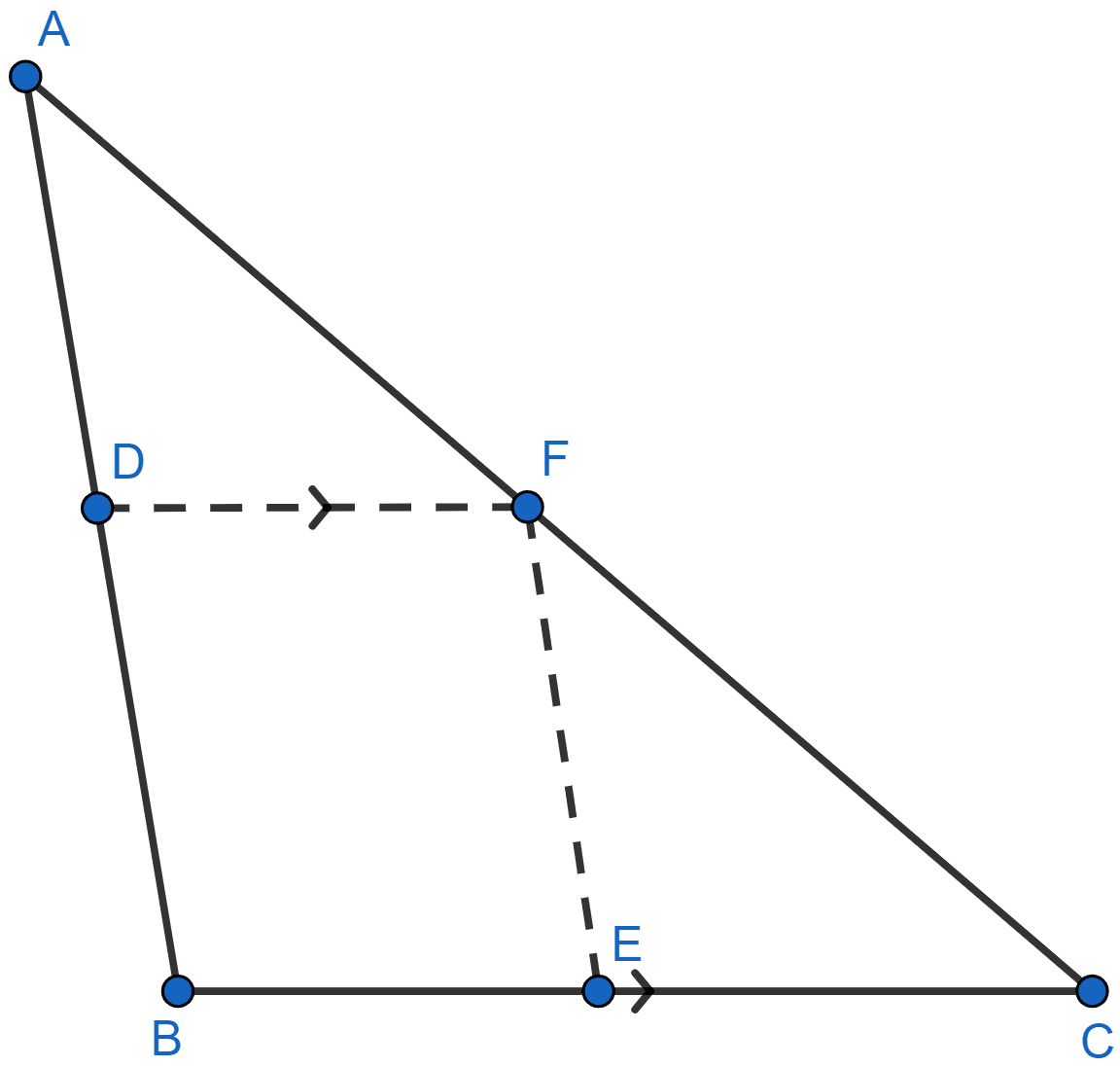
If D, E and F are mid-points of the sides AB, BC and CA respectively of an isosceles triangle, ABC, prove that △DEF is also isosceles.
The diagonals AC and BD of a parallelogram ABCD intersect at O. If P is the mid-point of AD, prove that
(i) PO || AB
(ii) PO = CD.