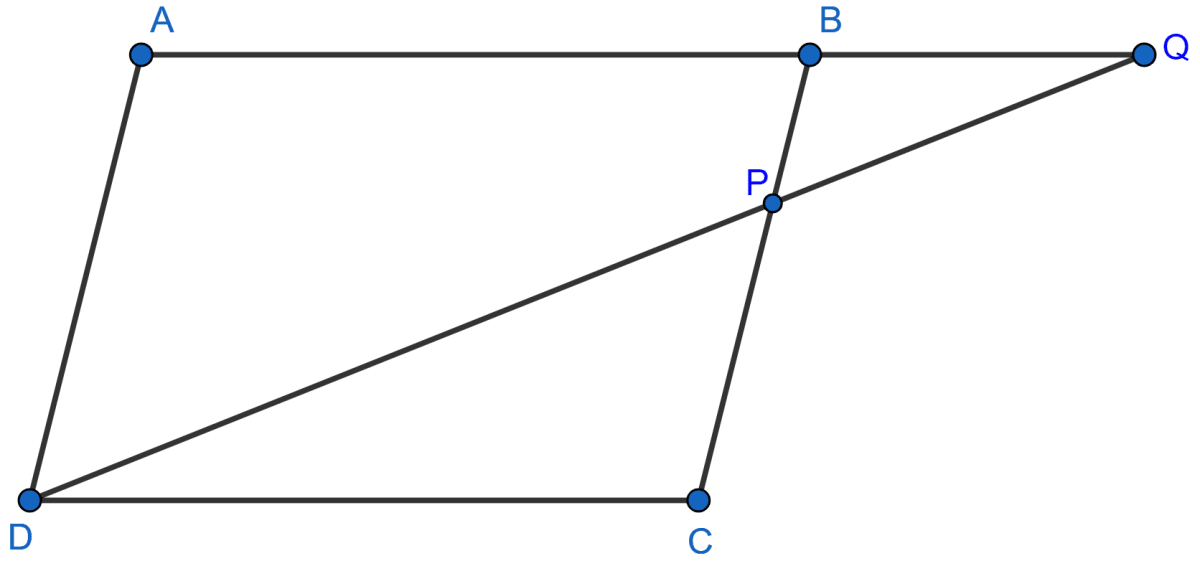
In the figure, given below, ABCD is a parallelogram. P is a point on BC such that BP : PC = 1 : 2. DP produced meets AB produced at Q. Given the area of triangle CPQ = 20 cm².

Calculate :

(i) area of triangle CDP,

(ii) area of parallelogram ABCD.

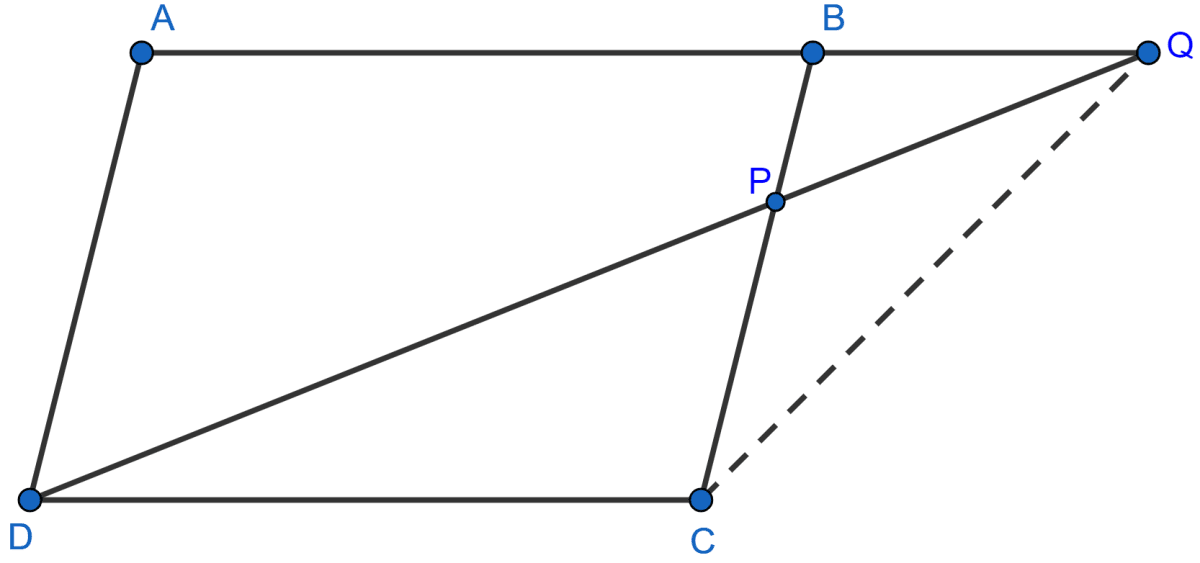
(i) In △BPQ and △CPD,

⇒ ∠BPQ = ∠CPD [Vertically opposite angles are equal]

⇒ ∠BQP = ∠PDC [Alternate angles are equal]

∴ △BPQ ~ △CPD [By AA]

Since, corresponding sides of similar triangle are proportional to each other.

$\therefore \dfrac{BP}{PC} = \dfrac{PQ}{PD} = \dfrac{BQ}{CD} = \dfrac{1}{2}$.

As ΔBPQ and ΔCPQ have common vertex at Q and their bases BP and CP are along the same straight line.

So,

$\Rightarrow \dfrac{\text{Area of ΔBPQ}}{\text{Area of ΔCPQ}} = \dfrac{BP}{PC} \\[1em] \Rightarrow \dfrac{\text{Area of ΔBPQ}}{20} = \dfrac{1}{2} \\[1em] \Rightarrow \text{Area of ΔBPQ} = \dfrac{20}{2} \\[1em] \Rightarrow \text{Area of ΔBPQ} = 10 \text{ cm}^2$

We know that,

The ratio of the areas of two similar triangles is equal to the ratio of squares of their corresponding sides.

$\phantom{\Rightarrow} \dfrac{\text{Area of ΔBPQ}}{\text{Area of ΔCPD}} = \dfrac{BP^2}{PC^2} \\[1em] \Rightarrow \dfrac{10}{\text{Area of ΔCPD}} = \dfrac{1^2}{2^2} \\[1em] \Rightarrow \text{ Area of ΔCPD} = \dfrac{10 \times 4}{1} \\[1em] \Rightarrow \text{ Area of ΔCPD} = 40 \text{ cm}^2.$

Hence, area of ΔCPD = 40 cm².

(ii) From part (i),

$\dfrac{PQ}{PD} = \dfrac{1}{2}$

Let PQ = x and PD = 2x.

From figure,

QD = PQ + PD = x + 2x = 3x.

$\dfrac{QD}{QP} = \dfrac{3x}{x} = 3$ ……..(1)

In △BPQ and △AQD,

⇒ ∠QBP = ∠QAD [Corresponding angles are equal]

⇒ ∠BQP = ∠AQD [Common]

∴ △BPQ ~ △AQD [By AA]

Since, corresponding sides of similar triangle are proportional to each other.

$\therefore \dfrac{AQ}{BQ} = \dfrac{QD}{QP} = \dfrac{AD}{BP} = 3.$

We know that,

The ratio of the areas of two similar triangles is equal to the ratio of squares of their corresponding sides.

$\therefore \dfrac{\text{Area of △AQD}}{\text{Area of △BPQ}} = \Big(\dfrac{AQ}{BQ}\Big)^2 \\[1em] \Rightarrow \dfrac{\text{Area of △AQD}}{10} = 3^2 \\[1em] \Rightarrow \dfrac{\text{Area of △AQD}}{10} = 9 \\[1em] \Rightarrow \text{Area of △AQD} = 90 \text{ cm}^2.$

Area of trapezium ADPB = Area of △AQD - Area of △BPQ = 90 - 10 = 80 cm².

Area of || gm ABCD = Area of △CDP + Area of trapezium ADPB = 40 + 80 = 120 cm².

Hence, area of || gm ABCD = 120 cm².