Mathematics
In the figure, given, ABC is a triangle and BC is parallel to the y-axis. AB and AC intersect the y-axis at P and Q respectively.
(i) Write the co-ordinates of A.
(ii) Find the length of AB and AC.
(iii) Find the ratio in which Q divides AC.
(iv) Find the equation of the line AC.
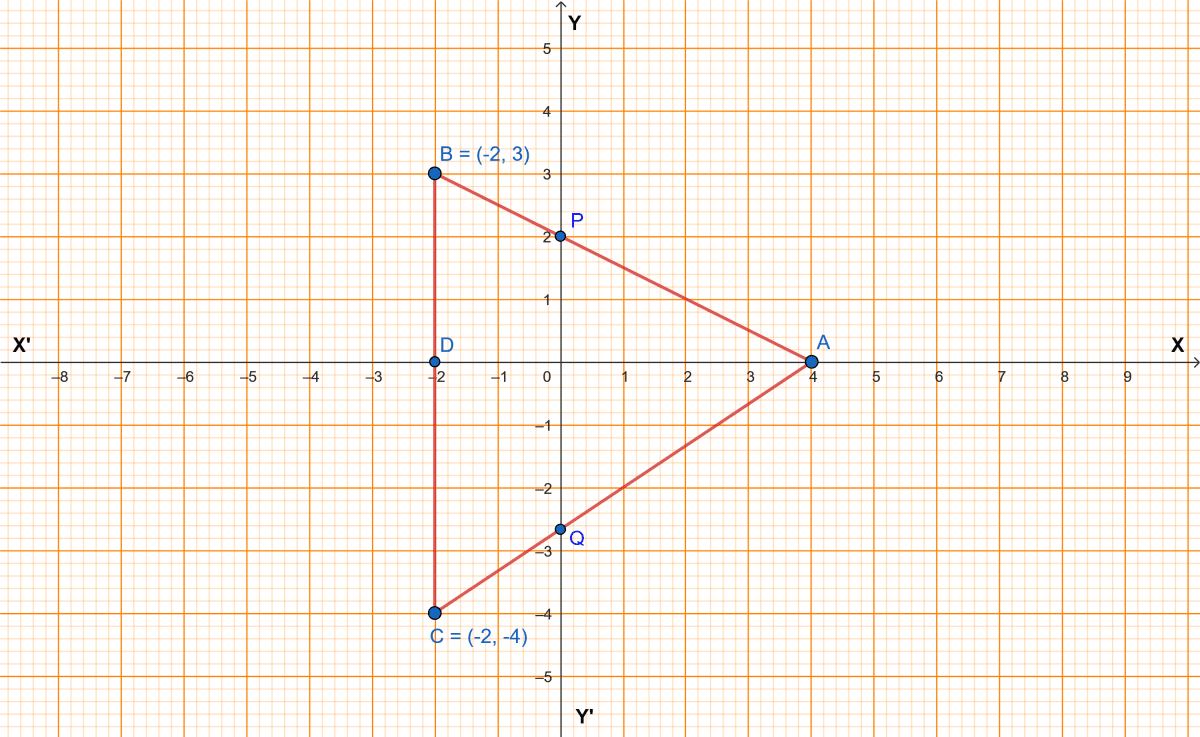
Straight Line Eq
9 Likes
Answer
(i) From graph,
The co-ordinates of A are (4, 0).
(ii) By distance formula,
Distance =
Substituting values we get,
Hence, length of AB = units and AC = units.
(iii) From figure,
Q lies on y-axis.
∴ x co-ordinate of Q = 0.
Let co-ordinate of Q are (0, a).
Let ratio in which Q divides AC be k : 1.
By section-formula,
k : 1 = 2 : 1.
Hence, Q divides AC in the ratio 2 : 1.
(iv) Slope of AC = .
By point-slope form,
Equation of AB is :
⇒ y - y1 = m(x - x1)
⇒ y - 0 = (x - 4)
⇒ 3y = 2x - 8
⇒ 2x - 3y = 8.
Hence, equation of AC is 2x - 3y = 8.
Answered By
6 Likes
Related Questions
A straight line passes through the points P(-1, 4) and Q(5, -2). It intersects x-axis at point A and y-axis at point B. M is the mid-point of the line segment AB. Find :
(i) the equation of the line.
(ii) the co-ordinates of points A and B.
(iii) the co-ordinates of point M.
In the given figure, line AB meets y-axis at point A. Line through C(2, 10) and D intersects line AB at right angle at point P. Find :
(i) equation of line AB.
(ii) equation of line CD.
(iii) co-ordinates of point E and D.

Find the equation of the line through the points A(-1, 3) and B(0, 2). Hence, show that the points A, B and C(1, 1) are collinear.
A line AB meets X-axis at A and Y-axis at B. P(4, -1) divides AB in the ratio 1 : 2.
(i) Find the co-ordinates of A and B.
(ii) Find the equation of the line through P and perpendicular to AB.
