Mathematics
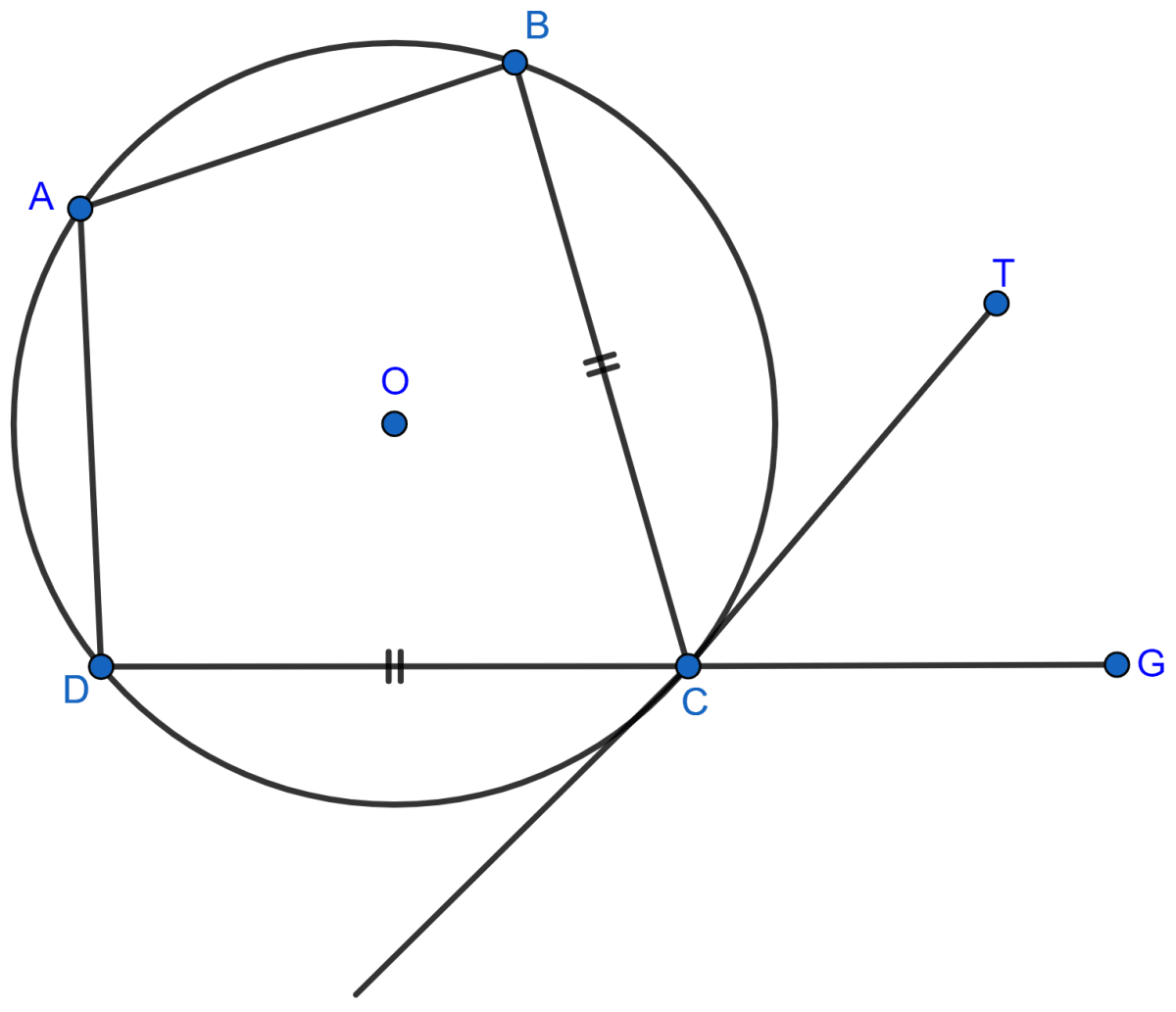
In the figure, ABCD is a cyclic quadrilateral with BC = CD. TC is tangent to the circle at point C and DC is produced to point G. If ∠BCG = 108° and O is the center of the circle, find :
(i) angle BCT
(ii) angle DOC
Circles
14 Likes
Answer
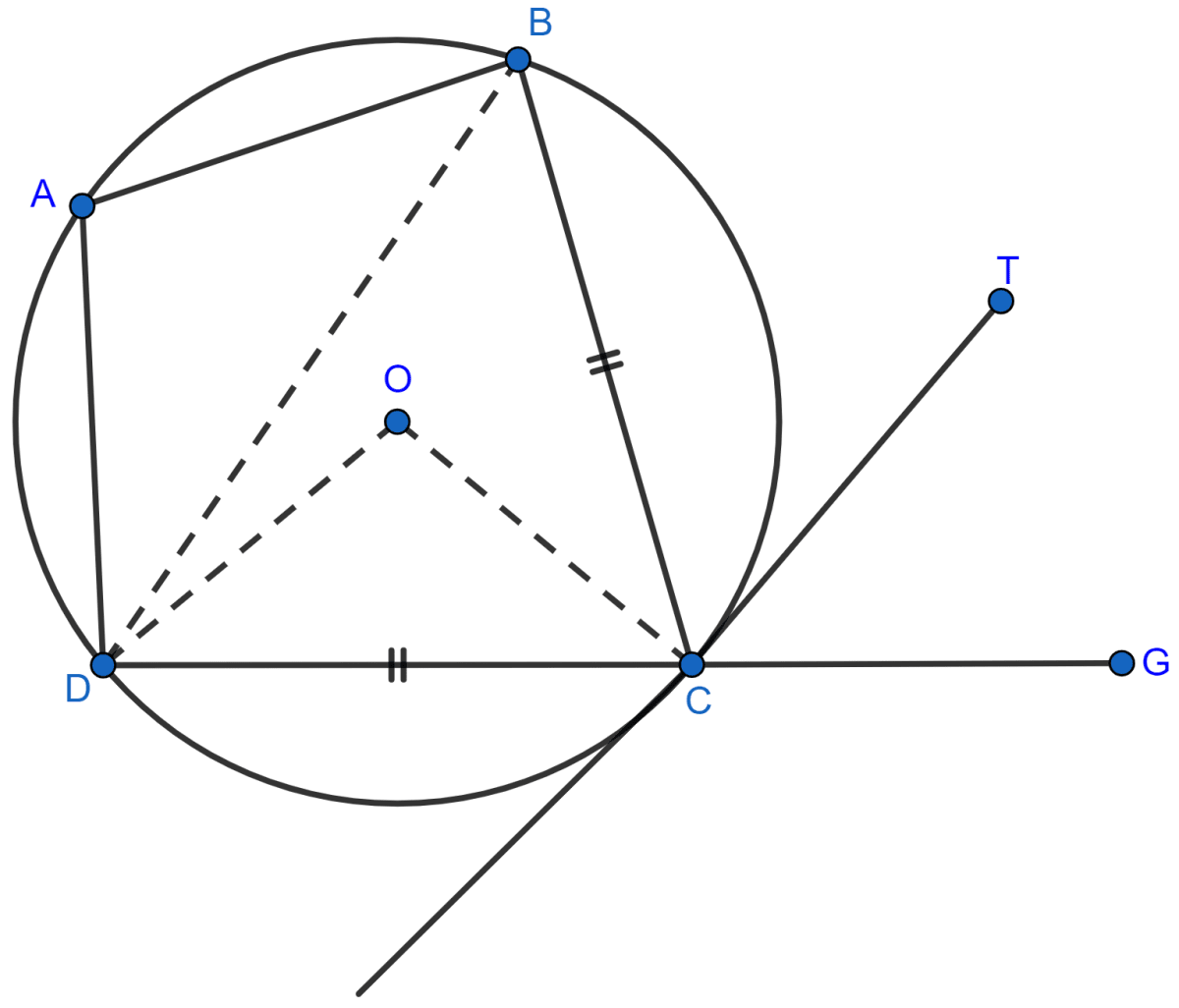
Join OC, OD and BD.
Given,
∠BCG = 108°
From figure,
⇒ ∠BCG + ∠BCD = 180° [Linear pairs]
⇒ 108° + ∠BCD = 180°
⇒ ∠BCD = 180° - 108°
⇒ ∠BCD = 72°.
From figure,
⇒ ∠BDC = ∠DBC = x(let) [As, angles opposite to equal sides are equal]
In triangle BDC,
⇒ ∠DBC + ∠BDC + ∠BCD = 180° [Angle sum property of triangle]
⇒ x + x + 72° = 180°
⇒ 2x + 72° = 180°
⇒ 2x = 180° - 72°
⇒ 2x = 108°
⇒ x =
⇒ x = 54°.
From figure.
∠BCT = ∠BDC (Angles in alternate segment are equal)
∠BCT = 54°.
Hence, ∠BCT = 54°.
(ii) As, angle subtended by a segment on center is twice the angle subtended by it on any other part of circumference.
⇒ ∠DOC = 2∠DBC
⇒ ∠DOC = 2(54°) = 108°.
Hence, ∠DOC = 108°.
Answered By
10 Likes
Related Questions
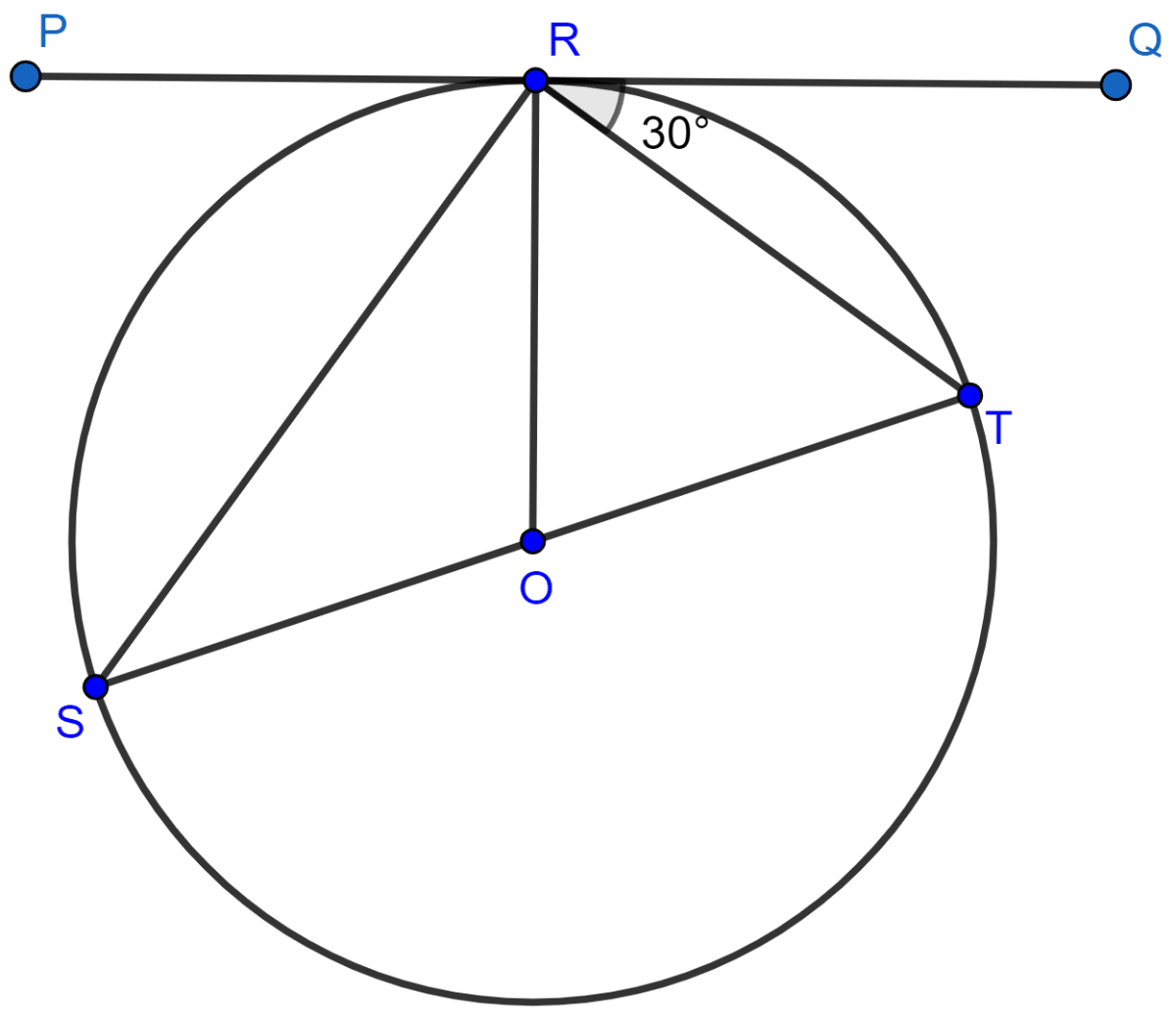
If PQ is a tangent to the circle at R; calculate :
(i) ∠PRS,
(ii) ∠ROT.
Given, O is the center of the circle and angle TRQ = 30°.
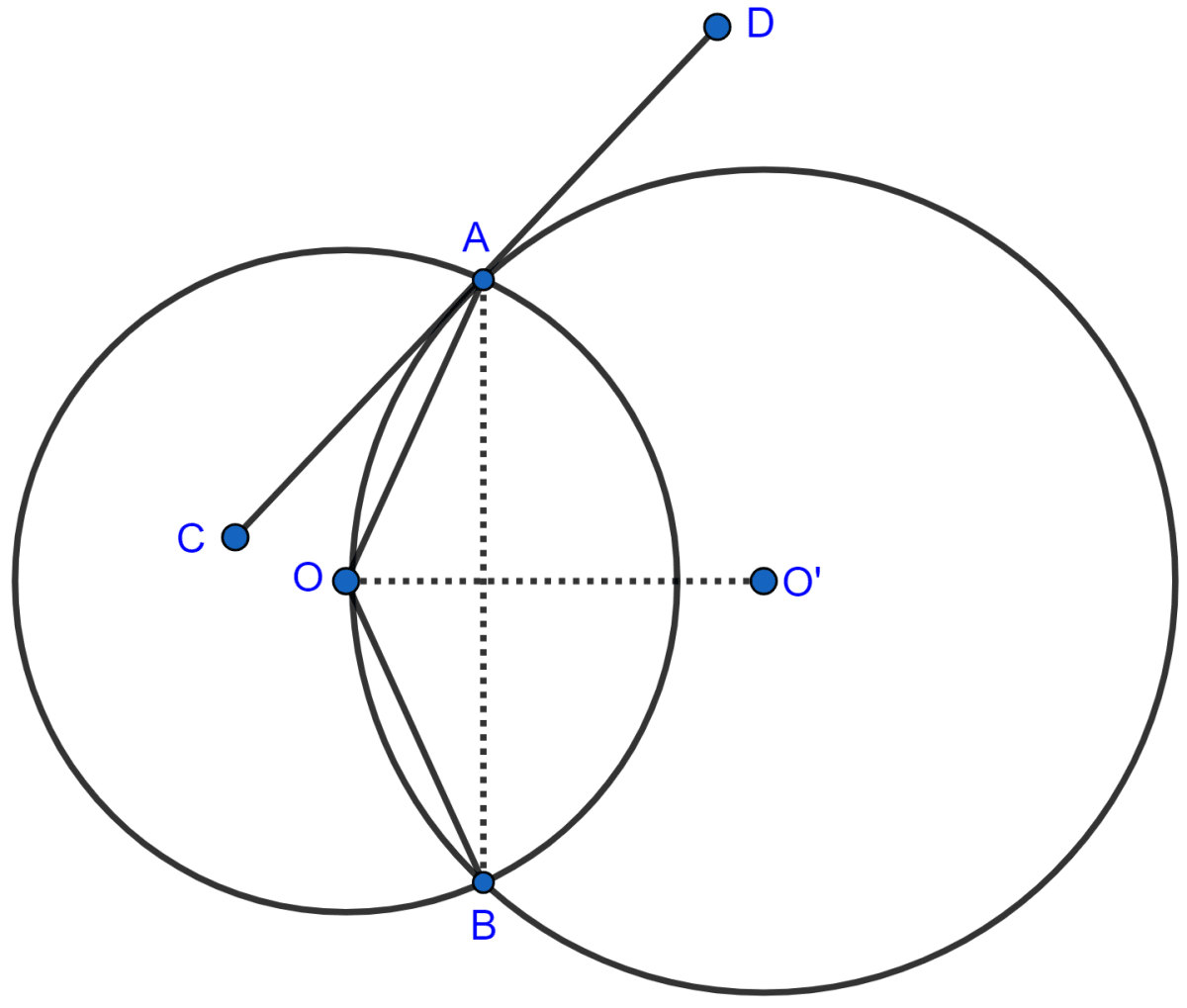
Two circles with centers O and O' are drawn to intersect each other at points A and B. Center O of one circle lies on the circumference of the other circle and CD is drawn tangent to the circle with center O' at A. Prove that OA bisects angle BAC.
In the figure; PA is a tangent to the circle, PBC is secant and AD bisects angle BAC. Show that triangle PAD is an isosceles triangle. Also, show that :
∠CAD = [∠PBA - ∠PAB]
![In the figure; PA is a tangent to the circle, PBC is secant and AD bisects angle BAC. Show that triangle PAD is an isosceles triangle. Also, show that ∠CAD = 1/2 [∠PBA - ∠PAB]. Tangents and Intersecting Chords, Concise Mathematics Solutions ICSE Class 10.](https://cdn1.knowledgeboat.com/img/cm10/q12-c18-ex-18-b-tangents-concise-maths-solutions-icse-class-10-1200x800.png)
Two circles intersect each other at point A and B. Their common tangent touches the circles at points P and Q as shown in the figure. Show that the angles PAQ and PBQ are supplementary.
![In the figure; PA is a tangent to the circle, PBC is secant and AD bisects angle BAC. Show that triangle PAD is an isosceles triangle. Also, show that ∠CAD = 1/2 [∠PBA - ∠PAB]. Tangents and Intersecting Chords, Concise Mathematics Solutions ICSE Class 10.](https://cdn1.knowledgeboat.com/img/cm10/q13-c18-ex-18-b-tangents-concise-maths-solutions-icse-class-10-1200x843.png)