Mathematics
Two circles intersect each other at point A and B. Their common tangent touches the circles at points P and Q as shown in the figure. Show that the angles PAQ and PBQ are supplementary.
![In the figure; PA is a tangent to the circle, PBC is secant and AD bisects angle BAC. Show that triangle PAD is an isosceles triangle. Also, show that ∠CAD = 1/2 [∠PBA - ∠PAB]. Tangents and Intersecting Chords, Concise Mathematics Solutions ICSE Class 10.](https://cdn1.knowledgeboat.com/img/cm10/q13-c18-ex-18-b-tangents-concise-maths-solutions-icse-class-10-1200x843.png)
Circles
12 Likes
Answer
Join AB.
![In the figure; PA is a tangent to the circle, PBC is secant and AD bisects angle BAC. Show that triangle PAD is an isosceles triangle. Also, show that ∠CAD = 1/2 [∠PBA - ∠PAB]. Tangents and Intersecting Chords, Concise Mathematics Solutions ICSE Class 10.](https://cdn1.knowledgeboat.com/img/cm10/q13-c18-ex-18-b-answer-tangents-concise-maths-solutions-icse-class-10-1200x848.png)
As, the angle between a tangent and a chord through the point of contact is equal to the angle in the alternate segment, we have :
From figure,
PQ is a tangent and AB is a chord.
∴ ∠QPA = ∠PBA [Angles in alternate segment are equal] ………(1)
Also,
∴ ∠PQA = ∠QBA [Angles in alternate segment are equal] ……….(2)
Adding (1) and (2) we get,
⇒ ∠QPA + ∠PQA = ∠PBA + ∠QBA ……….(3)
⇒ ∠PBA + ∠QBA = ∠PBQ ………..(4)
In △PAQ,
⇒ ∠QPA + ∠PQA + ∠PAQ = 180° [Angle sum property of triangle]
⇒ ∠QPA + ∠PQA = 180° - ∠PAQ
⇒ ∠PBA + ∠QBA = 180° - ∠PAQ [From (3)] ……….(5)
From (4) and (5), we get :
⇒ ∠PBQ = 180° - ∠PAQ
⇒ ∠PBQ + ∠PAQ = 180°.
Hence, proved that PAQ and PBQ are supplementary.
Answered By
7 Likes
Related Questions
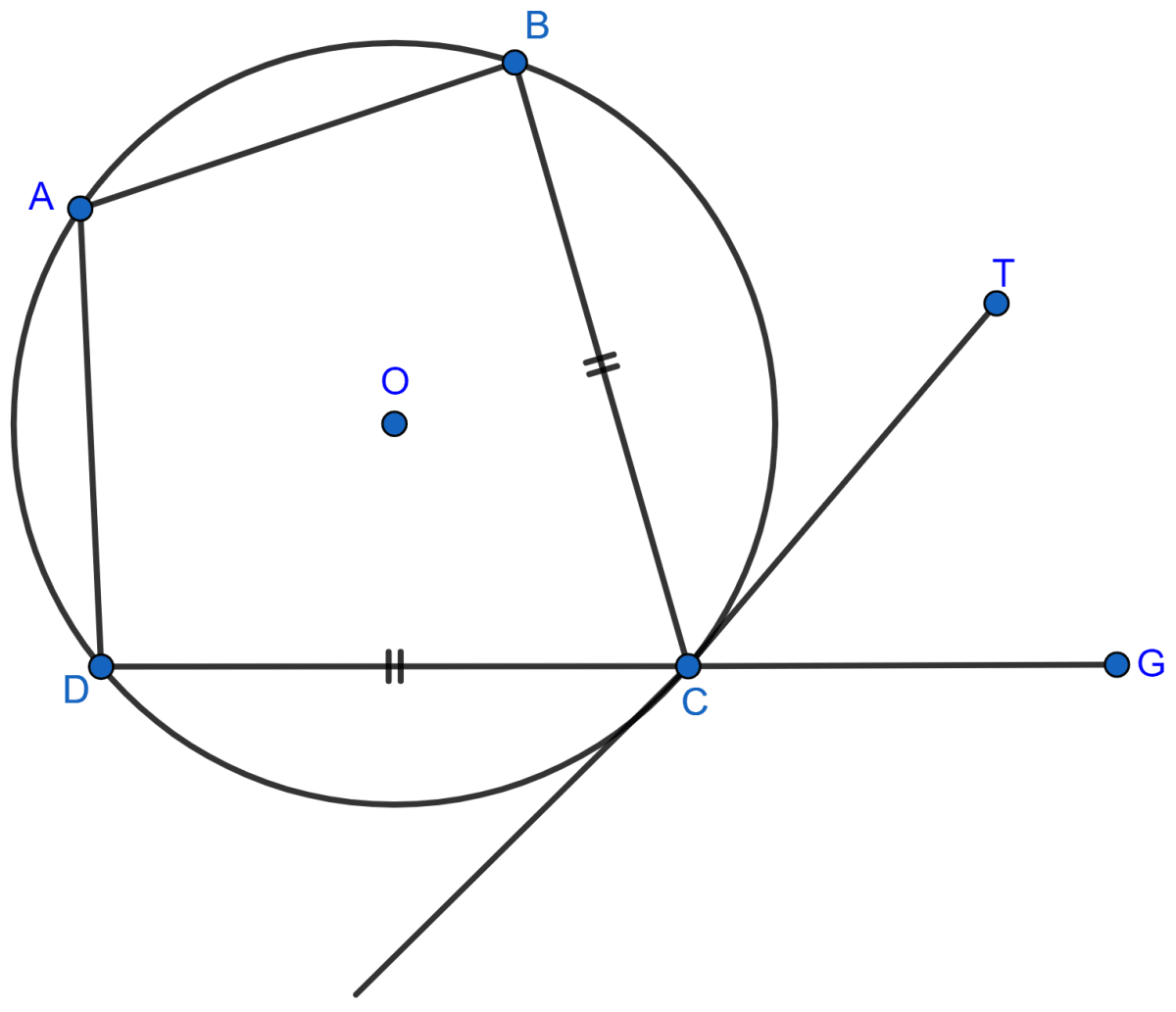
In the figure, ABCD is a cyclic quadrilateral with BC = CD. TC is tangent to the circle at point C and DC is produced to point G. If ∠BCG = 108° and O is the center of the circle, find :
(i) angle BCT
(ii) angle DOC
In the figure; PA is a tangent to the circle, PBC is secant and AD bisects angle BAC. Show that triangle PAD is an isosceles triangle. Also, show that :
∠CAD = [∠PBA - ∠PAB]
![In the figure; PA is a tangent to the circle, PBC is secant and AD bisects angle BAC. Show that triangle PAD is an isosceles triangle. Also, show that ∠CAD = 1/2 [∠PBA - ∠PAB]. Tangents and Intersecting Chords, Concise Mathematics Solutions ICSE Class 10.](https://cdn1.knowledgeboat.com/img/cm10/q12-c18-ex-18-b-tangents-concise-maths-solutions-icse-class-10-1200x800.png)
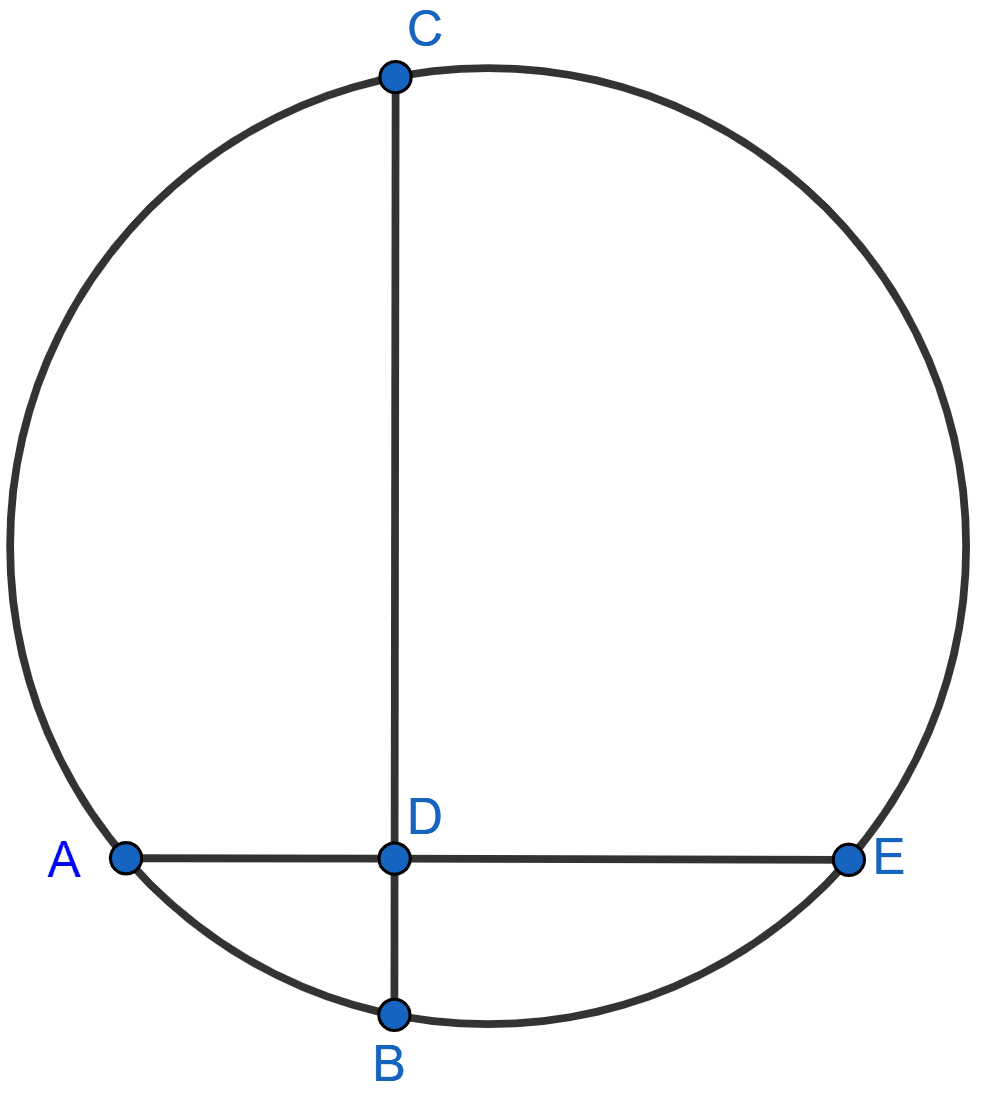
In the figure, chords AE and BC intersect each other at point D.
(i) If ∠CDE = 90°, AB = 5 cm, BD = 4 cm and CD = 9 cm; find DE.
(ii) If AD = BD, show that : AE = BC.
In the adjoining figure, O is the center of the circle and AB is a tangent to it at point B. ∠BDC = 65°. Find ∠BAO.
