Mathematics
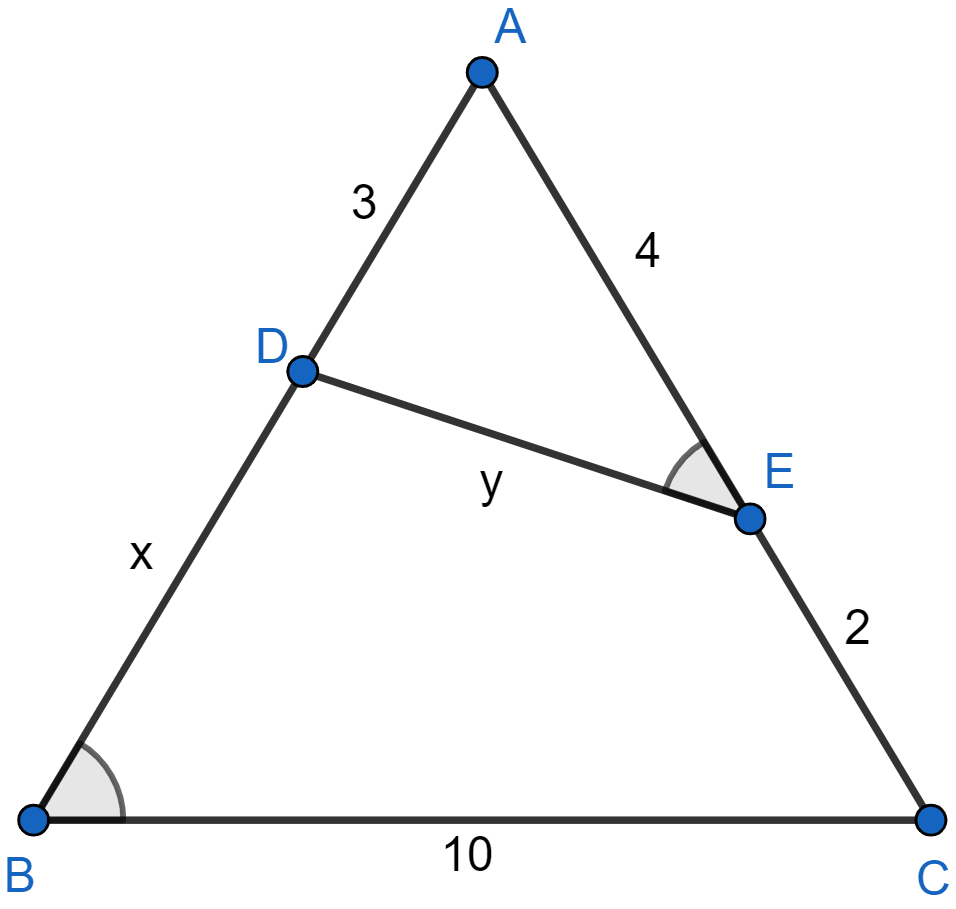
In the figure (1) given below, ∠AED = ∠ABC. Find the values of x and y.
Similarity
13 Likes
Answer
Considering △ABC and △ADE,
∠AED = ∠ABC (Given)
∠A = ∠A (Common angles)
Hence by AA axiom △ABC ~ △ADE.
Since, triangles are similar so ratio of their corresponding sides will be equal.
Similarly,
Hence, the value of x = 5 and y = 5.
Answered By
7 Likes
Related Questions
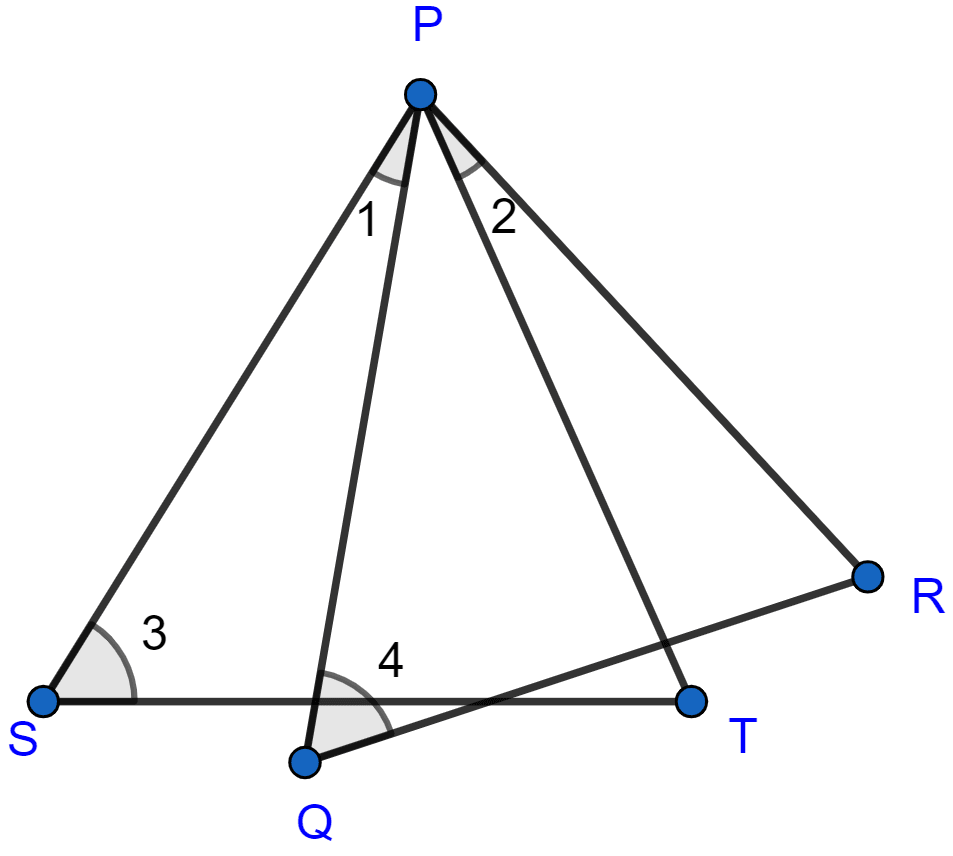
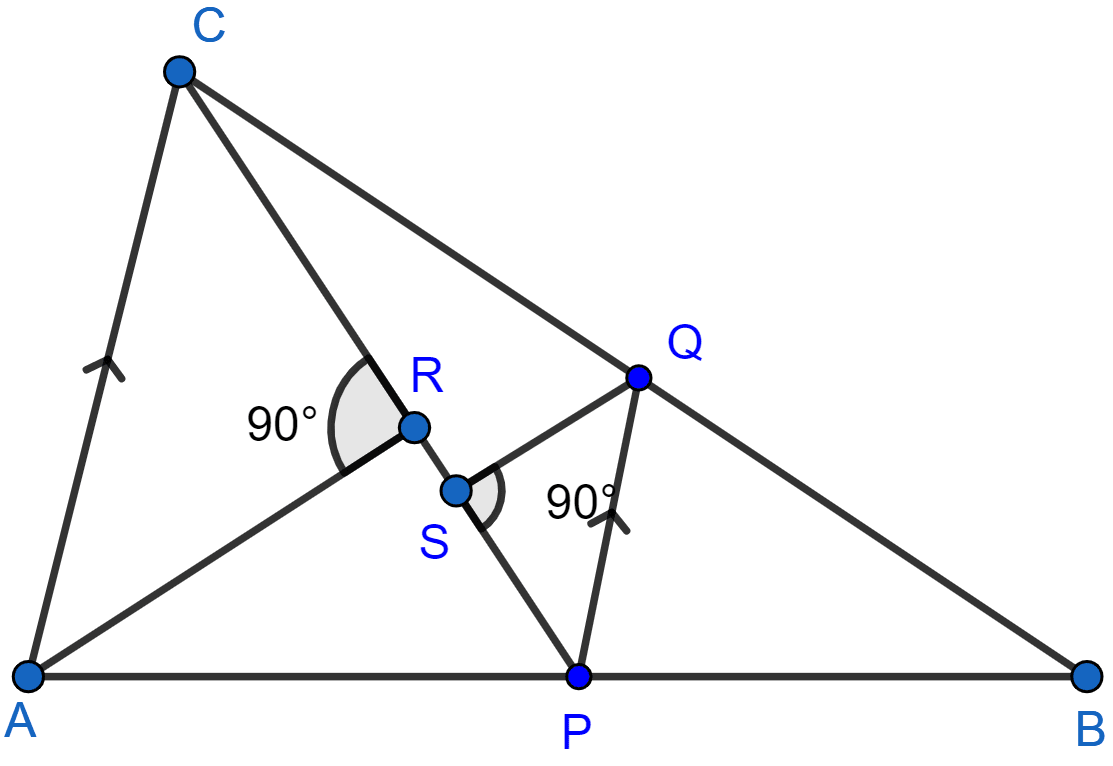
In the adjoining figure, ∠1 = ∠2 and ∠3 = ∠4. Show that PT × QR = PR × ST.
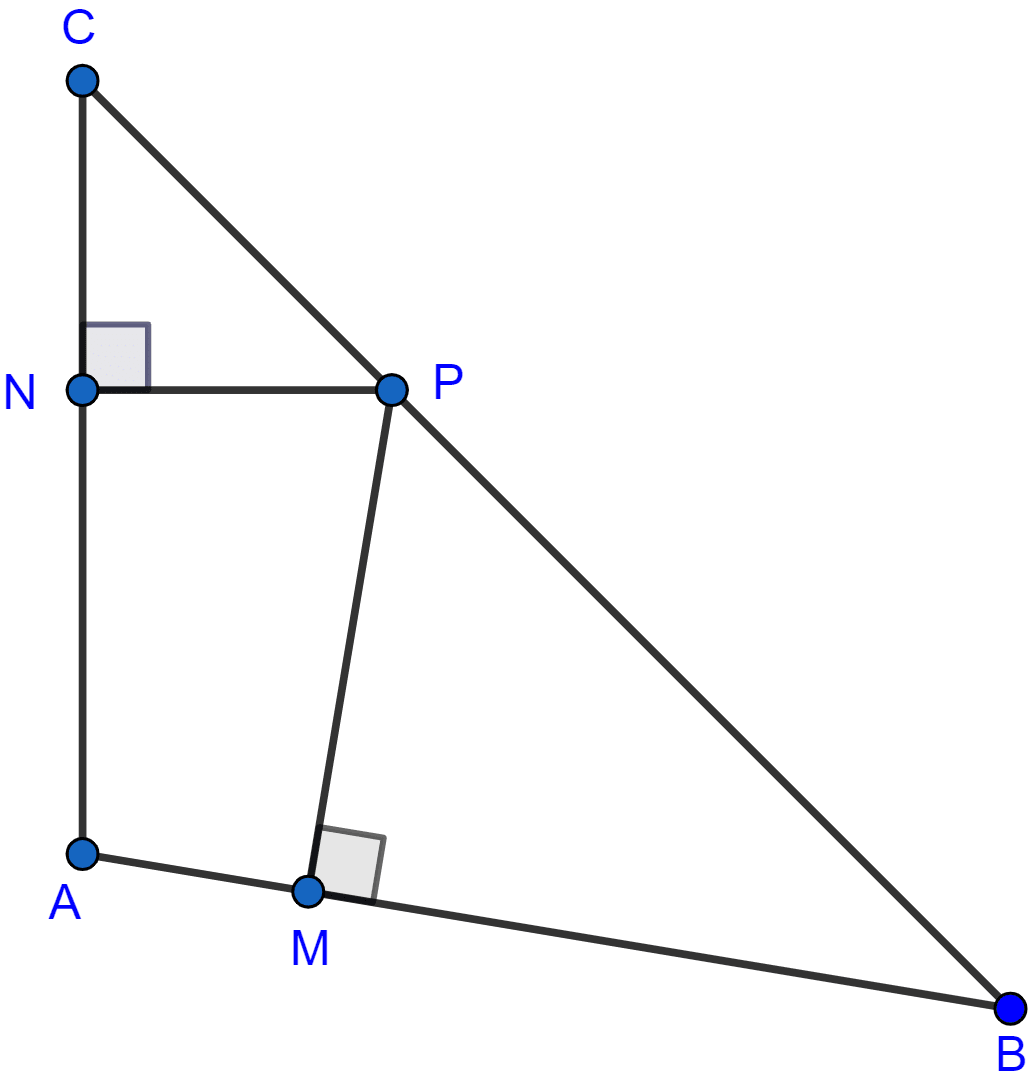
In the adjoining figure, AB = AC. If PM ⊥ AB and PN ⊥ AP, show that PM × PC = PN × PB.
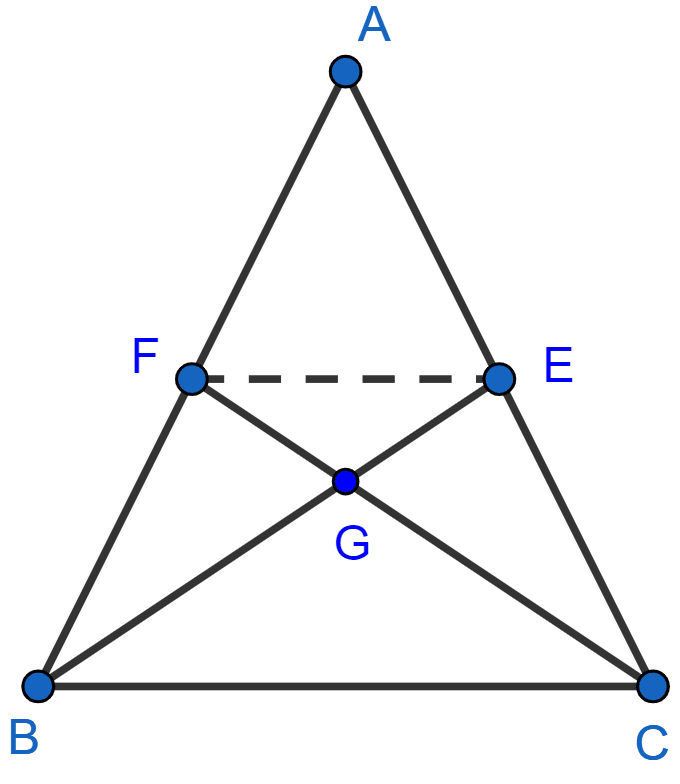
In the figure (2) given below, medians BE and CF of a △ABC meet at G. Prove that :
(i) △FGE ~ △CGB
(ii) BG = 2GE
In the given figure, P is a point on AB such that PB : AP = 3 : 4 and PQ || AC.
(i) Calculate PQ : AC.
(ii) If AR ⊥ CP, QS ⊥ CB and QS = 6 cm, calculate the length of AR.