Mathematics
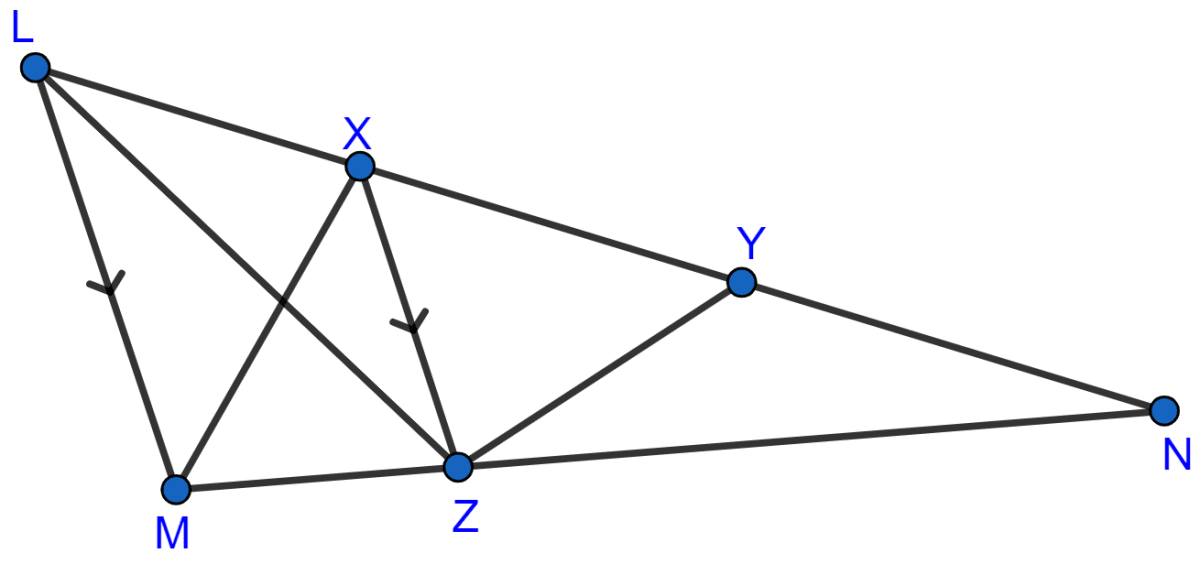
In the adjoining figure, X and Y are points on the side LN of triangle LMN. Through X, a line is drawn parallel to LM to meet MN at Z. Prove that area of ∆LZY = area of quad. MZYX.
Answer
From figure,
∆LZX and ∆MZX are on the same base XZ and between the same parallel lines LM and XZ.
∴ Area of ∆LZX = Area of ∆MZX
Adding area ∆XZY to both sides of the above equation we get,
⇒ area of ∆LZX + area ∆XZY = area ∆MZX + area ∆XZY
From figure,
∆LZX + ∆XZY = ∆LZY and ∆MZX + ∆XZY = MZYX.
∴ Area of ∆LZY = Area of quadrilateral MZYX.
Hence, proved that area of ∆LZY = area of quadrilateral MZYX.
Related Questions
Perpendiculars are drawn from a point within an equilateral triangle to the three sides. Prove that the sum of the three perpendiculars is equal to the altitude of the triangle.
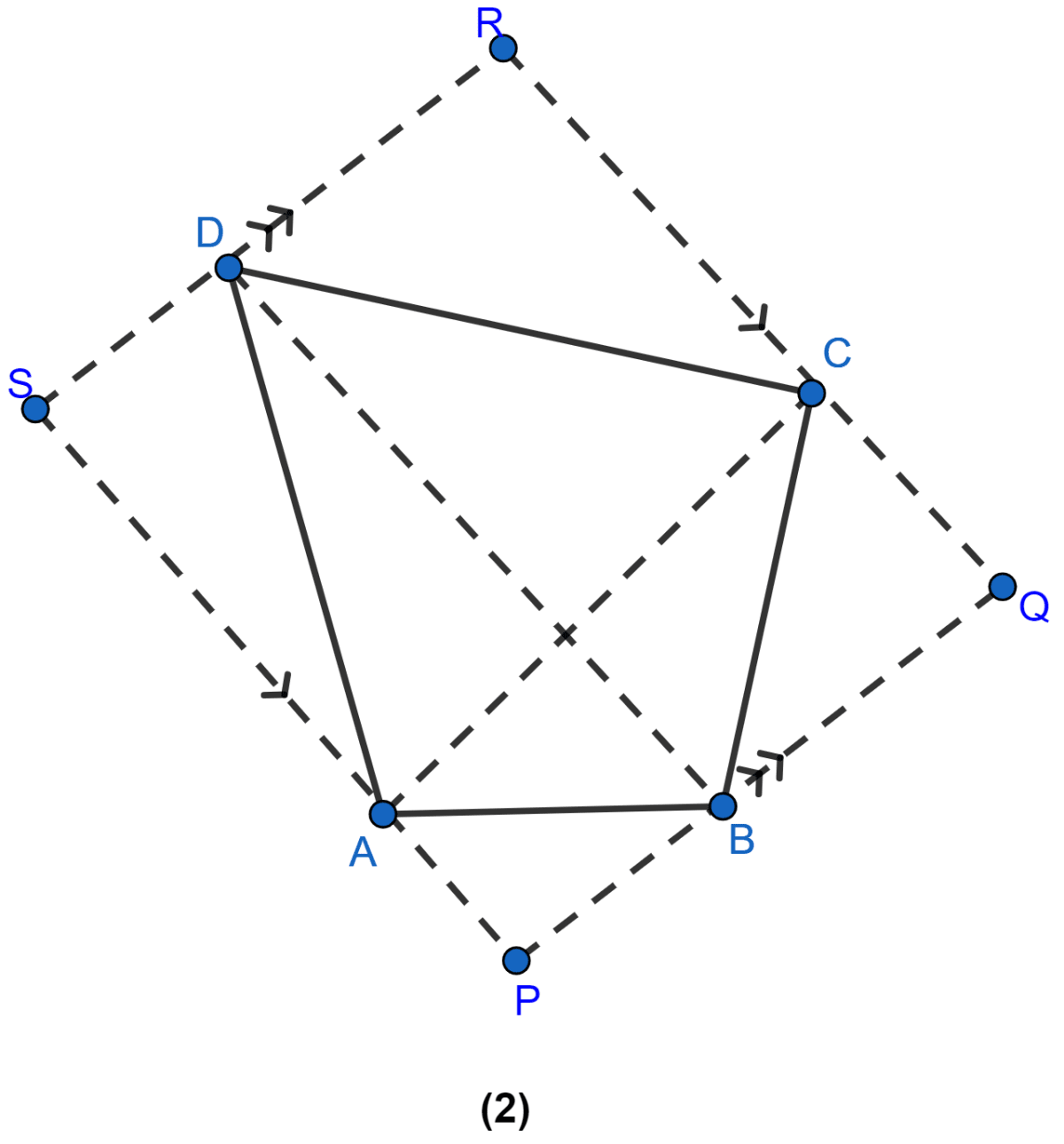
In the figure (2) given below, PQRS is a parallelogram formed by drawing lines parallel to the diagonals of a quadrilateral ABCD through its corners. Prove that area of || gm PQRS = 2 × area of quad. ABCD.
In the parallelogram ABCD, P is a point on the side AB and Q is a point on the side BC. Prove that
(i) area of ∆CPD = area of ∆AQD
(ii) area of ∆ADQ = area of ∆APD + area of ∆CPB.
If each diagonal of a quadrilateral divides it into two triangles of equal areas, then prove that the quadrilateral is a parallelogram.