Mathematics
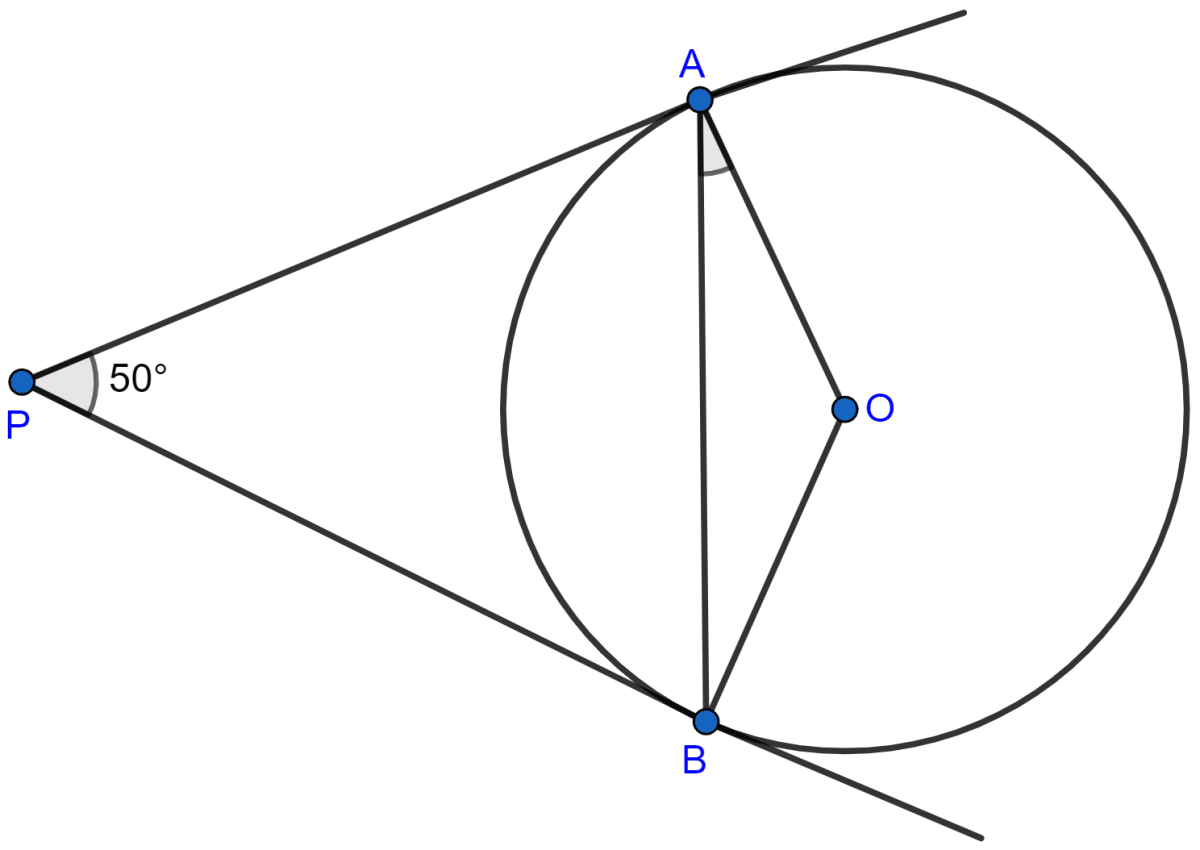
In the adjoining figure, PA and PB are tangents to a circle with centre O. If ∠APB = 50°, then ∠OAB is equal to
25°
30°
40°
50°
Answer
In the given figure,
PA and PB are tangents to the circle with centre O.
∠APB = 50°
Since sum of opposite angles of quadrilateral = 180°.
∴ ∠AOB + ∠APB = 180°
⇒ ∠AOB + 50° = 180°
⇒ ∠AOB = 180° - 50° = 130°.
In △OAB,
OA = OB (Radius of the same circle)
Hence, △OAB is an isosceles triangle with ∠OAB = ∠OBA.
Since, sum of angles of a triangle = 180°.
In △OAB,
⇒ ∠OAB + ∠OBA + ∠AOB = 180°
⇒ ∠OAB + ∠OAB + 130° = 180°
⇒ 2∠OAB = 180° - 130°
⇒ 2∠OAB = 50°
⇒ ∠OAB = 25°.
Hence, Option 1 is the correct option.
Related Questions
In the adjoining figure, O is the centre of a circle and PQ is a chord. If the tangent PR at P makes an angle of 50° with PQ, then ∠POQ is
100°
80°
90°
75°

In the adjoining figure, sides BC, CA and AB of △ABC touch a circle at the points P, Q and R respectively. If PC = 5 cm, AR = 4 cm and RB = 6 cm, then the perimeter of △ABC is
60 cm
45 cm
30 cm
15 cm

In the adjoining figure, sides BC, CA and AB of △ABC touch a circle at point D, E and F respectively. If BD = 4 cm, DC = 3 cm and CA = 8 cm, then the length of side AB is
12 cm
11 cm
10 cm
9 cm

If radii of two concentric circles are 4 cm and 5 cm, then the length of each chord of one circle which is tangent to the other is
3 cm
6 cm
9 cm
1 cm