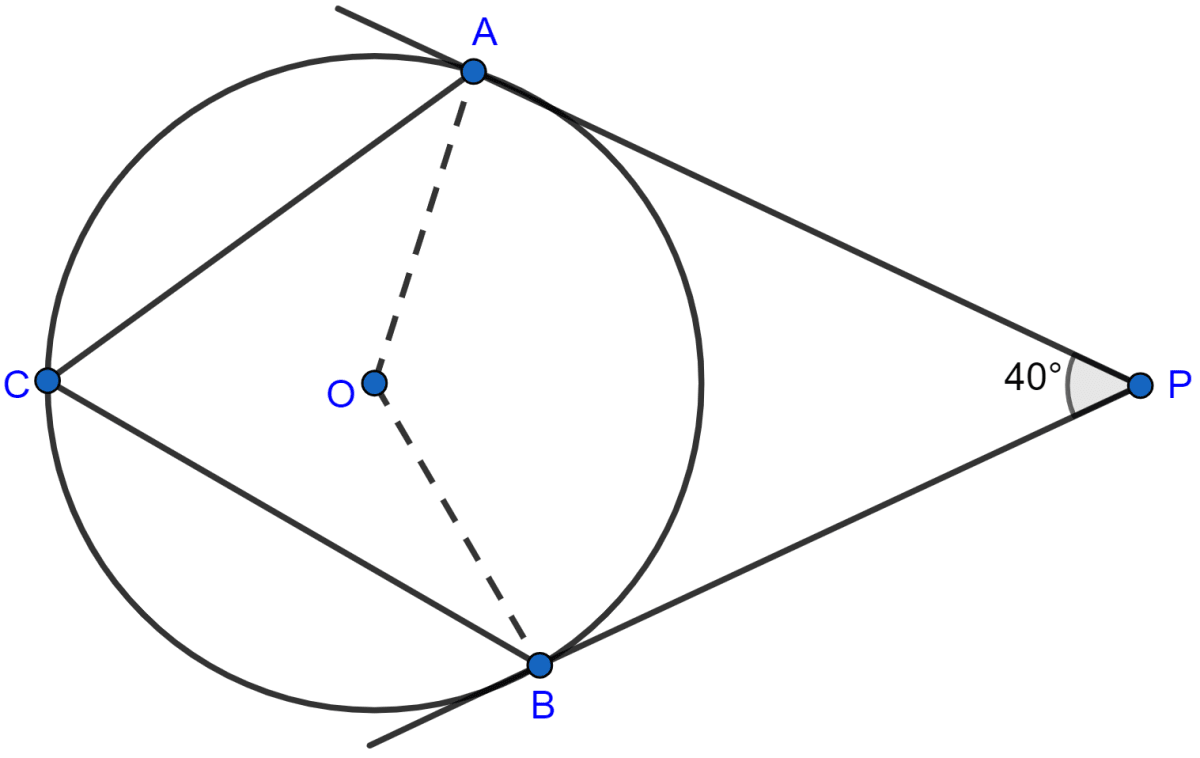
In the adjoining figure, PA and PB are tangents at points A and B respectively to a circle with centre O. If C is a point on the circle and ∠APB = 40°, then ∠ACB is equal to

1. 80°

2. 70°

3. 90°

4. 140°

Join OP.

We know that the tangents are equally inclined to the line joining the point and the centre of the circle.

∴ ∠APO = $\dfrac{∠\text{APB}}{2} = \dfrac{40°}{2}$ = 20°.

AP ⊥ OA.

∴ ∠OAP = 90°.

In right angle triangle OAP,

⇒ ∠APO + ∠AOP + ∠OAP = 180°
⇒ 20° + ∠AOP + 90° = 180°
⇒ ∠AOP + 110° = 180°
⇒ ∠AOP = 180° - 110° = 70°.

As the tangents subtends equal angles at centre.

∴ ∠BOP = ∠AOP = 70°.

∠AOB = ∠AOP + ∠BOP = 70° + 70° = 140°.

Arc AB subtends ∠AOB at center and ∠ACB on the remaining part of the circle.

∴ ∠AOB = 2∠ACB

⇒ ∠ACB = $\dfrac{1}{2} \times$ ∠AOB

⇒ ∠ACB = $\dfrac{1}{2} \times 140°$

⇒ ∠ACB = 70°.

Hence, Option 2 is the correct option.