Mathematics
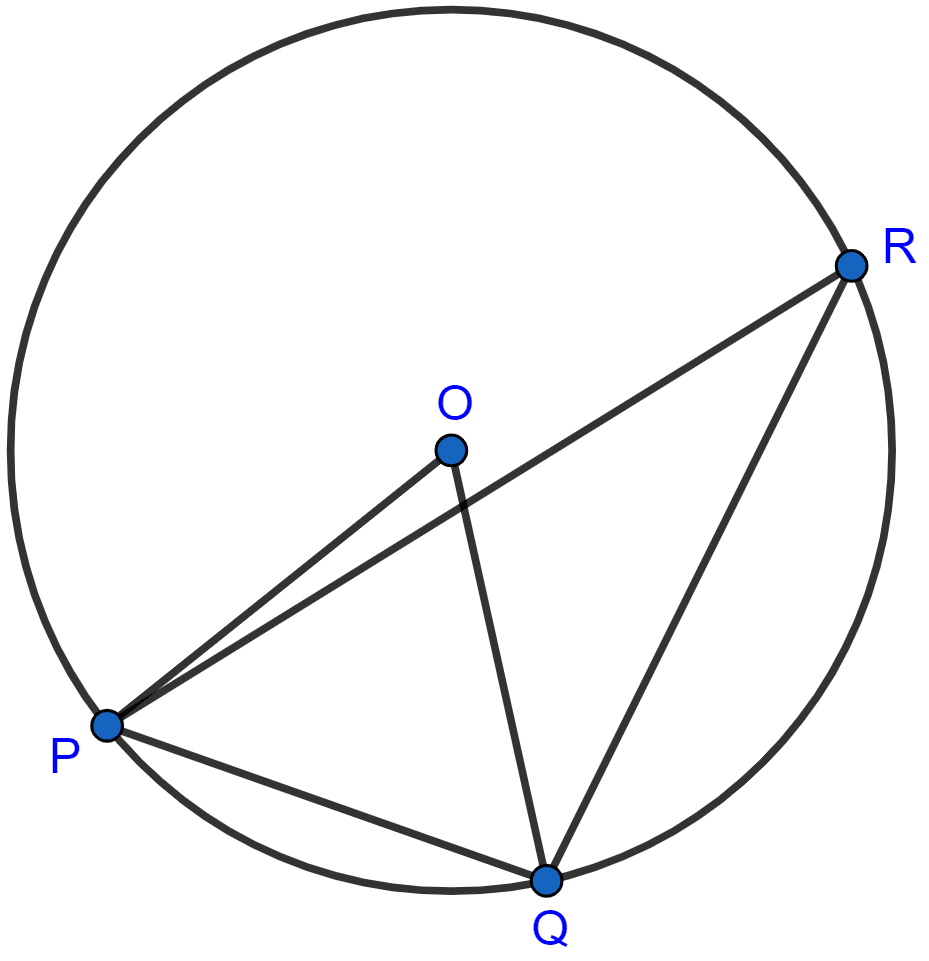
In the adjoining figure, O is the centre of the circle. If the length of the chord PQ is equal to the radius of the circle, then ∠PRQ is
60°
45°
30°
15°
Circles
22 Likes
Answer
From figure,
In △OPQ,
OP = OQ = PQ = (Radius of the circle.)
Hence, △OPQ is an equilateral triangle.
∴ ∠POQ = 60° (∵ all angles of an equilateral triangle = 60°.)
Arc PQ subtends ∠POQ at centre and ∠PRQ at remaining part of circle.
∠POQ = 2∠PRQ (∵ angle subtended at centre is double the angle subtended at remaining part of circle.)
60° = 2∠PRQ
∠PRQ = = 30°.
Hence, Option 3 is the correct option.
Answered By
12 Likes
Related Questions
ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC = 140°, then ∠BAC is equal to
80°
50°
40°
30°
In the adjoining figure, O is the centre of the circle. If ∠BAO = 60°, then ∠ADC is equal to
30°
45°
60°
120°

In the adjoining figure, if O is the centre of the circle then the value of x is
18°
20°
24°
36°

From a point which is at a distance of 13 cm from the centre O of a circle of radius 5 cm, the pair of tangents PQ and PR to the circle are drawn. Then the area of the quadrilateral PQOR is
60 cm2
65 cm2
30 cm2
32.5 cm2