Mathematics
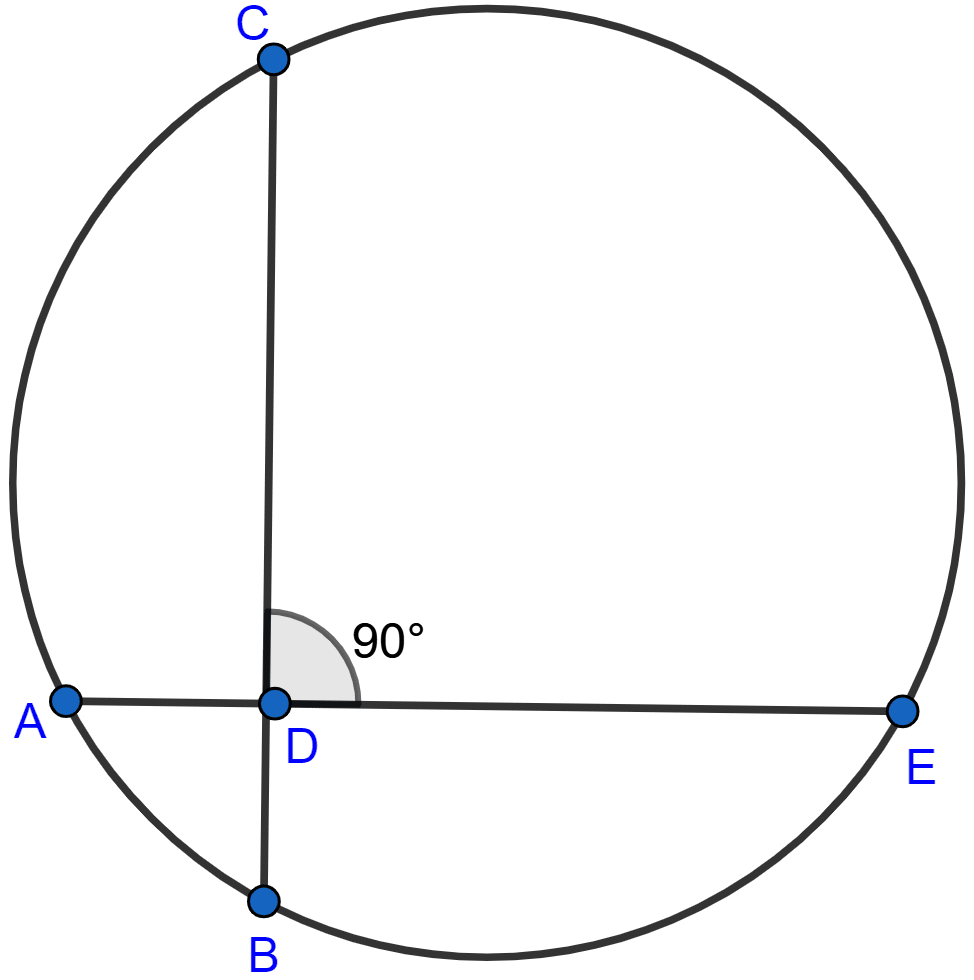
In the adjoining figure, AE and BC intersect each other at point D. If ∠CDE = 90°, AB = 5 cm, BD = 4 cm and CD = 9 cm, find DE.
Circles
29 Likes
Answer
Join A and B as shown in the figure below:
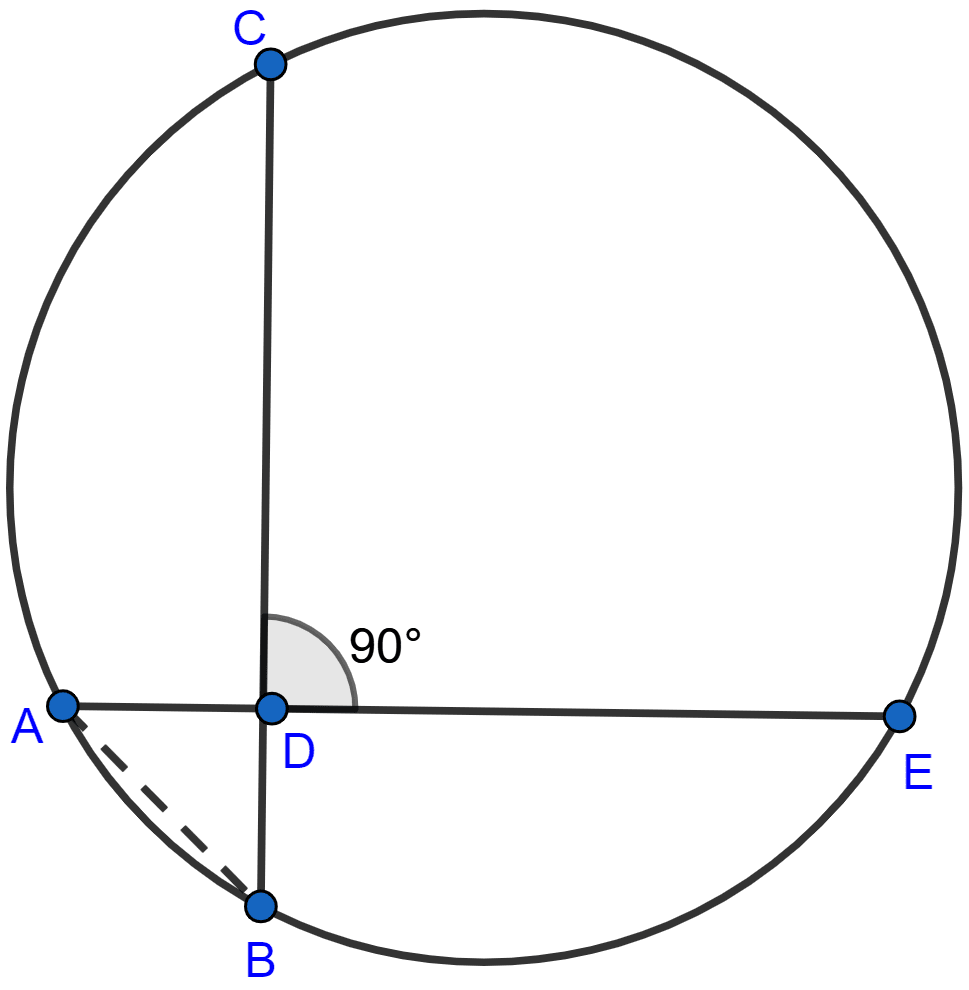
From figure,
Since, ∠CDE = 90° so, ∠ADB = 90° (∵ vertically opposite angles are equal.)
In right angle triangle △ADB, by pythagoras theorem,
Chords AE and CB intersect each other at D.
In △ADB and △CDE,
∠BAD = ∠DCE (∵ angles in same segment are equal.)
∠ADB = ∠CDE (∵ vertically opposite angles are equal.)
△ADB ~ △CDE. (By AA axiom)
Since △ADB ~ △CDE, Hence, the ratio of corresponding sides are equal.
∴ AD × DE = CD × BD
⇒ 3 × DE = 9 × 4
⇒ DE =
⇒ DE = 12 cm.
Hence, the length of DE = 12 cm.
Answered By
22 Likes
Related Questions
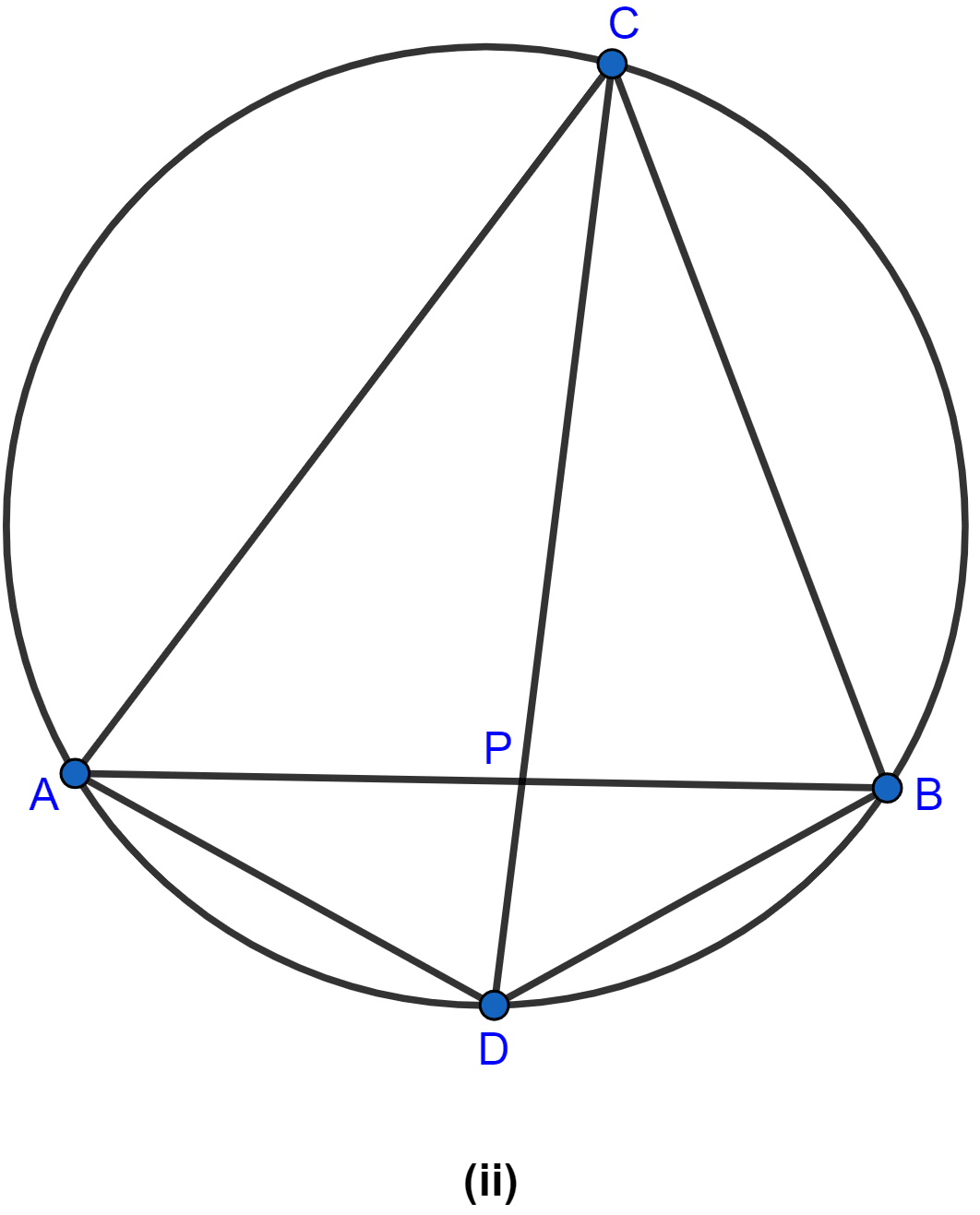
In the figure (i) given below, chords AB and CD of a circle intersect at E.
(i) Prove that triangles ADE and CBE are similar.
(ii) Given DC = 12 cm, DE = 4 cm and AE = 16 cm, calculate the length of BE.

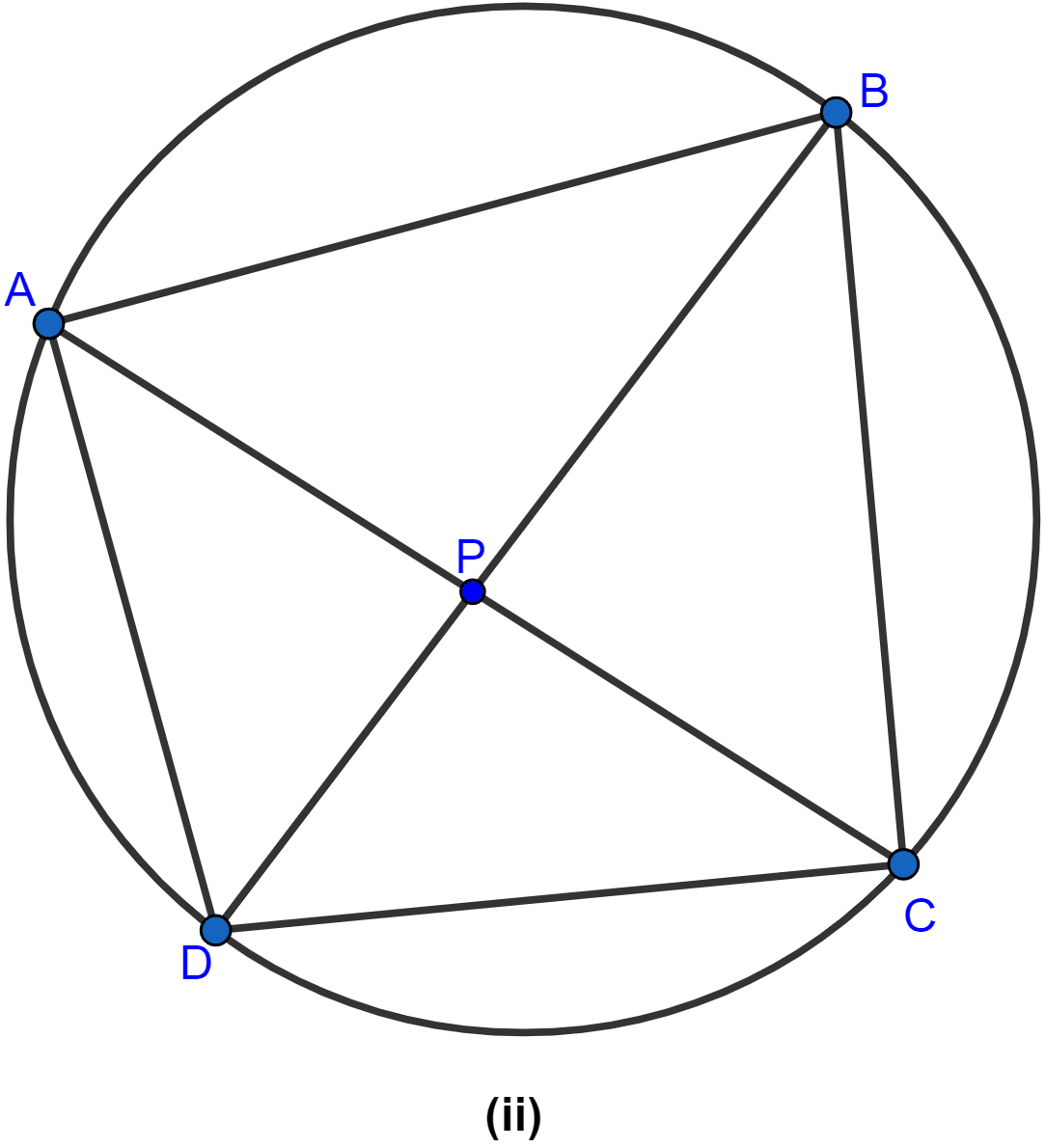
In the figure (ii) given below, AB and CD are two intersecting chords of a circle. Name two triangles which are similar. Hence, calculate CP given that AP = 6 cm, PB = 4 cm, and CD = 14 cm (PC > PD).
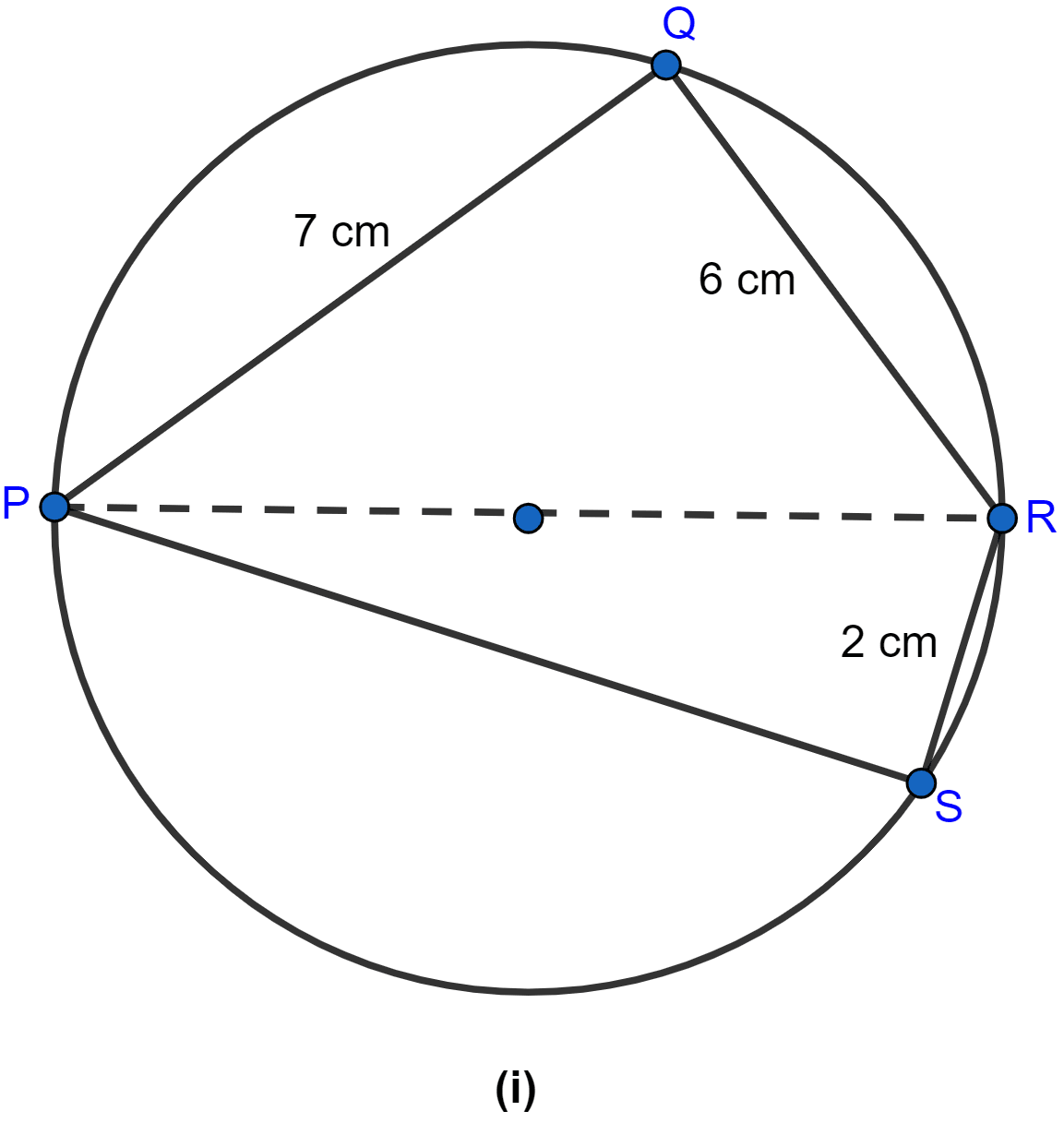
In the figure (i) given below, PR is a diameter of the circle, PQ = 7 cm, QR = 6 cm and RS = 2 cm. Calculate the perimeter of the cyclic quadrilateral PQRS.
In the figure (ii) given below, the diagonals of a cyclic quadrilateral ABCD intersect in P and the area of the triangle APB is 24 cm2. If AB = 8 cm and CD = 5 cm, calculate the area of △DPC.