Mathematics
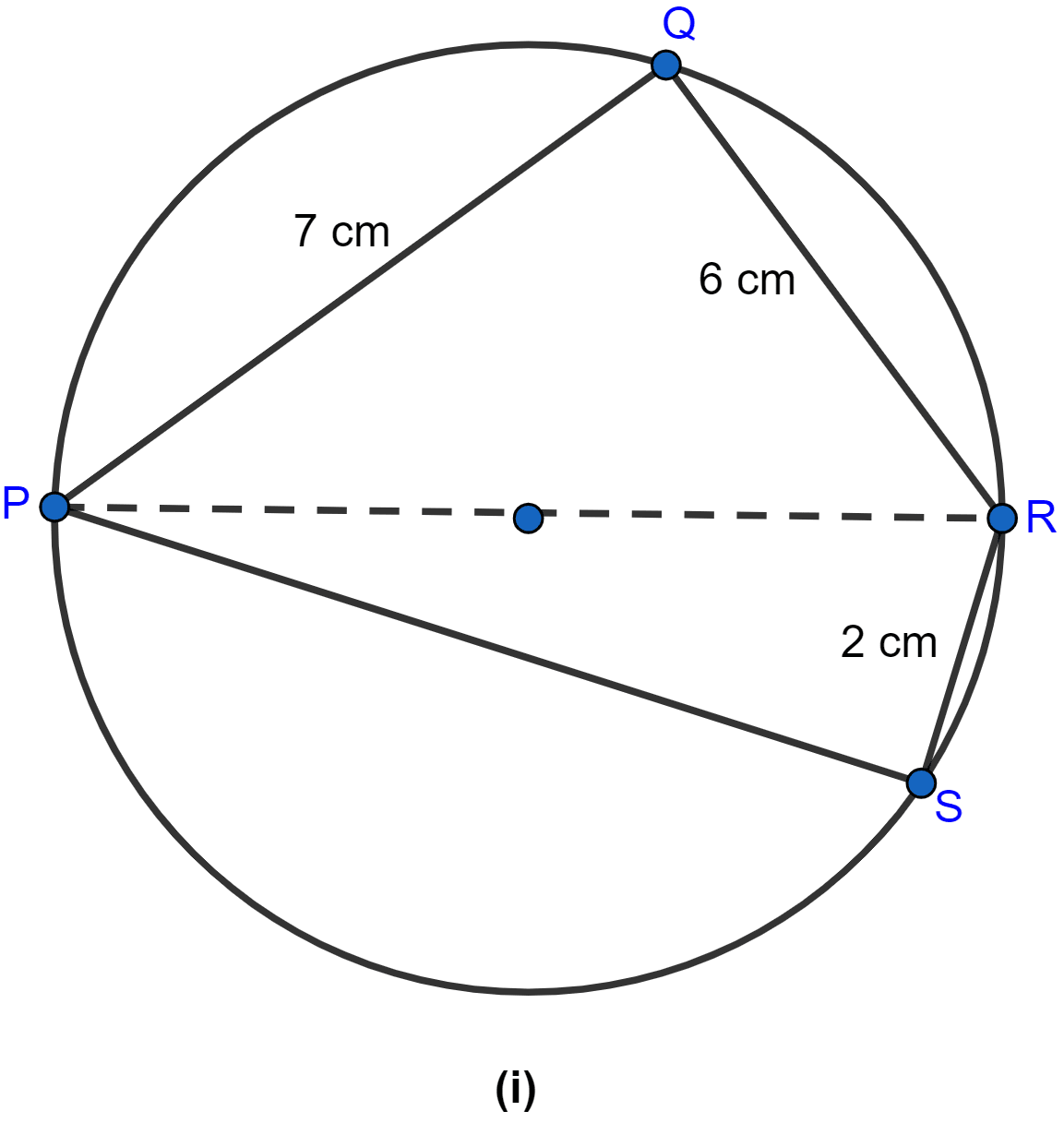
In the figure (i) given below, PR is a diameter of the circle, PQ = 7 cm, QR = 6 cm and RS = 2 cm. Calculate the perimeter of the cyclic quadrilateral PQRS.
Circles
24 Likes
Answer
We know that ∠PQR = 90° as angle in semicircle is equal to 90°.
So, △PQR is a right angled triangle.
By pythagoras theorem,
In △PRS,
∠PSR = 90° as angle in semicircle is equal to 90°.
So, △PRS is a right angled triangle.
By pythagoras theorem,
Perimeter of PQRS = PQ + QR + RS + SP = 7 + 6 + 2 + 9 = 24 cm.
Hence, the perimeter of cyclic quadrilateral is 24 cm.
Answered By
12 Likes
Related Questions
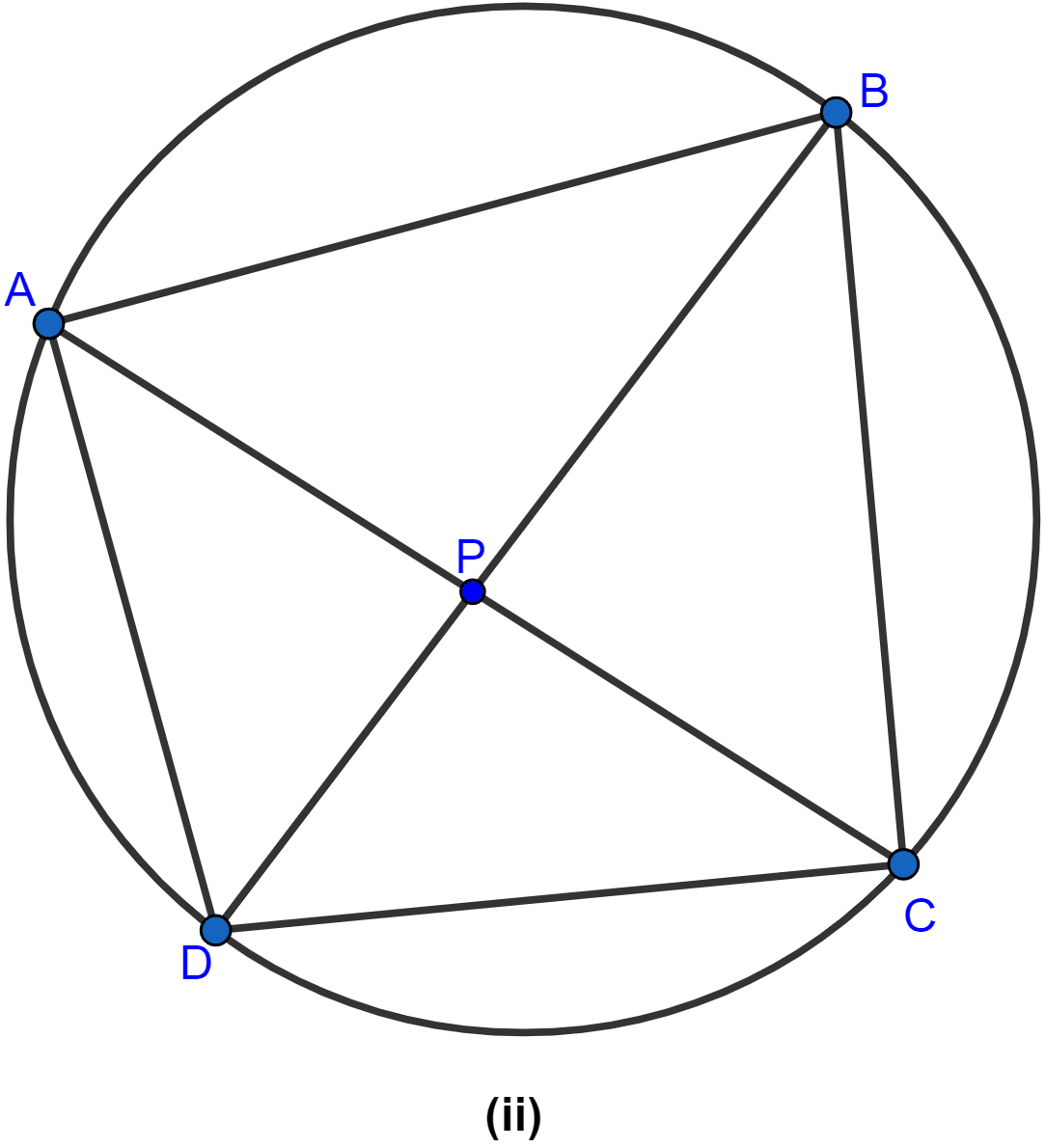
In the figure (ii) given below, AB and CD are two intersecting chords of a circle. Name two triangles which are similar. Hence, calculate CP given that AP = 6 cm, PB = 4 cm, and CD = 14 cm (PC > PD).
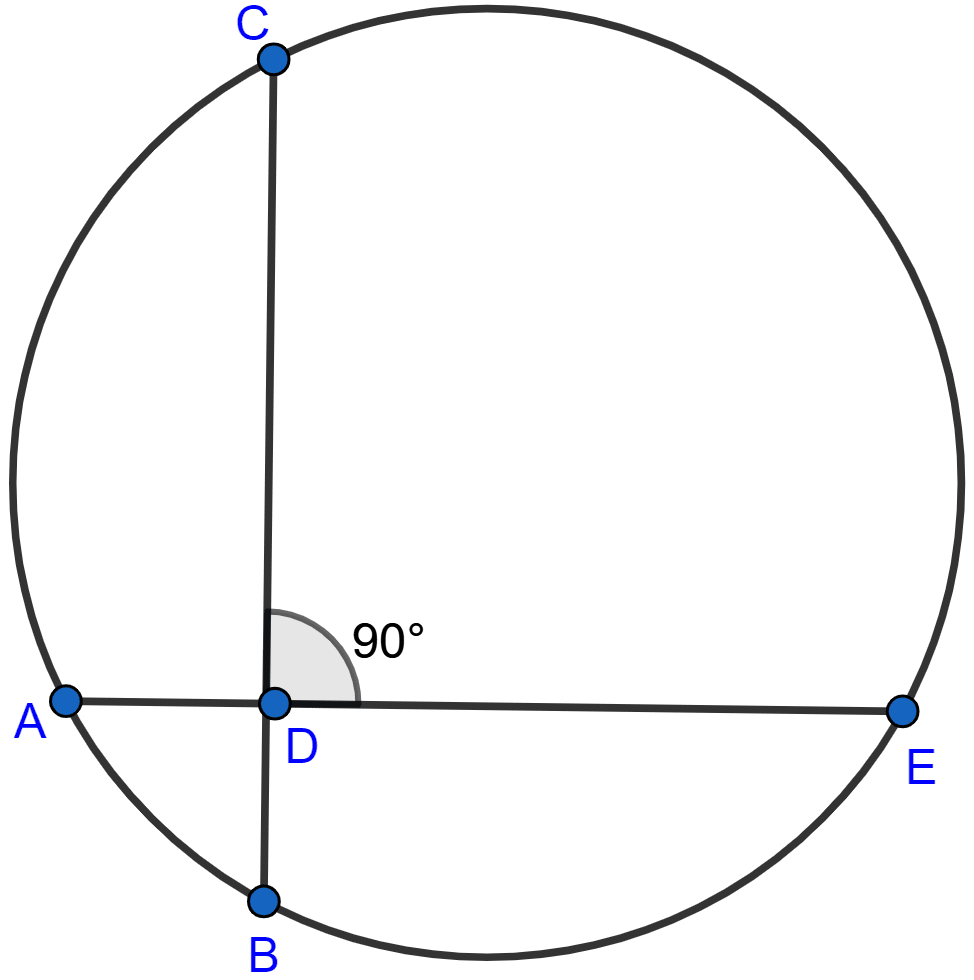
In the adjoining figure, AE and BC intersect each other at point D. If ∠CDE = 90°, AB = 5 cm, BD = 4 cm and CD = 9 cm, find DE.
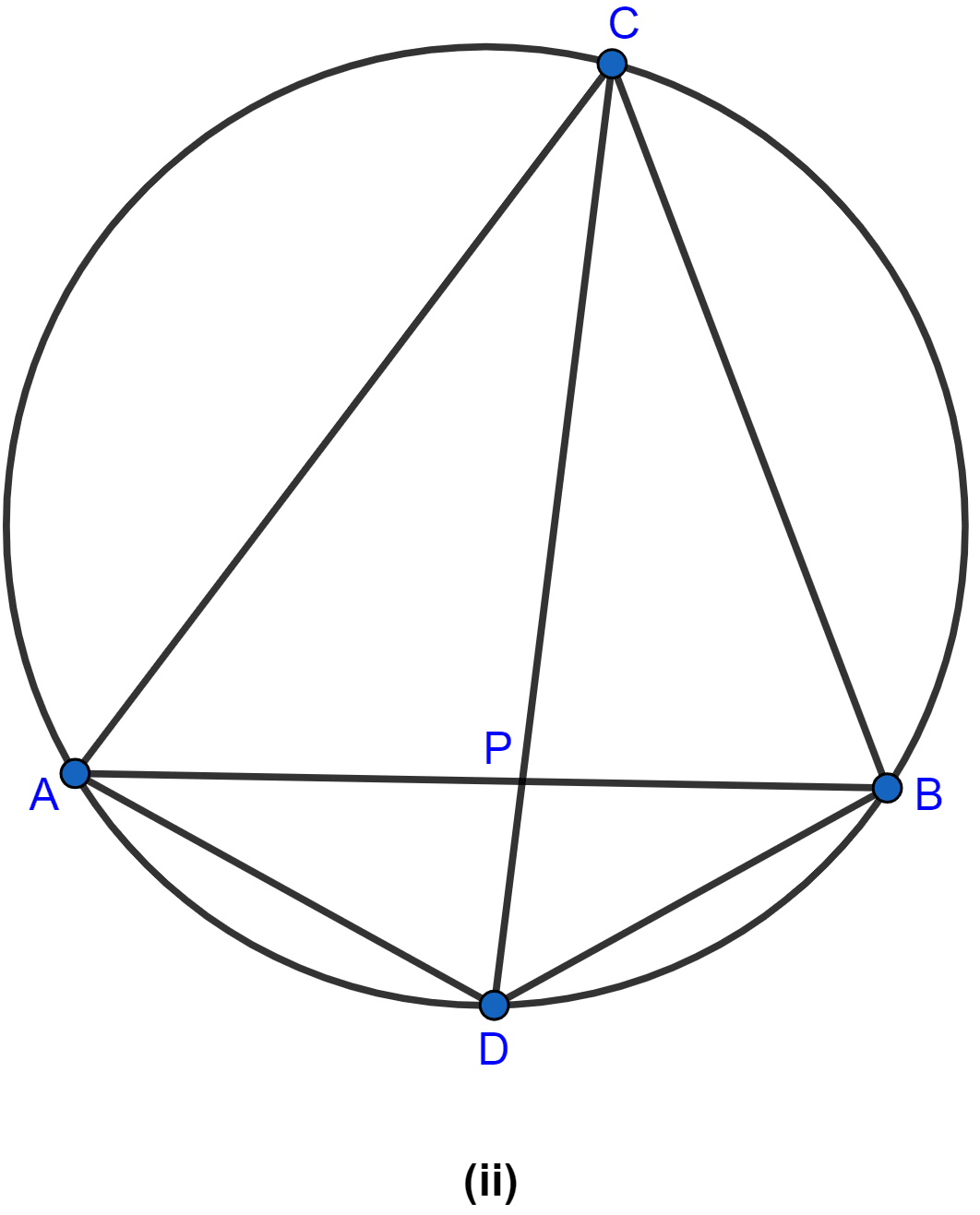
In the figure (ii) given below, the diagonals of a cyclic quadrilateral ABCD intersect in P and the area of the triangle APB is 24 cm2. If AB = 8 cm and CD = 5 cm, calculate the area of △DPC.
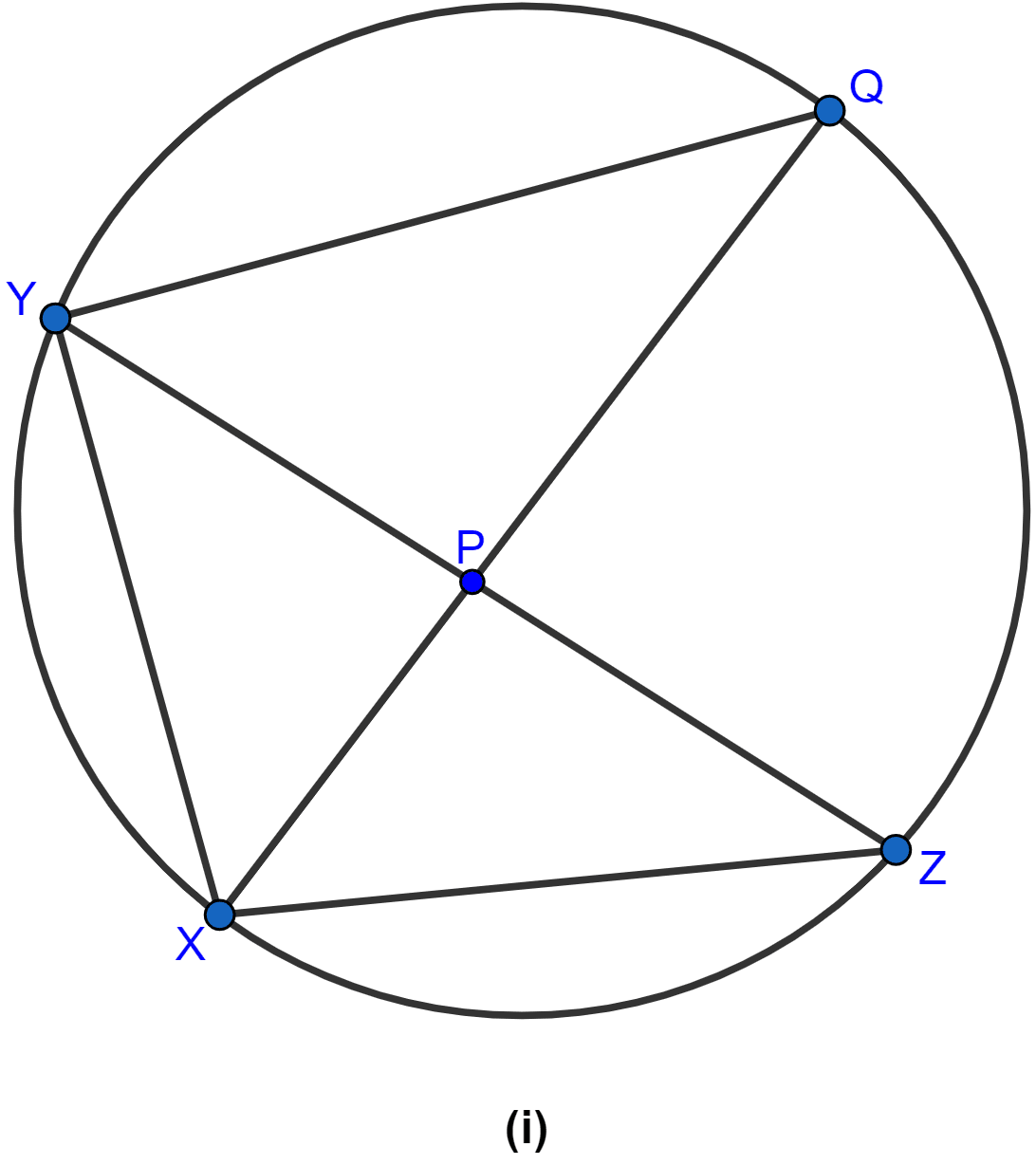
In the figure (i) given below, QPX is the bisector of ∠YXZ of the triangle XYZ. Prove that XY : XQ = XP : XZ.