Mathematics
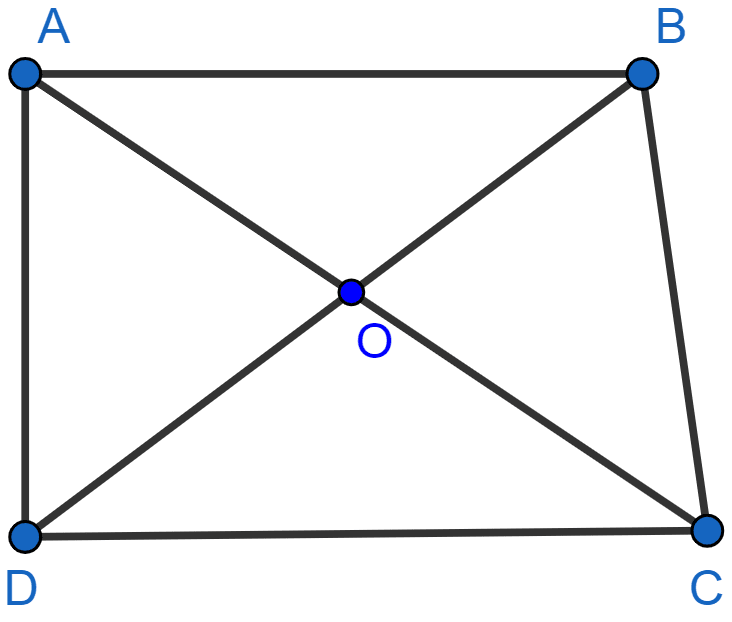
In the adjoining figure, ABCD is a trapezium in which AB ∥ DC. The diagonals AC and BD intersect at O. Prove that
Using the above result, find the value(s) of x if OA = 3x - 19, OB = x - 4, OC = x - 3 and OD = 4.
Similarity
52 Likes
Answer
Consider triangle AOB and COD,
∠ AOB = ∠ COD [Vertically opposite angles]
∠ OAB = ∠ OCD [Alternate angles]
So, by AA rule of similarity △AOB ~ △COD.
As triangles are similar, ratio of sides will be similar,
Putting values of sides from question in equation,
Hence, the value of x = 8 or 11.
Answered By
28 Likes
Related Questions
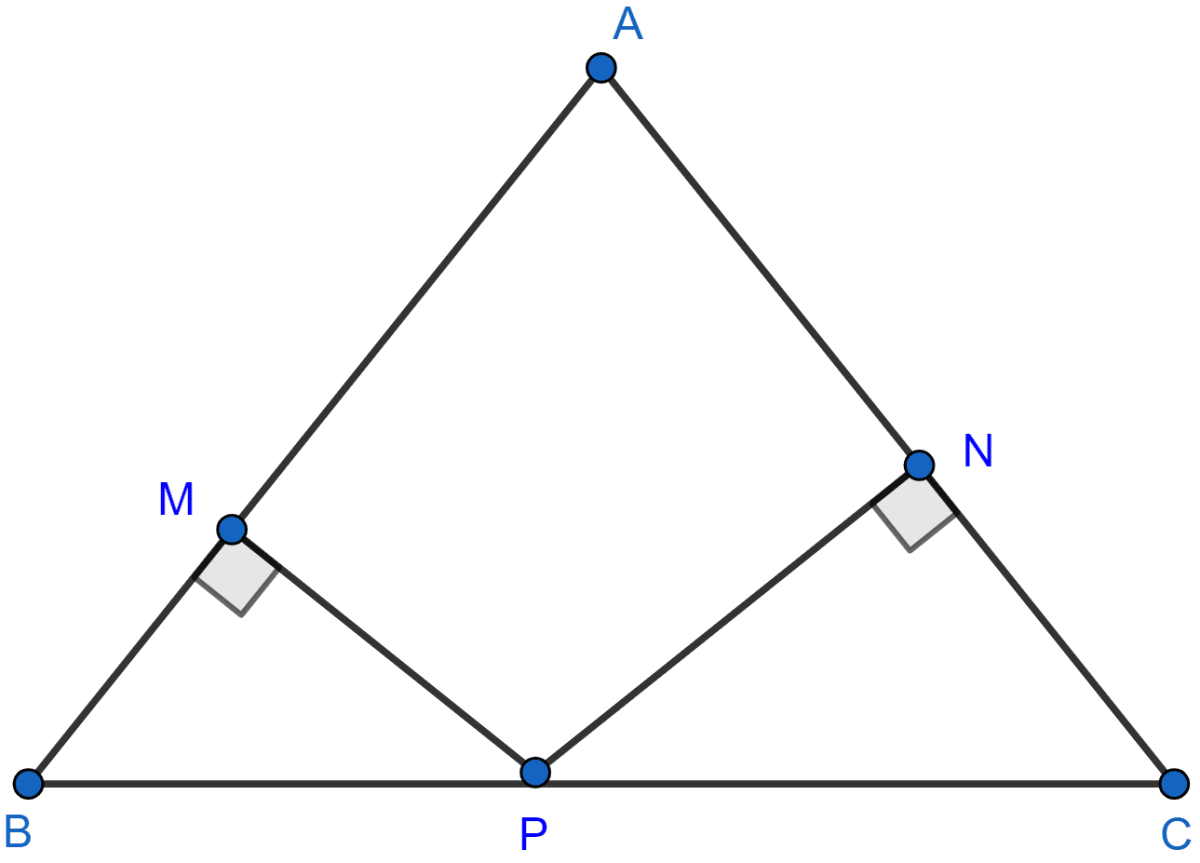
In the adjoining figure, ABC is a triangle in which AB = AC. P is a point on the side BC such that PM ⊥ AB and PN ⊥ AC. Prove that BM × NP = CN × MP.
Prove that the ratio of the perimeters of two similar triangles is the same as the ratio of their corresponding sides.
In the figure (1) given below, AB, EF and CD are parallel lines. Given that AB = 15 cm, EG = 5 cm, GC = 10 cm and DC = 18 cm. Calculate
(i) EF (ii) AC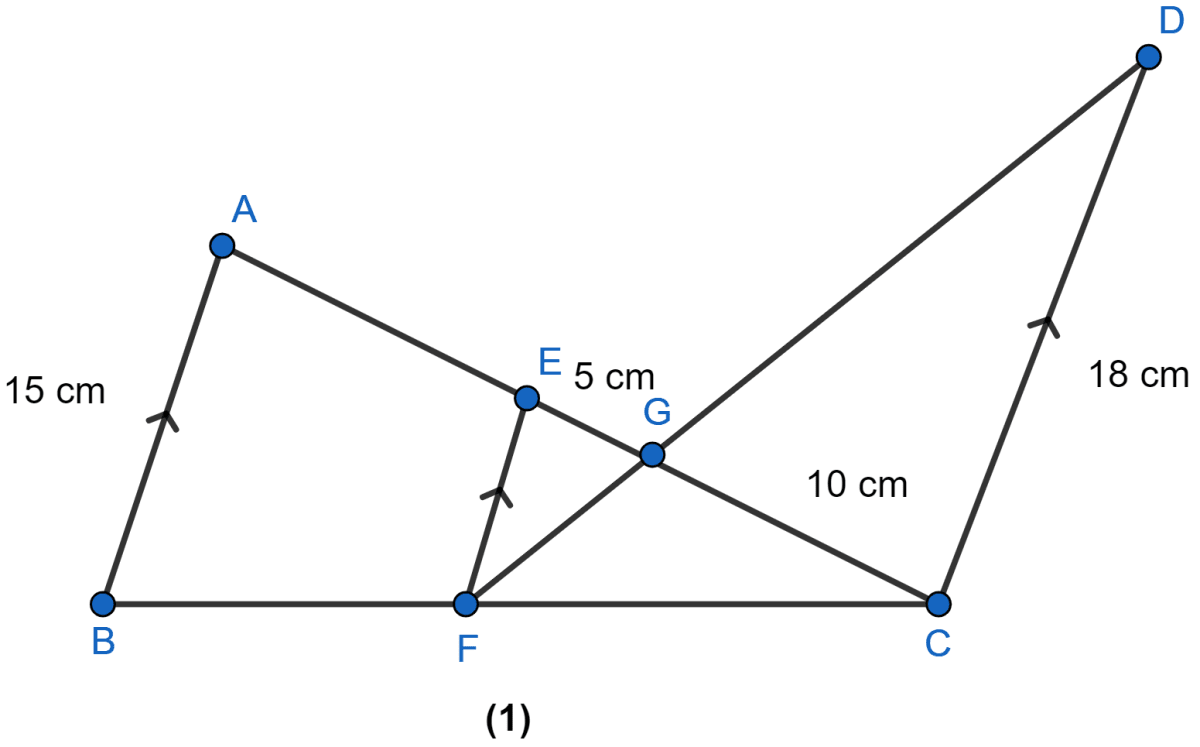
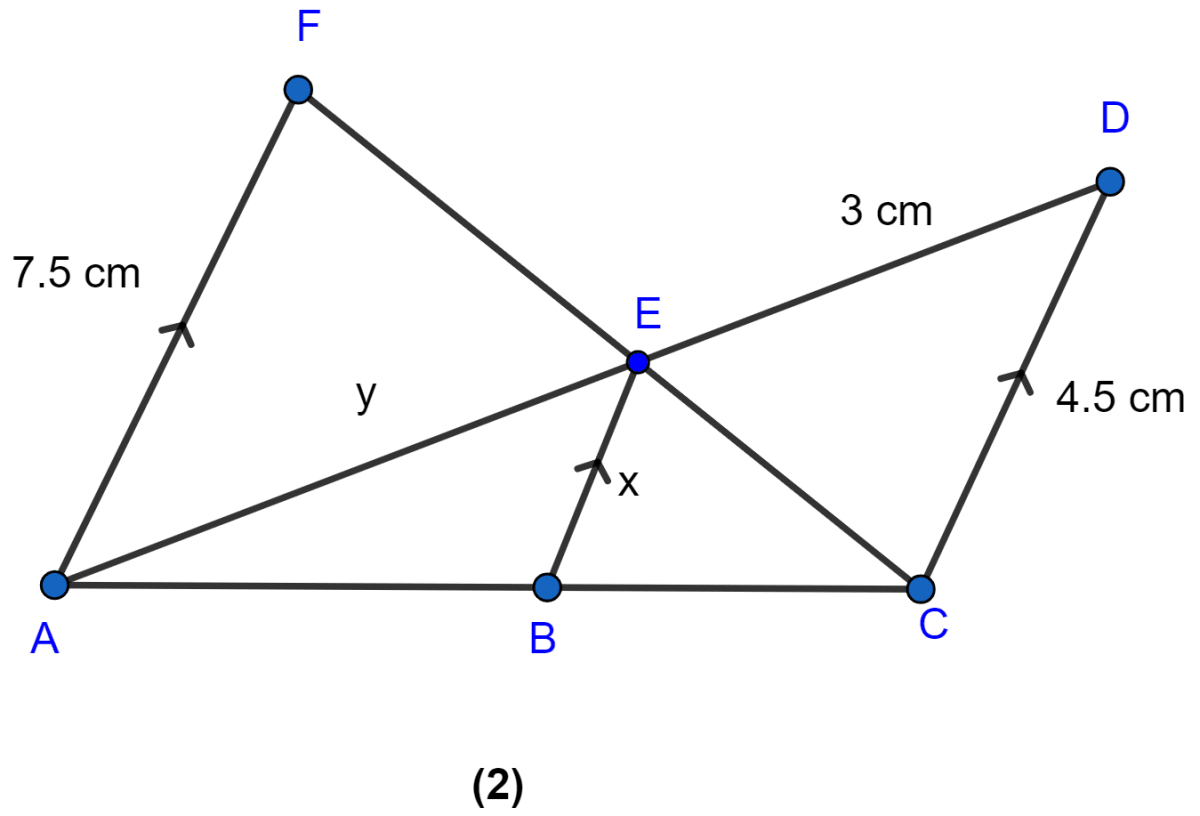
In the figure (2) given below, AF, BE and CD are parallel lines. Given that AF = 7.5 cm, CD = 4.5 cm, ED = 3 cm and BE = x and AE = y. Find the values of x and y.