Mathematics
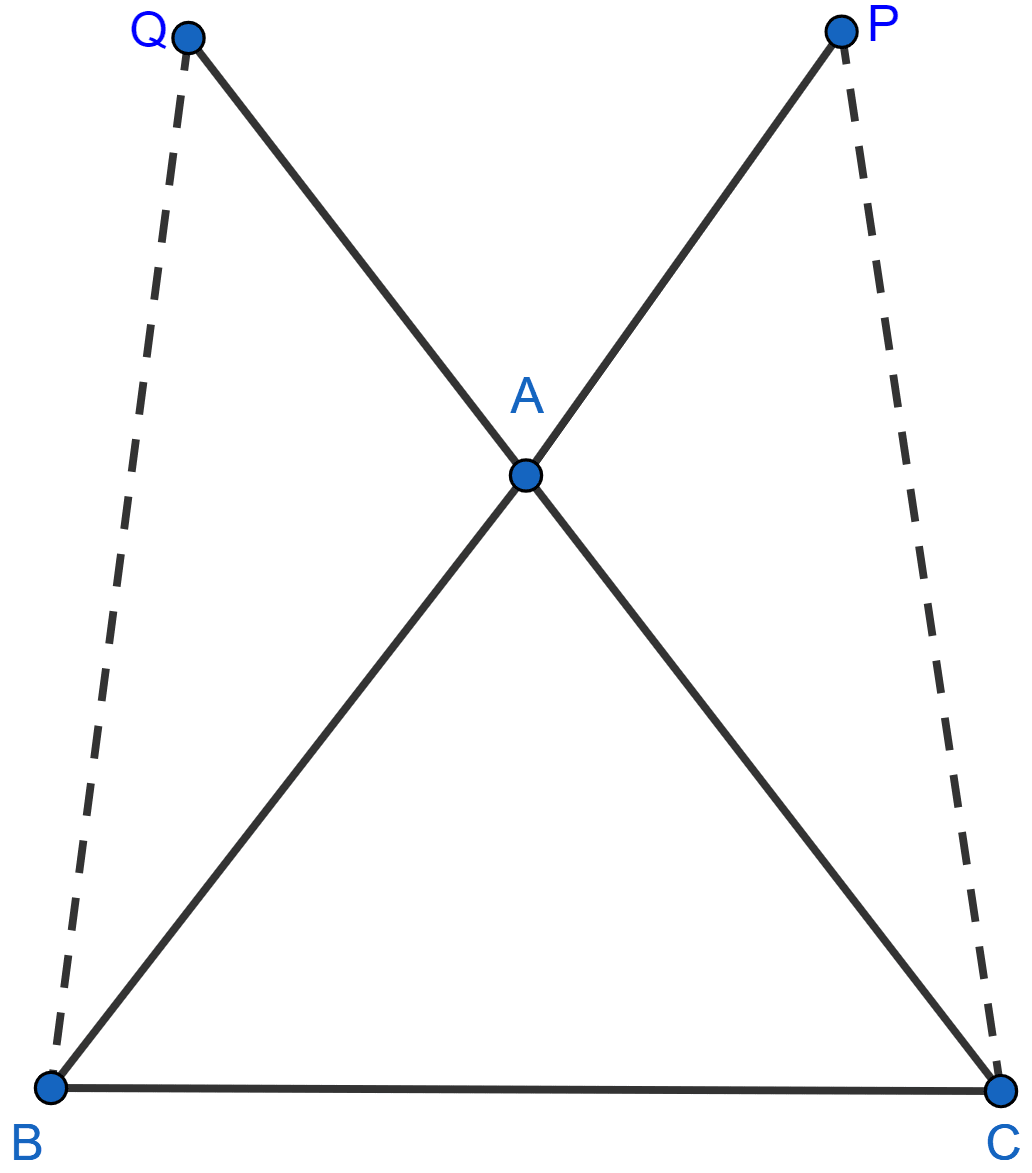
In the adjoining figure, AB = AC, P and Q are points on BA and CA respectively such that AP = AQ. Prove that
(i) △APC ≅ △AQB
(ii) CP = BQ
(iii) ∠ACP = ∠ABQ.
Triangles
83 Likes
Answer
(i) In △APC and △AQB we have,
AP = AQ (Given)
AB = AC (Given)
∠PAC = ∠QAB (Vertically opposite angles)
Hence, by SAS axiom △APC ≅ △AQB.
(ii) As, △APC ≅ △AQB.
We know that corresponding sides of congruent triangles are equal.
∴ CP = BQ.
Hence, proved that CP = BQ.
(iii) As, △APC ≅ △AQB.
We know that corresponding angles of congruent triangles are equal.
∴ ∠ACP = ∠ABQ.
Hence, proved that ∠ACP = ∠ABQ.
Answered By
52 Likes
Related Questions
"If two sides and an angle of one triangle are equal to two sides and an angle of another triangle, then the two triangles must be congruent." Is the statement true? Why?
In the adjoining figure, AB = AC and AP = AQ. Prove that
(i) △APC ≅ △AQB
(ii) CP = BQ
(iii) ∠APC = ∠AQB.
In the adjoining figure, ABCD is a quadrilateral in which AD = BC and ∠DAB = ∠CBA. Prove that
(i) △ABD ≅ △BAC
(ii) BD = AC
(iii) ∠ABD = ∠BAC
In the adjoining figure, AD = BC and BD = AC. Prove that:
∠ADB = ∠BCA and ∠DAB = ∠CBA.
