Mathematics
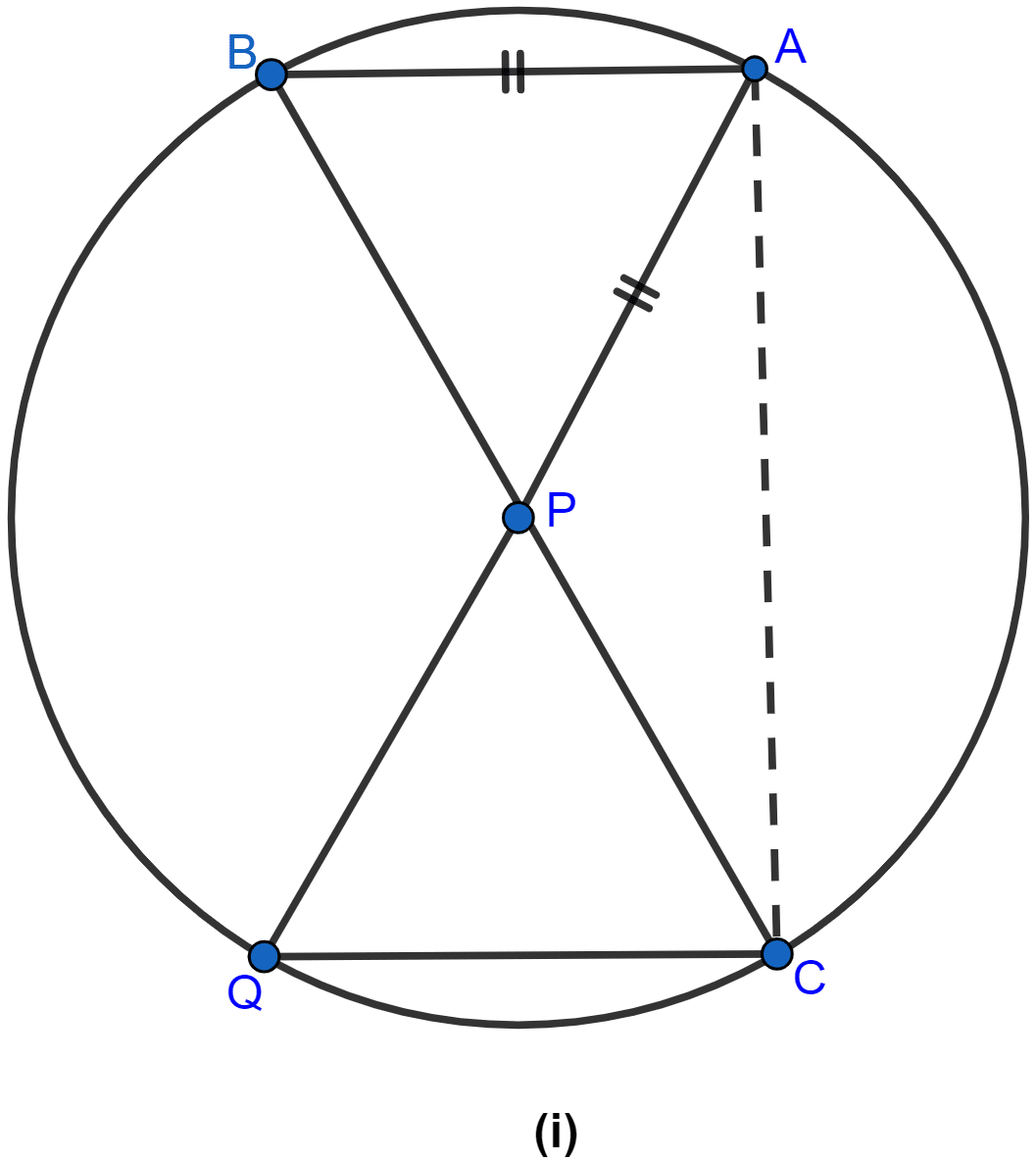
In figure (i) given below, P is the point of intersection of the chords BC and AQ such that AB = AP. Prove that CP = CQ.
Circles
40 Likes
Answer
Given, two chords AQ and BC intersect each other at P inside the circle. AB and CQ are joined and AB = AP.
To prove : CP = CQ
Construction : Join AC.
Proof :
In △ABP and △CQP
∠B = ∠Q (∵ angles in same segment are equal)
∠BAP = ∠PCQ (∵ angles in same segment are equal)
∠BPA = ∠CPQ (∵ vertically opposite angles are equal.)
∴ △ABP ~ △CQP (By AAA axiom of similarity.)
Since, triangles are similar hence, the ratio of the corresponding sides are equal.
We know, AB = AP,
Hence, proved that CQ = CP.
Answered By
27 Likes
Related Questions
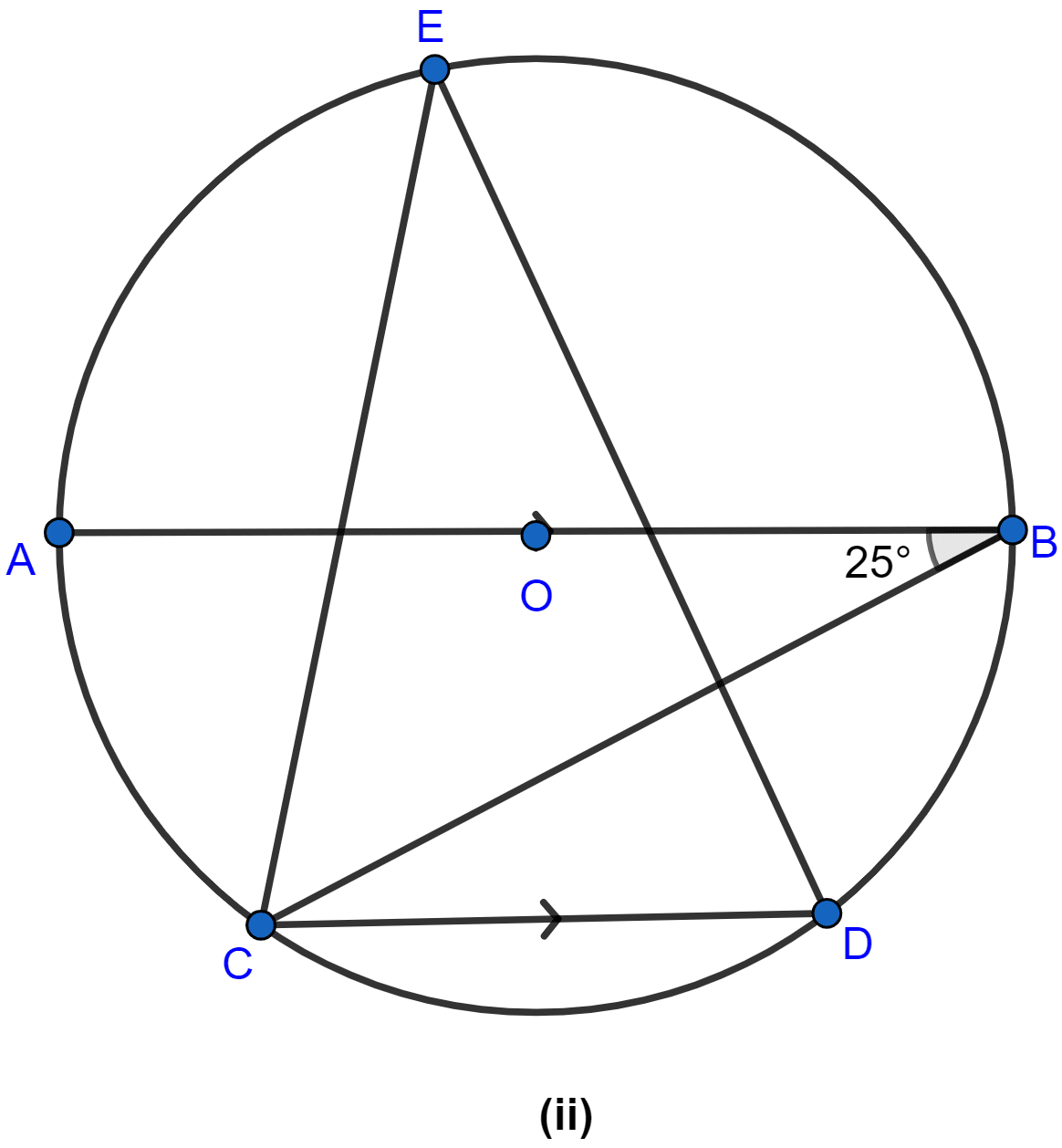
In the figure (ii) given below, O is the centre of a circle. Chord CD is parallel to the diameter AB. If ∠ABC = 25°, calculate ∠CED.
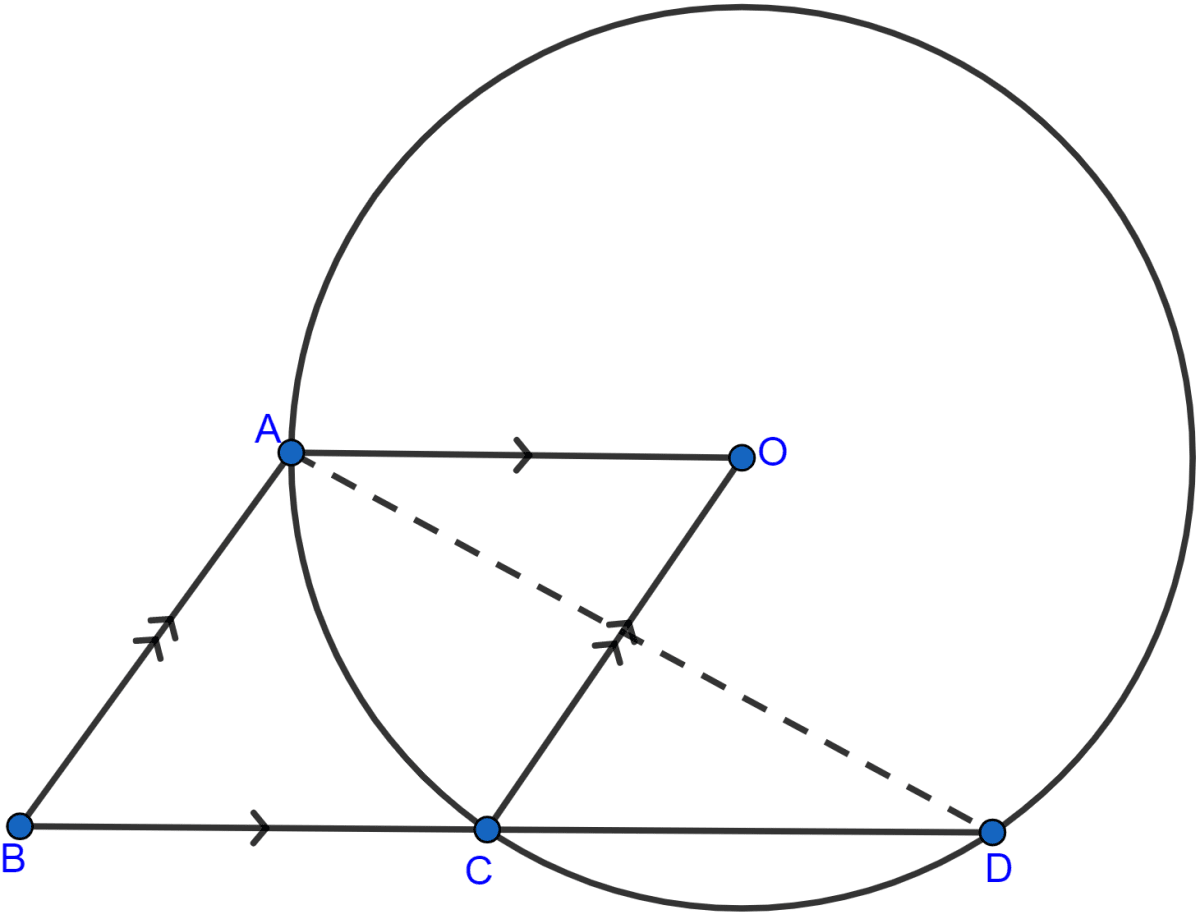
In the adjoining figure, O is the center of the given circle and OABC is a parallelogram. BC is produced to meet the circle at D. Prove that ∠ABC = 2∠OAD.
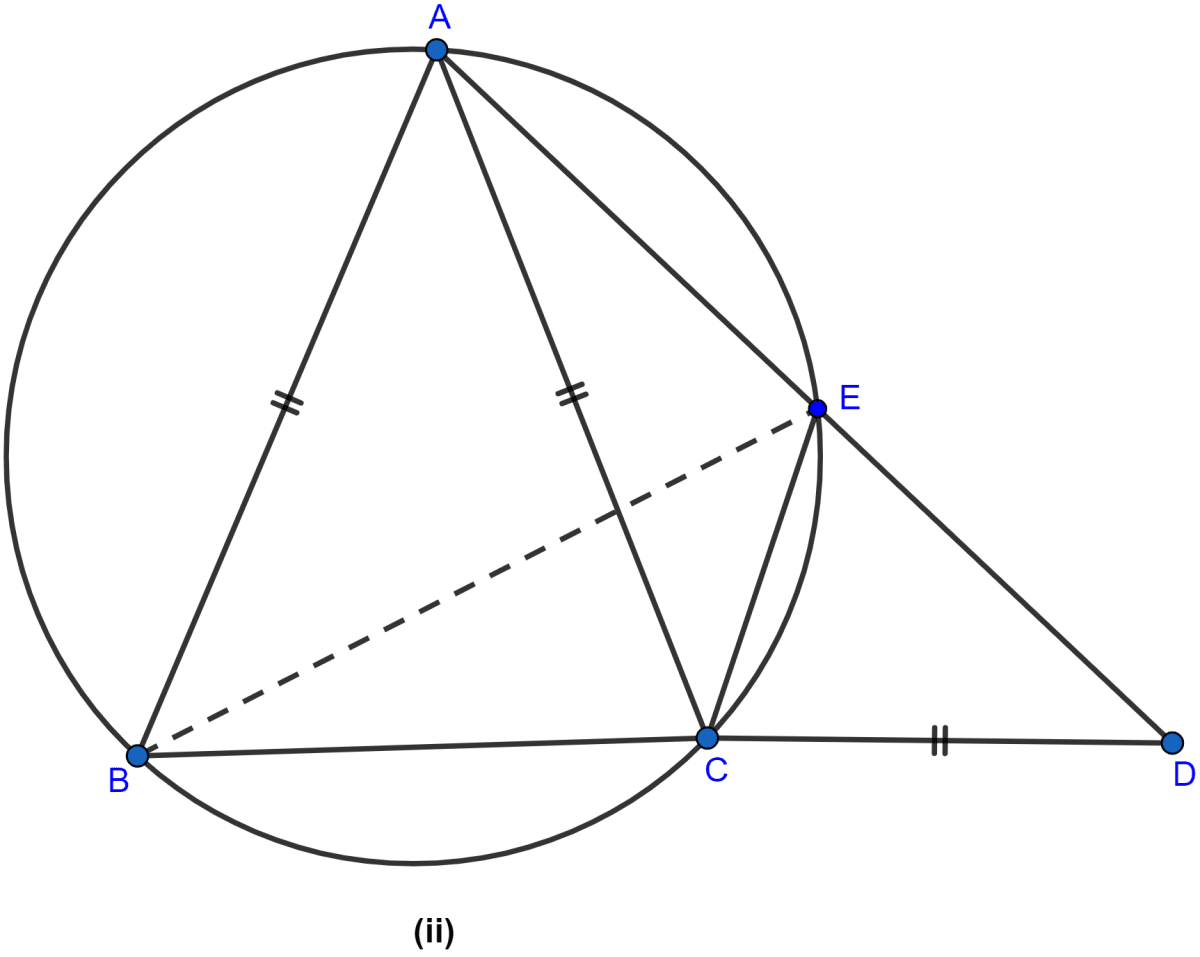
In the figure (ii) given below, AB = AC = CD, ∠ADC = 38°. Calculate
(i) ∠ABC
(ii) ∠BEC.
In the figure (i) given below, CP bisects ∠ACB. Prove that DP bisects ∠ADB.
