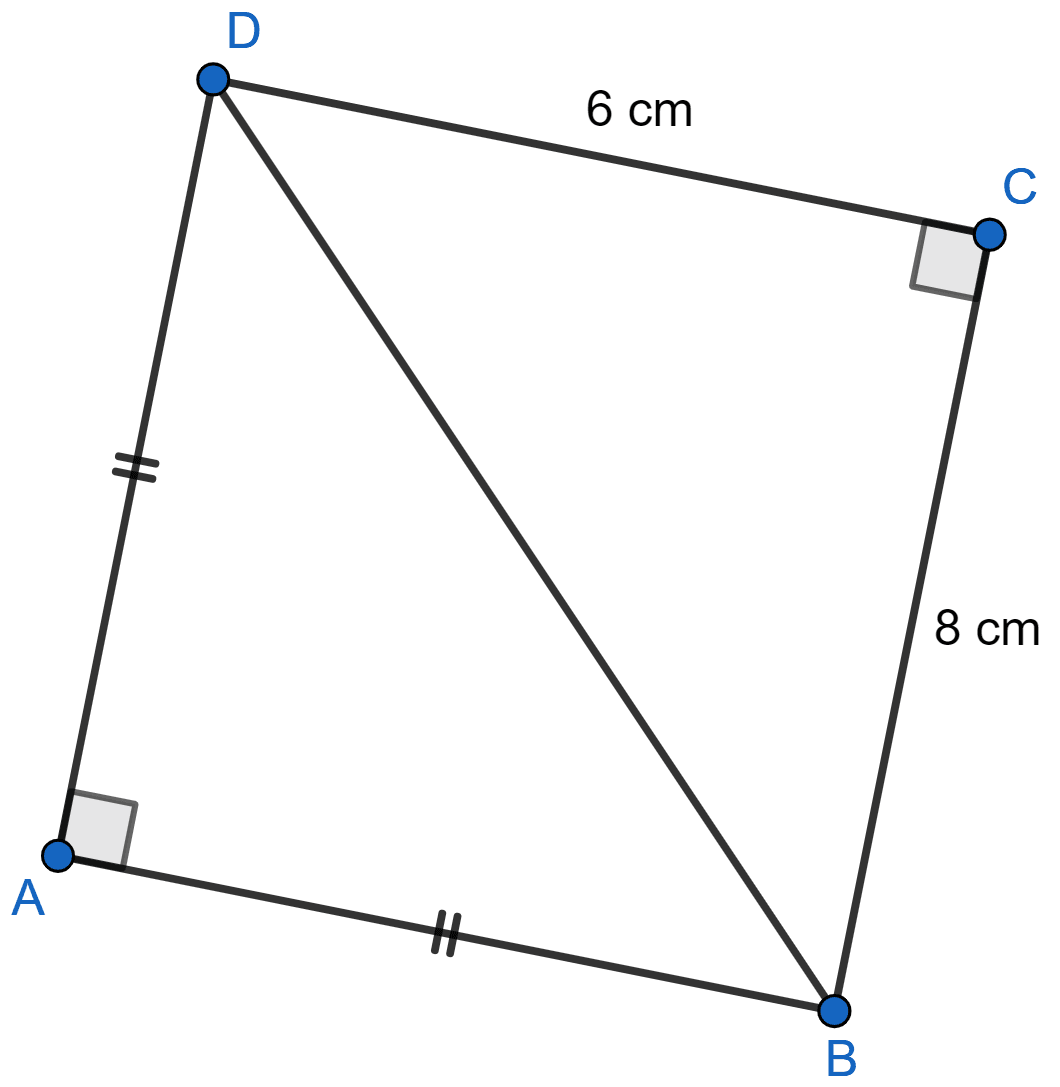
In figure given below, ABCD is a quadrilateral in which AB = AD, ∠A = 90° = ∠C, BC = 8 cm and CD = 6 cm. Find AB and calculate the area of △ABD.

Let AB = AD = x cm.

In right angle △BCD,

By pythagoras theorem,

⇒ BD² = BC² + CD²

⇒ BD² = 8² + 6²

⇒ BD² = 64 + 36

⇒ BD² = 100

⇒ BD = $\sqrt{100}$ = 10 cm.

In right angle △ABD,

By pythagoras theorem,

⇒ BD² = AB² + AD²

⇒ 10² = x² + x²

⇒ 100 = 2x²

⇒ x² = 50

⇒ x = $\sqrt{50} = 5\sqrt{2}$ cm.

Area of right angle △ABD

$= \dfrac{1}{2} \times \text{base} \times \text{ height} \\[1em] = \dfrac{1}{2} \times AB \times AD \\[1em] = \dfrac{1}{2} \times 5\sqrt{2} \times 5\sqrt{2} \\[1em] = 25 \text{ cm}^2.$

Hence, AB = $5\sqrt{2}$ cm and area of right angle △ABD = 25 cm².