Mathematics
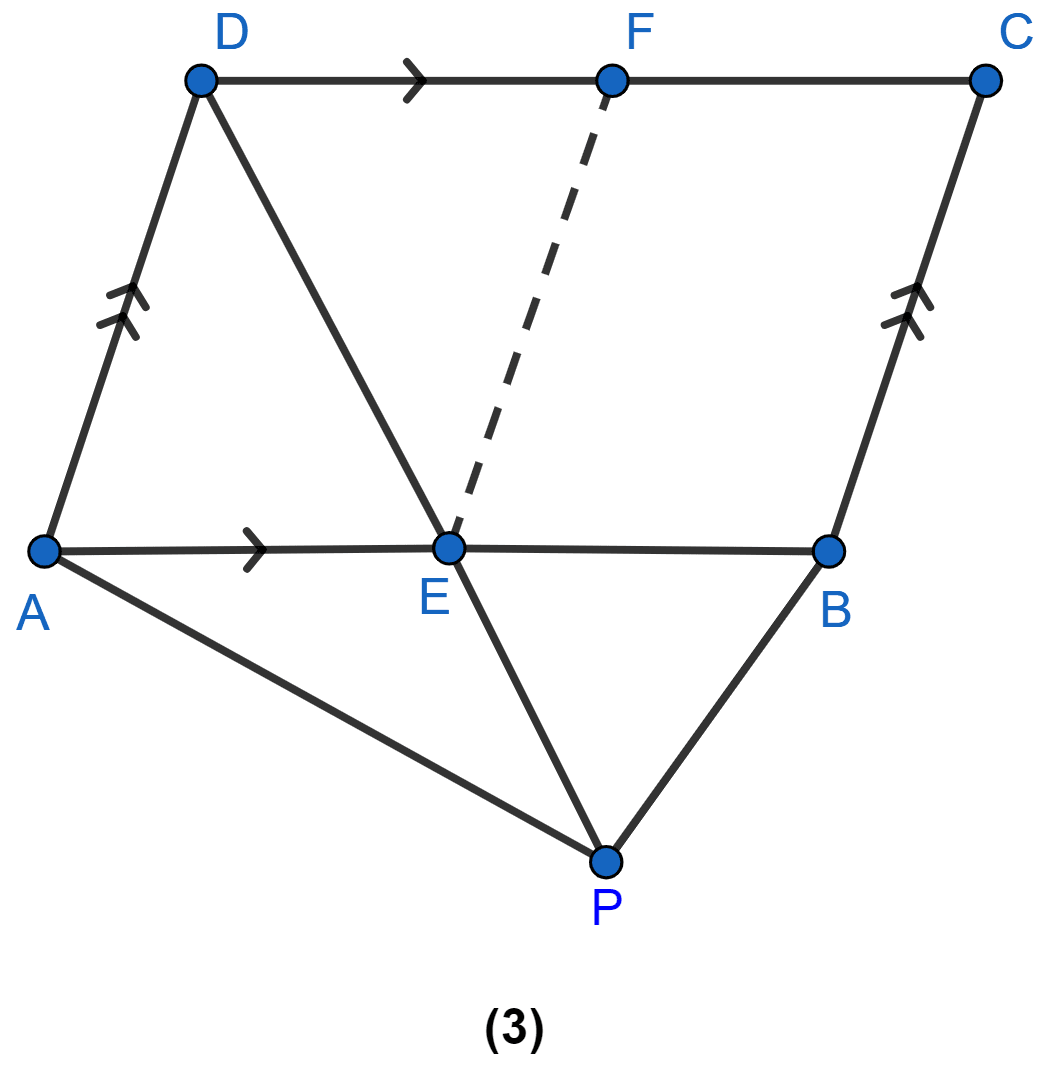
In figure (3) given below, E and F are midpoints of sides AB and CD, respectively, of parallelogram ABCD. If the area of parallelogram ABCD is 36 cm2,
(i) state the area of ∆APD.
(ii) Name the parallelogram whose area is equal to the area of ∆APD.
Theorems on Area
Answer
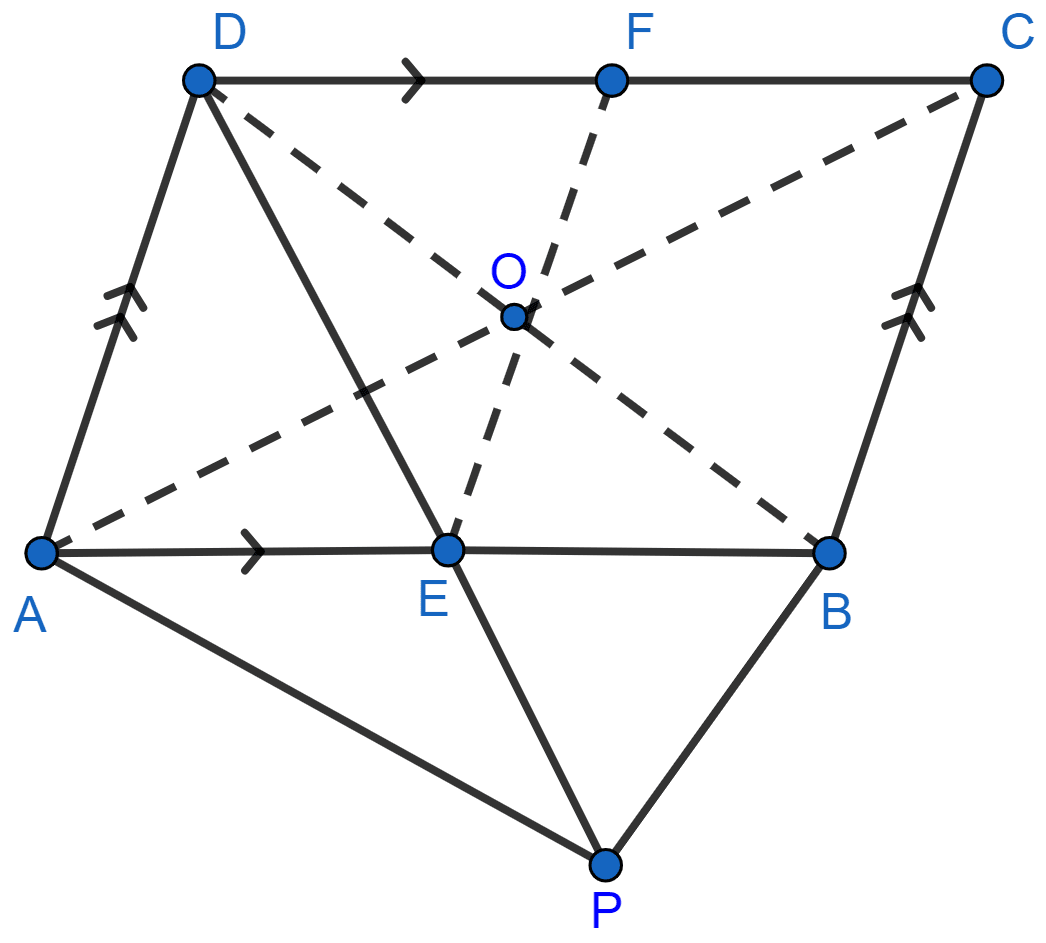
Join the diagonals AC and BD as shown below:
(i) ∆APD and || gm ABCD are on the same base AD and between the same parallel lines AD and BC.
Area of ∆APD = Area of ||gm ABCD
= × 36
= 18 cm2.
Hence, area of ∆APD = 18 cm2.
(ii) Let diagonals AC and BD meet at point O.
In ∆ABC,
Since, O is mid-point of AC (as diagonals bisect each other) and E is mid-point of AB so by mid-point theorem,
EO || BC
∴ EF || BC.
Since, BC || AD so,
⇒ EF || AD.
AB || DC (ABCD is a parallelogram)
⇒ AE || DF
Since, EF || AD and AE || DF.
∴ AEFD is a parallelogram.
EF bisects the parallelogram ABCD in two equal halves as E and F are mid-points of AB and CD and EF || BC || AD.
∴ Area of || gm AEFD = Area of || gm ABCD = × 36 = 18 cm2.
∴ Area of ∆APD = Area of || gm AEFD.
Hence, AEFD is the required parallelogram which has area equal to the area of ∆APD.
Answered By
Related Questions
In figure (1) given below, area of parallelogram ABCD is 29 cm2. Calculate the height of parallelogram ABEF if AB = 5.8 cm.

In figure (2) given below, area of ∆ABD is 24 sq. units. If AB = 8 units, find the height of △ABC.

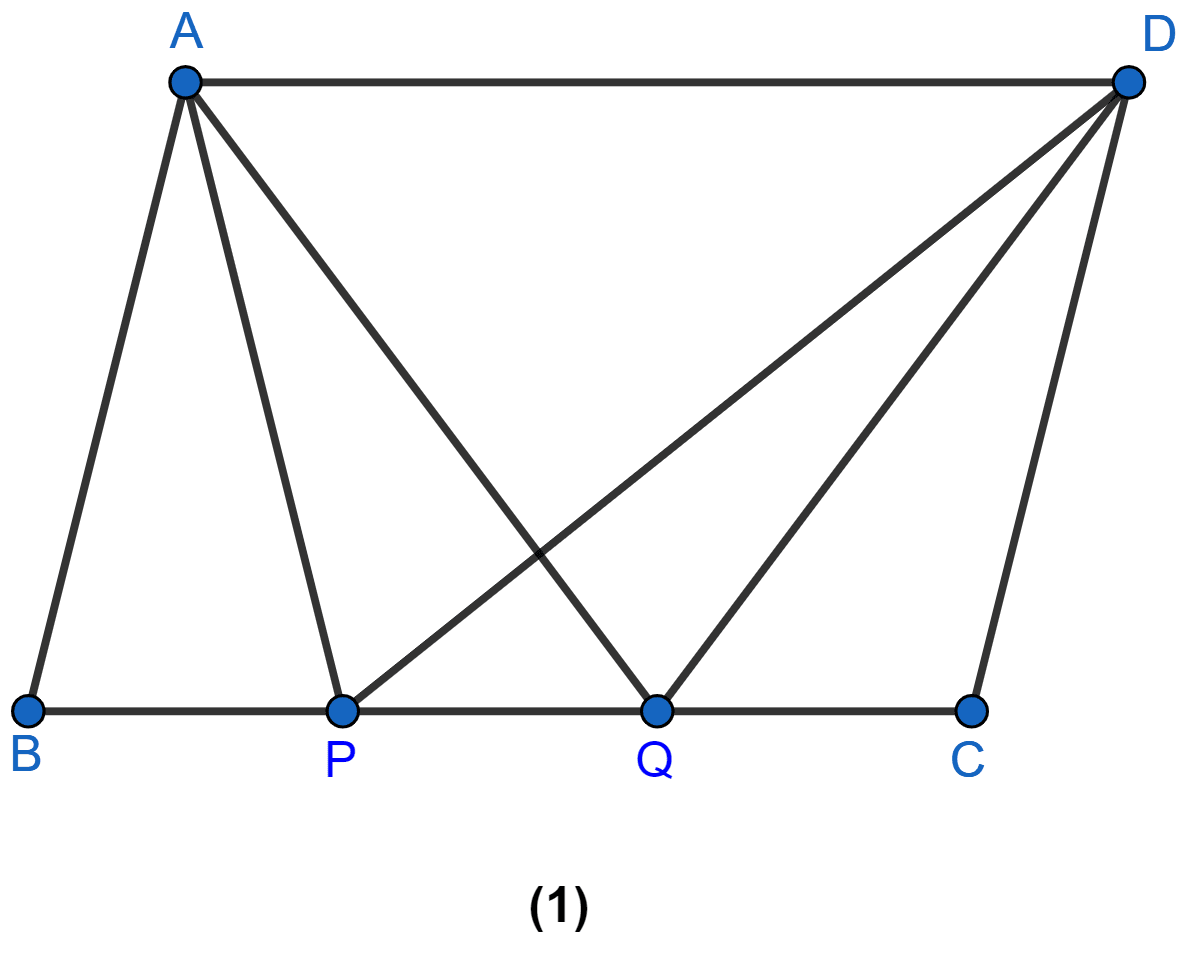
In figure (1) given below, ABCD is a parallelogram. Points P and Q on BC trisect BC into three equal parts. Prove that :
area of ∆APQ = area of ∆DPQ = (area of ||gm ABCD)
In figure (2) given below, DE is drawn parallel to the diagonal AC of the quadrilateral ABCD to meet BC produced at point E. Prove that area of quad. ABCD = area of ∆ABE.
