Mathematics
In ΔABC, ∠ABC = ∠DAC, AB = 8 cm, AC = 4 cm and AD = 5 cm.
(i) Prove that ΔACD is similar to ΔBCA.
(ii) Find BC and CD.
(iii) Find the area of ΔACD : area of ΔABC.
Similarity
8 Likes
Answer
(i) In ∆ACD and ∆BCA,
∠DAC = ∠ABC [Given]
∠ACD = ∠BCA [Common angles]
∴ ∆ACD ~ ∆BCA [By AA]
Hence, proved that ∆ACD ~ ∆BCA.
(ii) Since, ∆ACD ~ ∆BCA
We know that,
Corresponding sides of similar triangle are proportional.
Also,
Hence, BC = 6.4 cm and CD = 2.5 cm.
(iii) As, ∆ACD ~ ∆BCA
We know that,
The areas of two similar triangles are proportional to the squares of their corresponding sides.
Hence, area of ∆ACD : area of ∆ABC = 25 : 64.
Answered By
6 Likes
Related Questions
In the figure given below, AB ‖ EF ‖ CD. If AB = 22.5 cm, EP = 7.5 cm, PC = 15 cm and DC = 27 cm.
Calculate: (i) EF (ii) AC

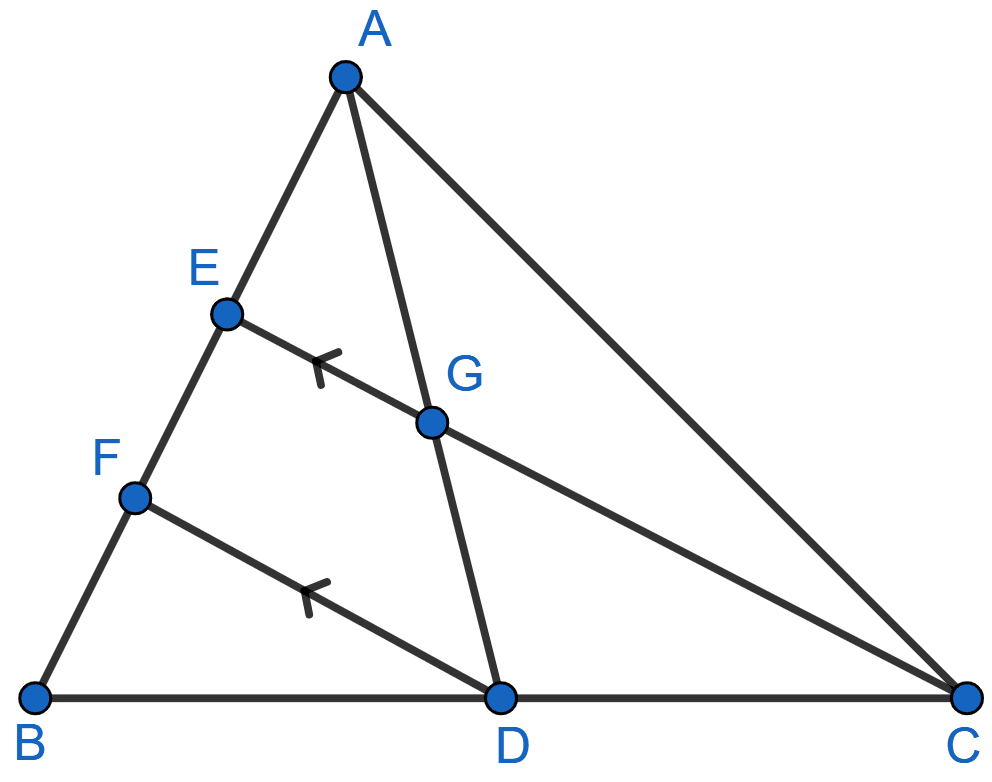
In the following figure, AD and CE are medians of ∆ABC. DF is drawn parallel to CE. Prove that:
(i) EF = FB,
(ii) AG : GD = 2 : 1
In the following figure, DE || AC and DC || AP. Prove that : .

The two similar triangles are equal in area. Prove that the triangles are congruent.