Mathematics
In a triangle ABC, AB = AC, D and E are points on the sides AB and AC respectively such that BD = CE. Show that:
(i) △DBC ≅ △ECB
(ii) ∠DCB = ∠EBC
(iii) OB = OC, where O is the point of intersection of BE and CD.
Answer
(i) From figure,
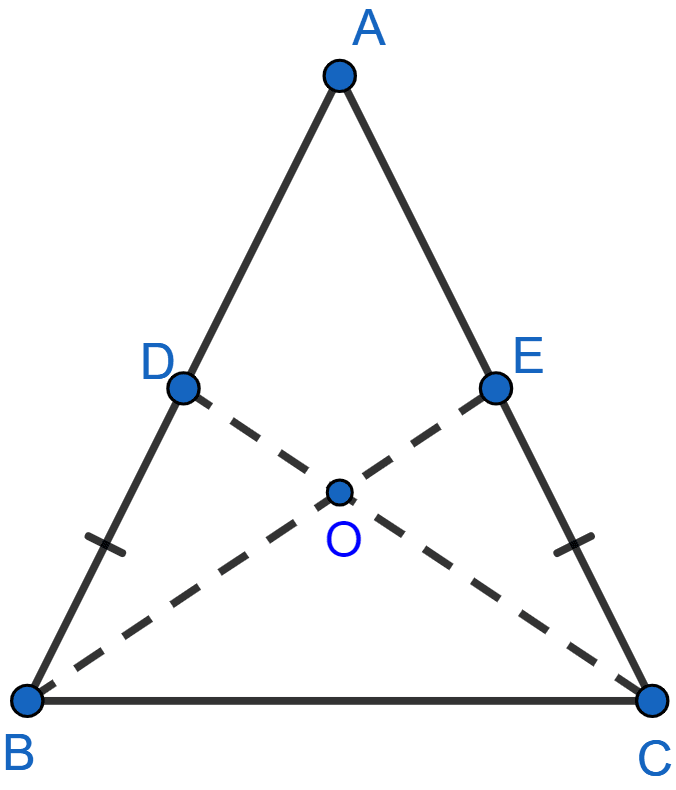
BD = CE (Given)
BC = BC (Common)
∠DBC = ∠ECB (as AB = AC).
∴ △DBC ≅ △ECB by SAS axiom.
Hence, proved △DBC ≅ △ECB.
(ii) We know that corresponding parts of congruent triangle are equal.
∴ ∠DCB = ∠EBC.
Hence, proved that ∠DCB = ∠EBC.
(iii) As △DBC ≅ △ECB,
∠BDO = ∠CEO (By c.p.c.t.)
∠DOB = ∠EOC (Vertically opposite angles)
BD = CE (Given)
Hence, △BOD ≅ △EOC by ASA axiom.
We know that corresponding parts of congruent triangles are equal.
∴ OB = OC.
Hence, proved that OB = OC.
Related Questions
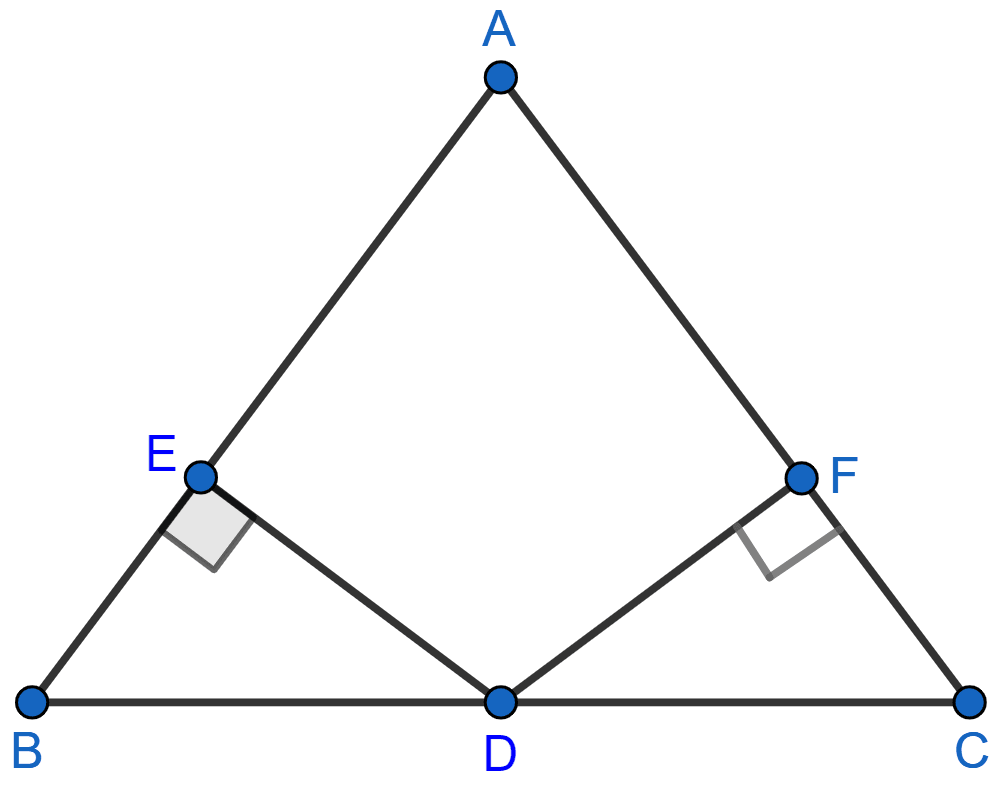
In the adjoining figure, D is the midpoint of BC, DE and DF are perpendiculars to AB and AC respectively such that DE = DF. Prove that ABC is an isosceles triangle.
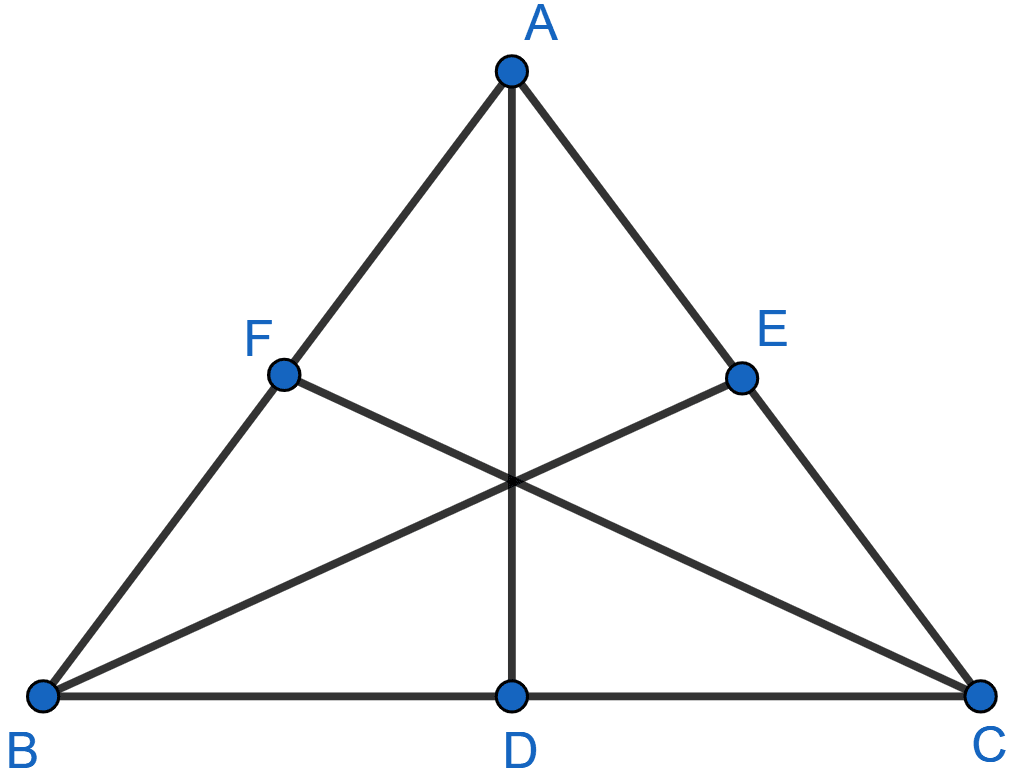
In the adjoining figure, D and E are points on the side BC of △ABC such that BD = EC and AD = AE. Show that △ABD ≅ △ACE.
ABC is an isosceles triangle in which AB = AC. P is any point in the interior of △ABC such that ∠ABP = ∠ACP. Prove that
(a) BP = CP
(b) AP bisects ∠BAC.
In the adjoining figure, AD, BE and CF are altitudes of △ABC. If AD = BE = CF, prove that ABC is an equilateral triangle.
