In a trapezium ABCD, side AB is parallel to side DC; and the diagonals AC and BD intersect each other at point P. Prove that:

(i) ΔAPB is similar to ΔCPD.

(ii) PA x PD = PB x PC.

Trapezium ABCD is shown in the figure below:

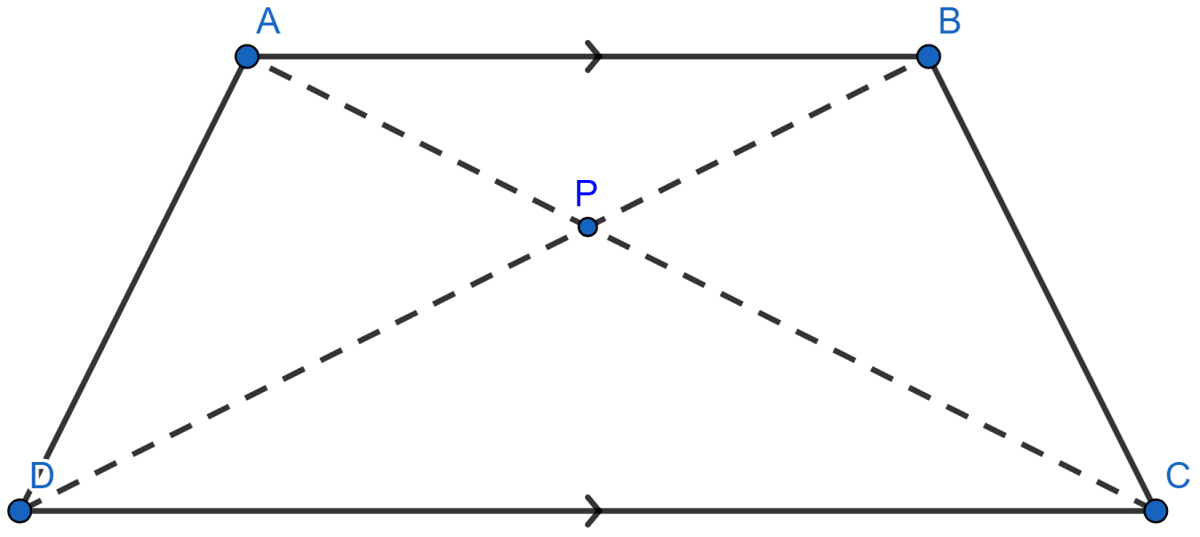
(i) In ∆APB and ∆CPD, we have

∠APB = ∠CPD [Vertically opposite angles]

∠ABP = ∠CDP [Alternate angles (as AB||DC) are equal]

∴ ∆APB ~ ∆CPD [By A.A.]

Hence, proved that ∆APB ~ ∆CPD.

(ii) We know that,

In similar triangles the ratio of corresponding sides are equal.

$\therefore \dfrac{\text{PA}}{\text{PC}} = \dfrac{\text{PB}}{\text{PD}} \\[1em] \Rightarrow \text{PA} \times \text{PD} = \text{PB} \times \text{PC}.$

Hence, proved that PA x PD = PB x PC.