Mathematics
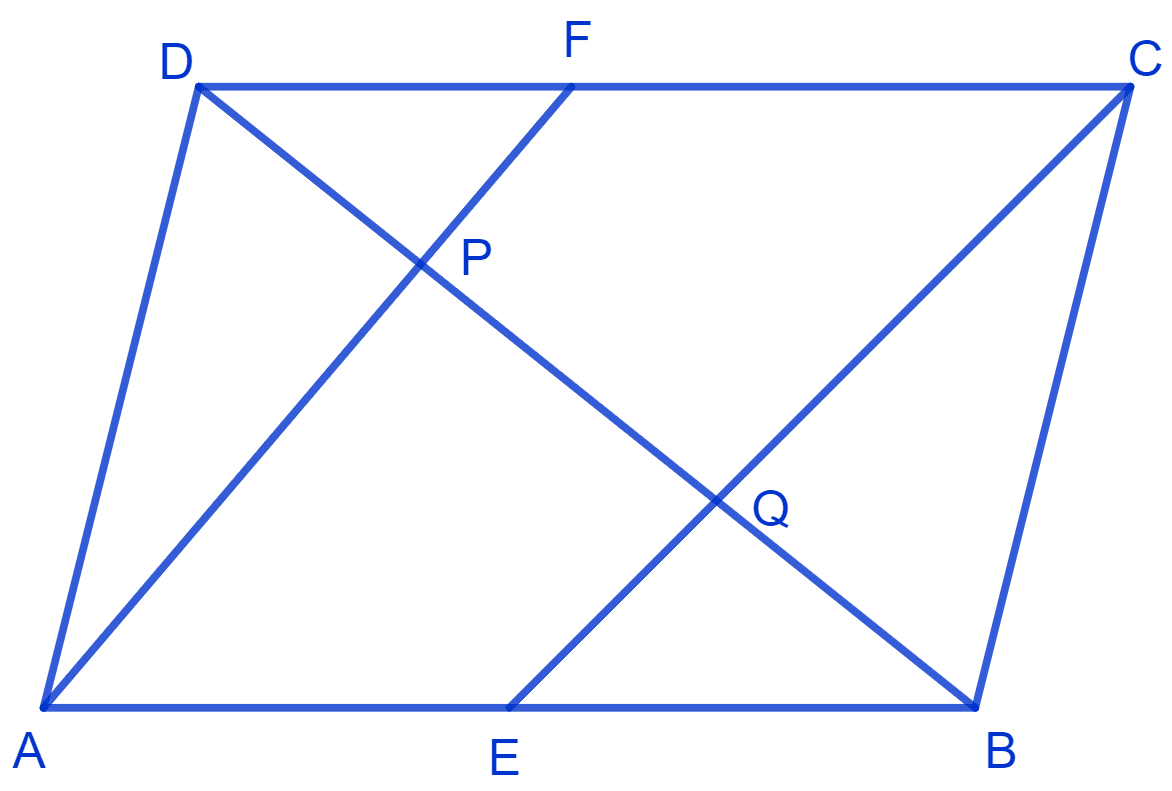
In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively see Fig. Show that the line segments AF and EC trisect the diagonal BD.
Rectilinear Figures
2 Likes
Answer
Given :
ABCD is a parallelogram, where E and F are the mid-points of sides AB and CD.
We know that,
Opposite sides of a parallelogram are equal and parallel.
∴ AB || CD and AB = CD
∴ AE || FC
Since, AB = CD
Dividing both sides of equation by 2, we get :
⇒ =
⇒ AE = FC
∴ AEFC is a parallelogram.
∴ AF || CE
From figure,
⇒ PF || QC
⇒ EQ || AP
In △ DQC,
F is the mid-point of DC and FP || CQ.
By converse of mid-point theorem, we get :
P is the mid-point of DQ.
⇒ DP = PQ …..(1)
In △ APB,
E is the mid-point of AB and EQ || AP.
By converse of mid-point theorem, we get :
Q is the mid-point of BP.
⇒ PQ = QB ……(2)
From equations (1) and (2) we get :
⇒ PQ = QB = DP.
∴ AF and EC trisect BD.
Hence, proved that AF and EC trisect the diagonal BD.
Answered By
3 Likes
Related Questions
ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F see Fig. Show that F is the mid-point of BC.
ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that
(i) D is the mid-point of AC
(ii) MD ⊥ AC
(iii) CM = MA =