Mathematics
If the area of the semi-circular region is 77 cm2, find its perimeter.
Mensuration
20 Likes
Answer
Let radius = r cm.
Given,
Area of semi-circular region = 77 cm2
Perimeter of semi-circle = (π + 2) = πr + 2r
=
= 22 + 14
= 36 cm.
Hence, perimeter of circle = 36 cm.
Answered By
12 Likes
Related Questions
Find the radius and circumference of a circle whose area is 144π cm2.
A sheet is 11 cm long and 2 cm wide. Circular pieces 0.5 cm in diameter are cut from it to prepare discs. Calculate the number of discs that can be prepared.
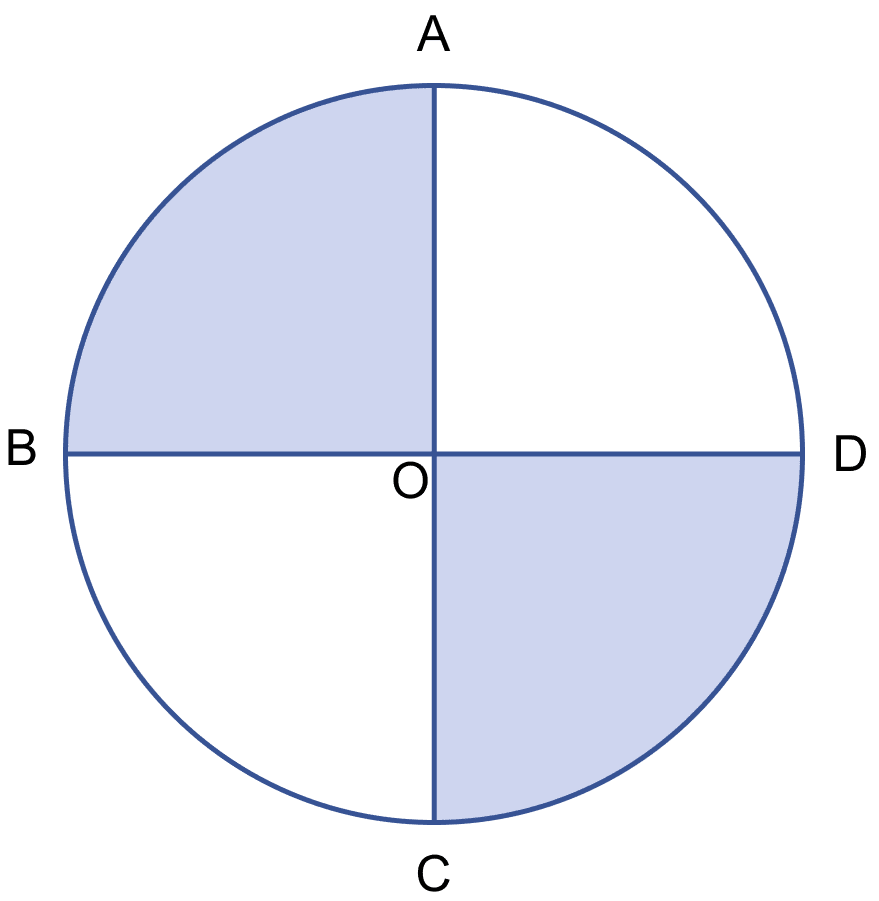
In the figure (i) given below, AC and BD are two perpendicular diameters of a circle ABCD. Given that the area of shaded portion is 308 cm2, calculate :
(i) the length of AC and
(ii) the circumference of the circle.

In the figure (ii) given below, AC and BD are two perpendicular diameters of a circle with center O. If AC = 16 cm, calculate the area and perimeter of the shaded part. (Take π = 3.14)