Mathematics
If circles are drawn taking two sides of a triangle as diameters, prove that the point of intersection of these circles lie on the third side.
Answer
Two circles are drawn taking sides AB and AC as diameter.
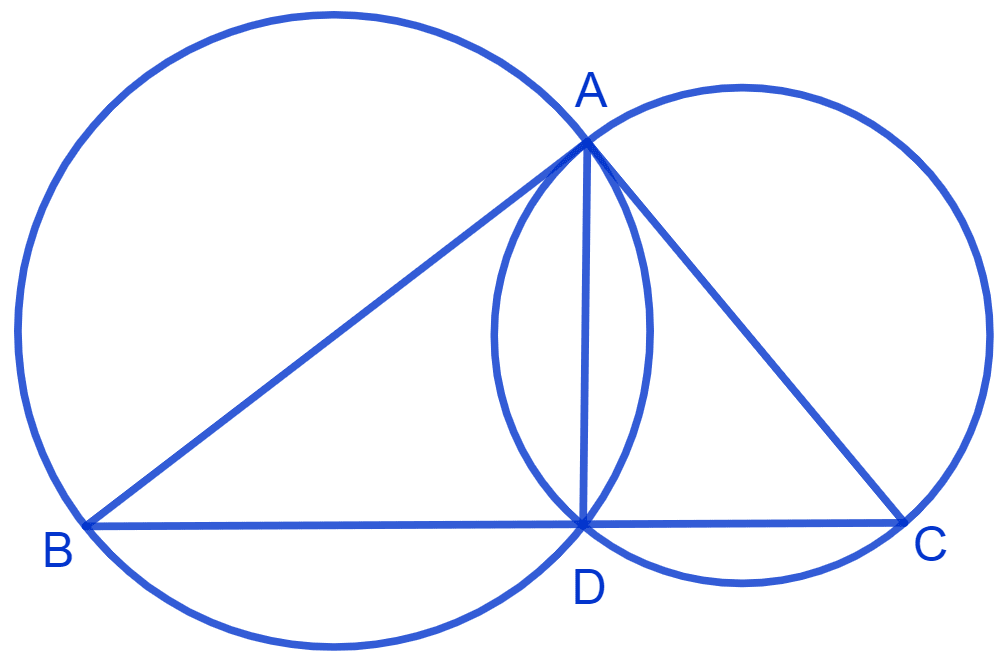
⇒ ∠ADB = ∠ADC = 90° (Angles in the semi circle is a right angle.)
⇒ ∠ADB + ∠ADC = 90° + 90°
⇒ ∠ADB + ∠ADC = 180°
∴ BDC is straight line.
Thus, D lies on BC.
Hence, proved that the point of intersection of circles lie on the third side.
Related Questions
ABC and ADC are two right triangles with common hypotenuse AC. Prove that ∠CAD = ∠CBD.
If the non-parallel sides of a trapezium are equal, prove that it is cyclic.
Prove that a cyclic parallelogram is a rectangle.
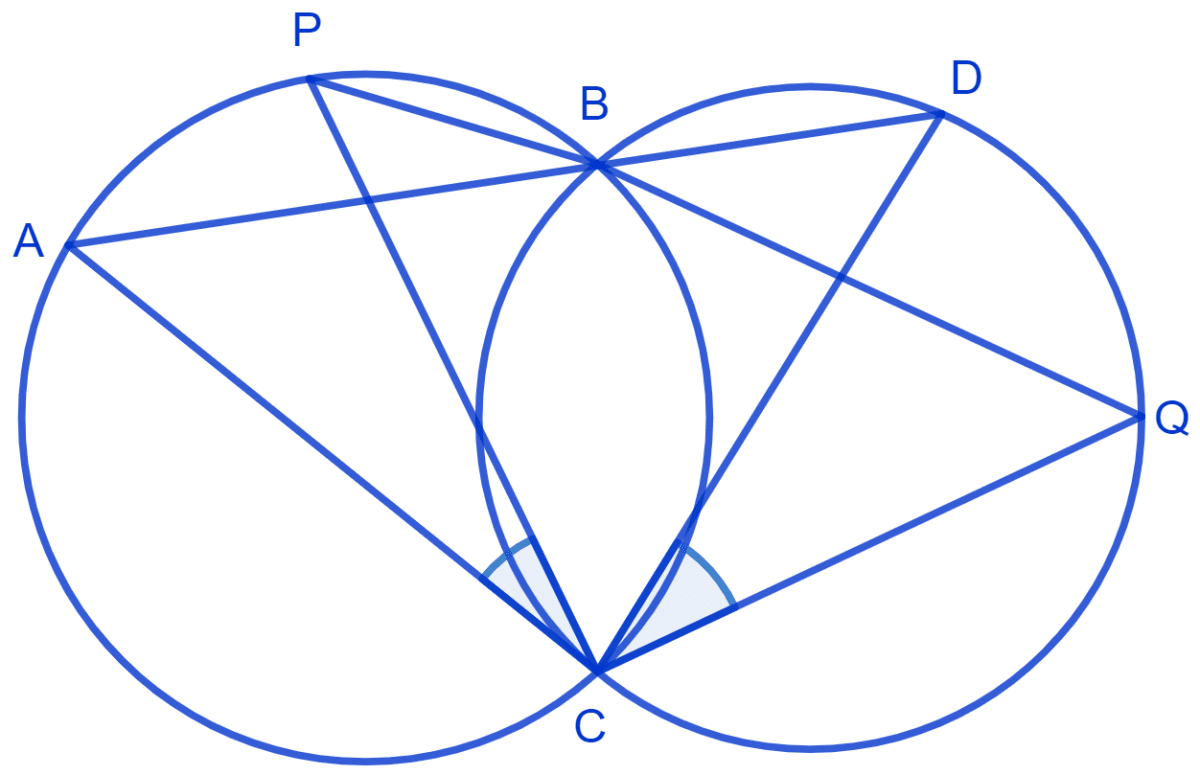
Two circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D and P, Q respectively. Prove that ∠ACP = ∠QCD.
