Physics
How does the time period (T) of a simple pendulum depend on its length (l) ? Draw a graph showing the variation of T2 with l. How will you use this graph to determine the value of g (acceleration due to gravity)?
Measurements
79 Likes
Answer
Time period of a simple pendulum is directly proportional to the square root of its effective length.
i.e., T ∝
Graph showing the variation of T2 with l is given below:
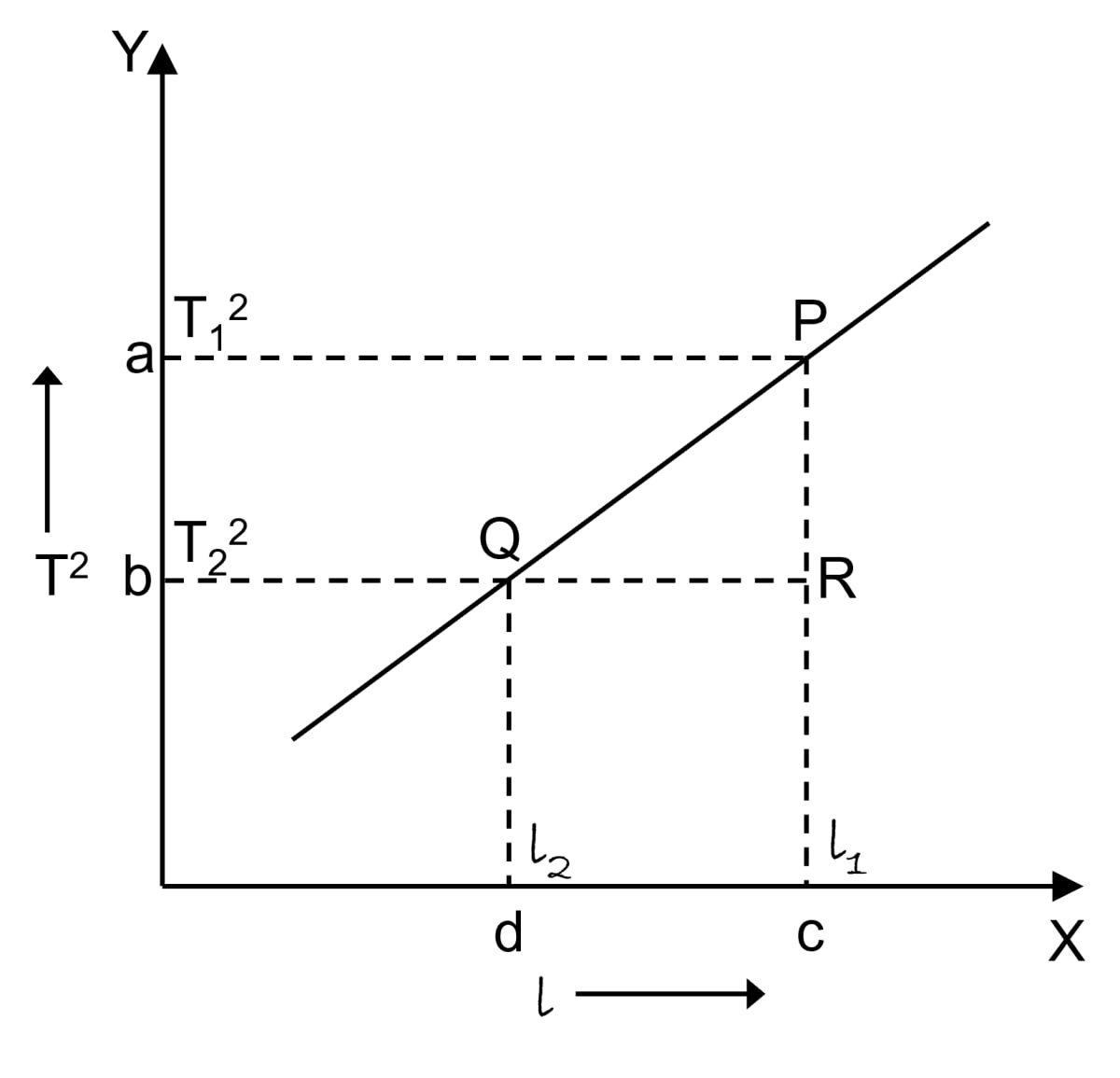
In order to find the acceleration due to gravity with the help of the above graph, we follow the following steps —
The slope of the straight line obtained in the T2 vs l graph, as shown in fig, can be obtained by taking two points P and Q on the straight line and drawing normals from these points on the X and Y axes. Then, note the value of T2, say T12 and T22 at a and b respectively, and also the value of l say l1 and l2 respectively at c and d.
Then,
This slope is found to be a constant at a place and,
where, g = acceleration due to gravity at that place.
Thus, g can be determined at a place from the graph using the following relation,
Answered By
44 Likes
Related Questions
Two simple pendulums A and B have lengths 1.0 m and 4.0 m respectively at a certain place. Which pendulum will make more oscillations in 1 minute? Explain your answer.
State how does the time period of a simple pendulum depend on —
(a) length of pendulum,
(b) mass of bob,
(c) amplitude of oscillation and
(d) acceleration due to gravity.
A simple pendulum completes 40 oscillations in one minute.
Find its —
(a) frequency,
(b) time period.
The time period of a simple pendulum is 2s. What is its frequency? What name is given to such a pendulum?