Mathematics
From a tower 126 m high, the angles of depression of two rocks which are in a horizontal line through the base of the tower are 16° and 12° 20'. Find the distance between the rocks if they are on
(i) the same side of the tower
(ii) the opposite sides of the tower.
Heights & Distances
80 Likes
Answer
(i) Let the rocks be at point A and D.
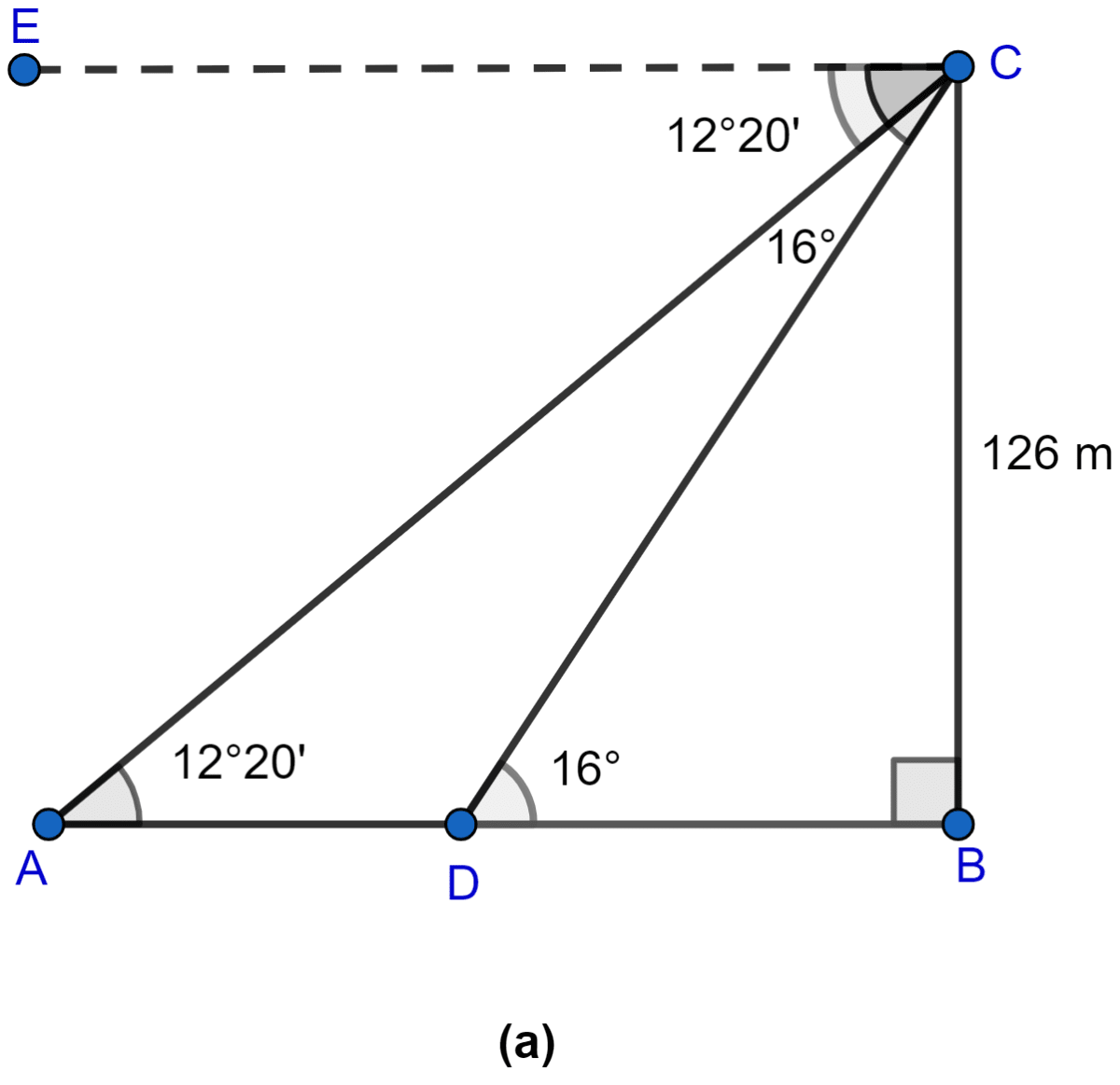
From figure a,
∠CAB = ∠ECA = 12° 20' (Alternate angles are equal)
∠CDB = ∠ECD = 16° (Alternate angles are equal)
Considering right angled △ABC, we get
Considering right angled △BCD, we get
Distance between two rocks (AD) = AB - BD = 576.29 - 439.48 = 136.81
Hence, the distance between two rocks when they are on same side of the tower is 136.81 meters.
(ii) Let the rocks be at point A and B.
From figure b,
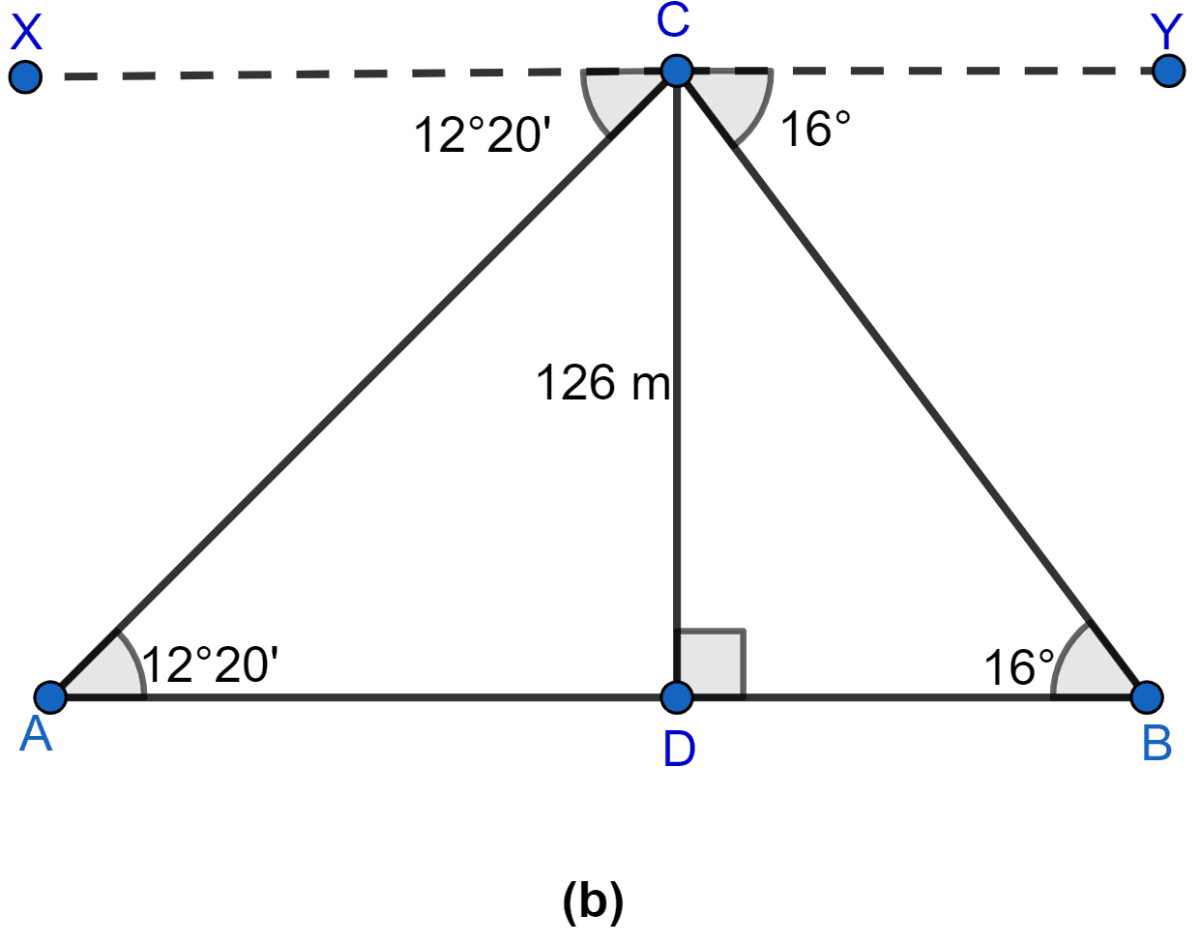
∠CAD = ∠XCA = 12° 20' (Alternate angles are equal)
∠CBD = ∠YCB = 16° (Alternate angles are equal)
Considering right angled △ADC, we get
Considering right angled △BCD, we get
Distance between two rocks (AB) = AD + DB = 576.29 + 439.48 = 1015.7
Hence, the distance between two rocks when they are on opposite sides of the tower is 1015.7 meters.
Answered By
21 Likes
Related Questions
A man 1.8 m high stands at a distance of 3.6 m from a lamp post and casts a shadow of 5.4 m on the ground. Find the height of the lamp post.
An aeroplane at an altitude of 250 m observes the angle of depression of two boats on the opposite banks of a river to be 45° and 60° respectively. Find the width of the river. Write the answer correct to the nearest whole number.
The angles of depression of the top and the bottom of a 8 m tall building from the top of a multi-storeyed building are 30° and 45° respectively. Find the height of the multi storeyed building and the distance between the two buildings, correct to two decimal places.
The angles of depression of two ships A and B as observed from the top of a light house 60 m high are 60° and 45° respectively. If the two ships are on the opposite sides of the light house, find the distance between the two ships. Give your answer correct to the nearest whole number.