Mathematics
The angles of depression of two ships A and B as observed from the top of a light house 60 m high are 60° and 45° respectively. If the two ships are on the opposite sides of the light house, find the distance between the two ships. Give your answer correct to the nearest whole number.
Heights & Distances
38 Likes
Answer
Let CD be the light house, 60 m tall and ships at point A and B.
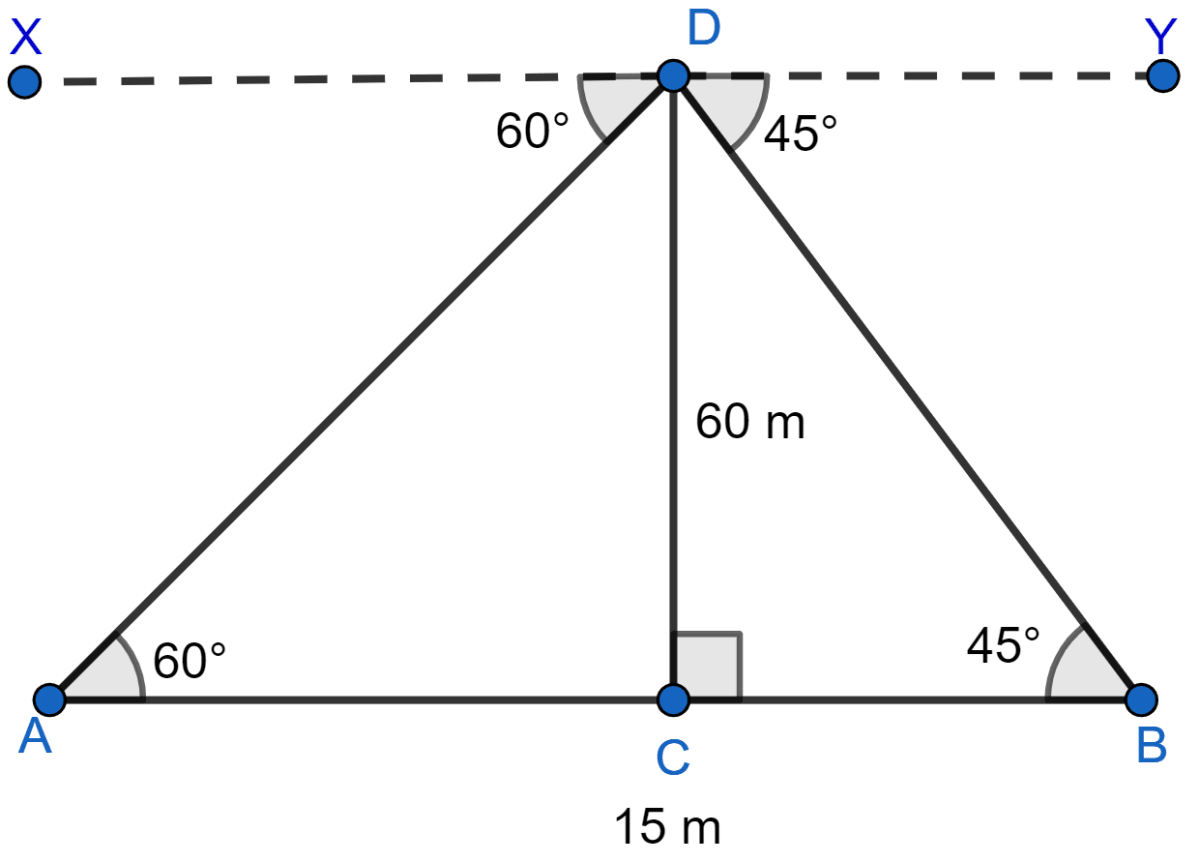
From figure,
∠DAC = ∠XDA = 60° (Alternate angles are equal)
∠DBC = ∠YDB = 45° (Alternate angles are equal)
Considering right angled △ACD, we get
Considering right angled △BCD, we get
Distance between two ships (AB) = AC + BC = 34.64 + 60 = 94.64 meters.
Rounding off to nearest meter, AB = 95 meters.
Hence, the distance between two ships is 95 meters.
Answered By
18 Likes
Related Questions
The angle of elevation of a pillar from a point A on the ground is 45° and from a point B diametrically opposite to A and on the other side of the pillar is 60°. Find the height of the pillar, given that the distance between A and B is 15 m.
From two points A and B on the same side of a building, the angles of elevation of the top of the building are 30° and 60° respectively. If the height of the building is 10 m, find the distance between A and B correct to two decimal places.
An aeroplane at an altitude of 250 m observes the angle of depression of two boats on the opposite banks of a river to be 45° and 60° respectively. Find the width of the river. Write the answer correct to the nearest whole number.
From a tower 126 m high, the angles of depression of two rocks which are in a horizontal line through the base of the tower are 16° and 12° 20'. Find the distance between the rocks if they are on
(i) the same side of the tower
(ii) the opposite sides of the tower.