From a cube of edge 14 cm, a cone of maximum size is carved out. Find the volume of the remaining material.

Edge of a cube = 14 cm.

Volume = (side)³ = (14)³ = 2744 cm³.

Cone of maximum size is carved out as shown in figure,

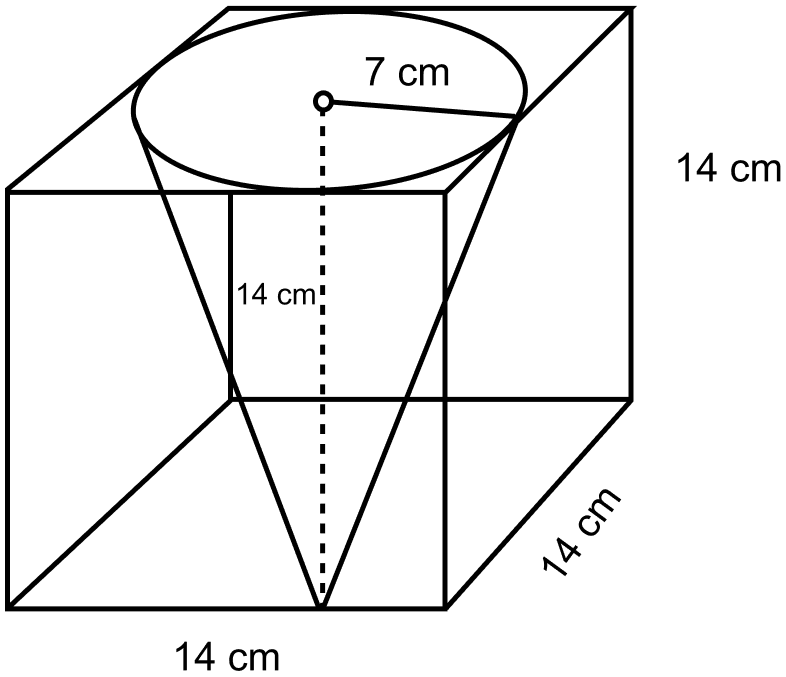
Diameter of the cone cut out from it = 14 cm.

Radius = $\dfrac{\text{Diameter}}{2}$

= $\dfrac{\text{14}}{2}$ = 7 cm.

Volume of cone = $\dfrac{1}{3}πr^2h$

Height = 14 cm.

Putting values we get,

$\text{Volume of cone } = \dfrac{1}{3} \times \dfrac{22}{7} \times 7^2 \times 14 \\[1em] = \dfrac{22 \times 49 \times 14}{3 \times 7} \\[1em] = \dfrac{15092}{21} \\[1em] = \dfrac{2156}{3} \text{ cm}^3.$

Volume of the remaining material = Volume of the cube - Volume of the cone.

Volume of remaining material = $2744 - \dfrac{2156}{3}$

$= \dfrac{(3 \times 2744) - 2156}{3} \\[1em] = \dfrac{8232 - 2156}{3} \\[1em] = \dfrac{6076}{3} \\[1em] = 2025\dfrac{1}{3} \text{ cm}^3.$

Hence, the volume of the remaining material is $2025\dfrac{1}{3} \text{ cm}^3$.