Find graphically the coordinates of the vertices of the triangle formed by the lines y = 0, y = x and 2x + 3y = 10. Hence, find the area of the triangle formed by these lines.

Given,

1st equation :

y = 0,

2nd equation :

y = x,

When, x = 0, y = 0,

x = 1, y = 1,

x = 2, y = 2.

Table of values of equation (2) :

Steps of construction :

1. Plot the points (0, 0), (1, 1) and (2, 2).

2. Join the points.

3rd equation :

⇒ 2x + 3y = 10

⇒ 3y = 10 - 2x

⇒ y = $\dfrac{10 - 2x}{3}$ ……….(3)

When, x = -1, y = $\dfrac{10 - 2 \times (-1)}{3} = \dfrac{10 + 2}{3} = \dfrac{12}{3}$ = 4,

x = 2, y = $\dfrac{10 - 2 \times 2}{3} = \dfrac{10 - 4}{3} = \dfrac{6}{3}$ = 2,

x = 5, y = $\dfrac{10 - 2 \times 5}{3} = \dfrac{10 - 10}{3}$ = 0.

Table of values of equation (3) :

Steps of construction :

1. Plot the points (-1, 4), (2, 2) and (5, 0).

2. Join the points.

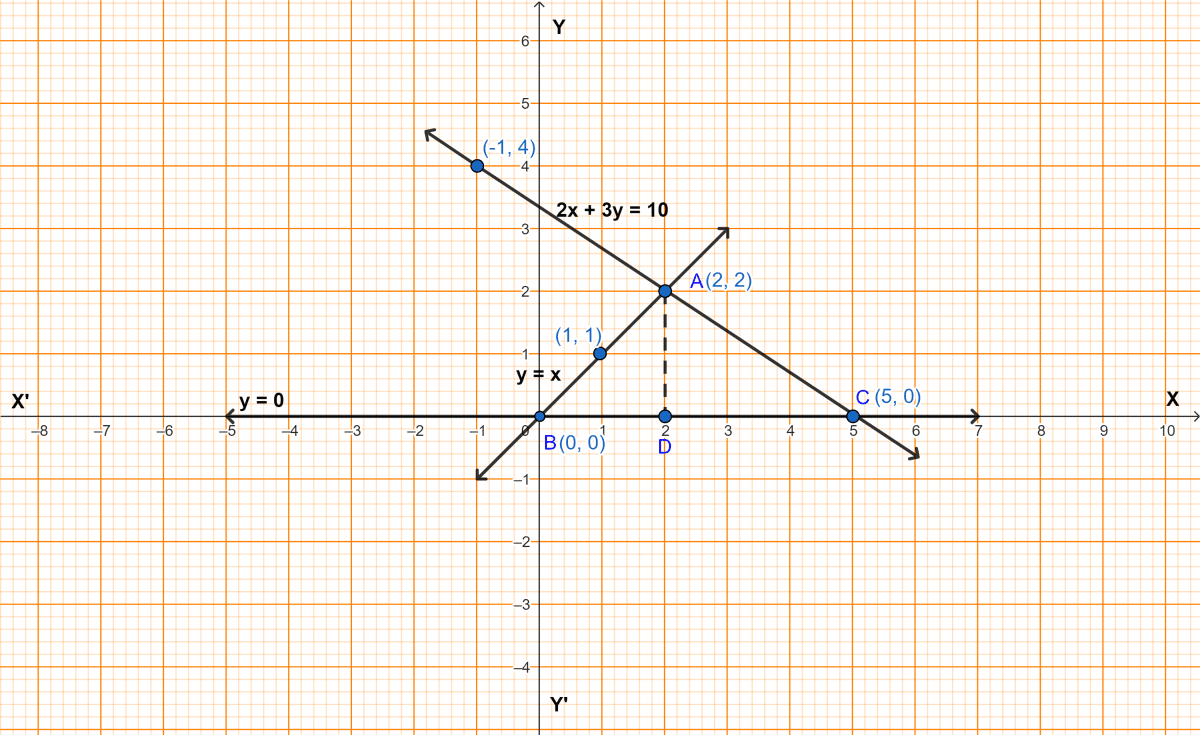
From graph,

ABC is the triangle.

From A, draw AD perpendicular to x-axis.

AD = 2 units and BC = 5 units.

Area of triangle = $\dfrac{1}{2}$ × base × height

= $\dfrac{1}{2} \times BC \times AD$

= $\dfrac{1}{2} \times 5 \times 2$

= 5 sq. units.

Hence, coordinates of triangle are (0, 0), (5, 0) and (2, 2) and area = 5 sq. units.