Mathematics
Describe the locus of points inside a circle and equidistant from two fixed points on the circumference of the circle.
Answer
Steps of construction :
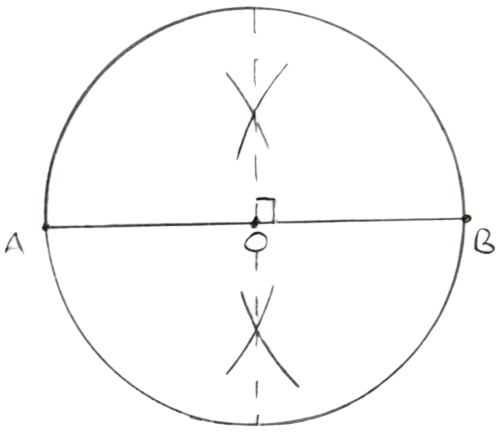
Draw a circle with O as center.
Mark two points A and B. Join AB.
Draw perpendicular bisector of AB. It should pass through center of the circle.
Since, O lies on perpendicular bisector of AB so OA = OB.
Hence, the locus of points inside the circle which are equidistant from the two fixed points on the circumference of a circle will be the diameter which is the perpendicular bisector of the chord joining the two fixed points on the circle.
Related Questions
Describe the locus of the door handle, as the door opens.
Describe the locus of a runner, running round a circular track and always keeping a distance of 1.5 m from the inner edge.
Describe the locus of the centers of all circles passing through two fixed points.
Describe the locus of vertices of all isosceles triangles having a common base.