Mathematics
Describe completely the locus of centre of a circle of radius 2 cm and touching a fixed circle of radius 3 cm with centre O.
Answer
From the figure,
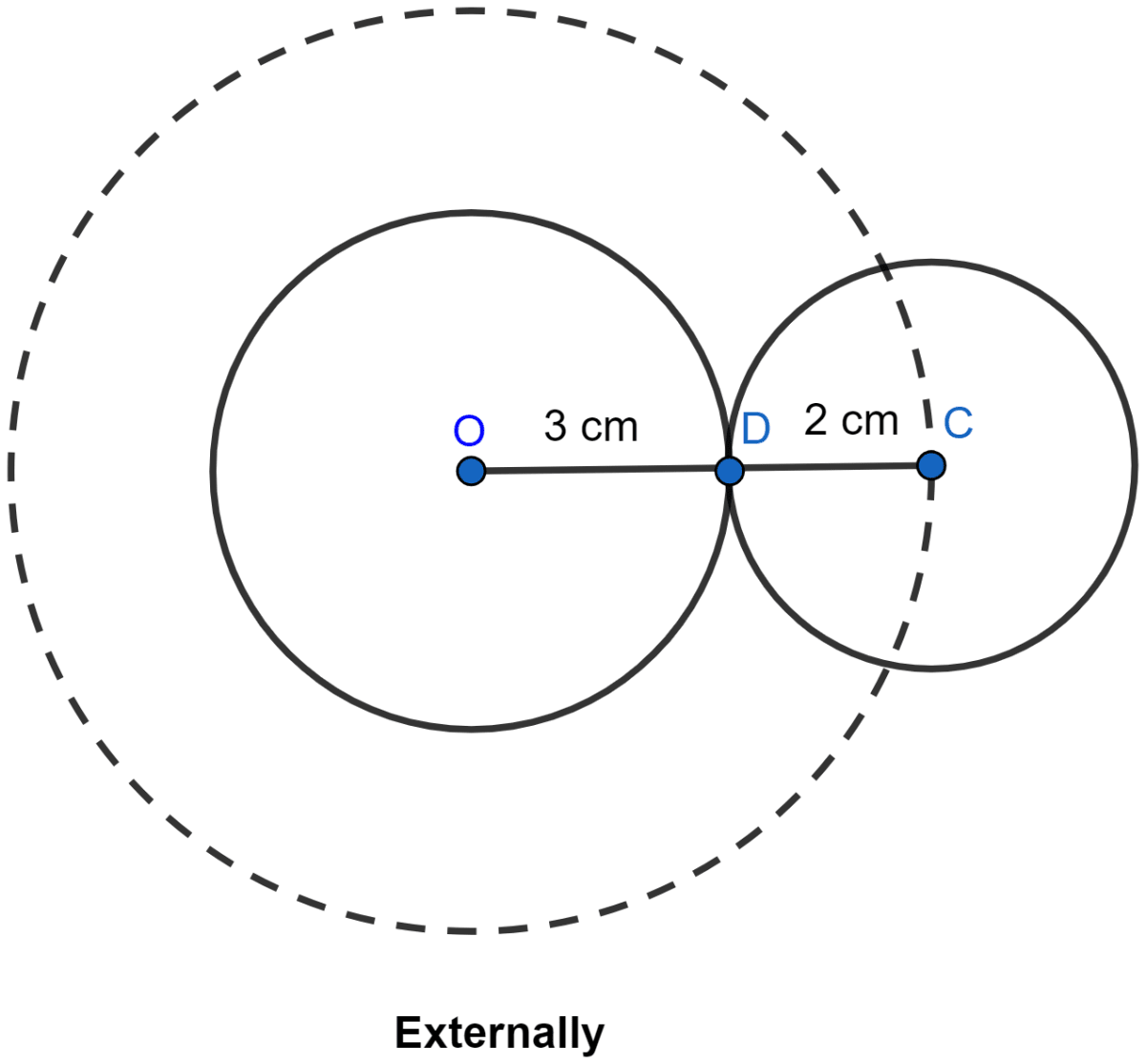
If the circle with 2 cm as radius touches the given circle externally then the locus of the centre of the circle will be a concentric circle with radius (3 + 2) = 5 cm.
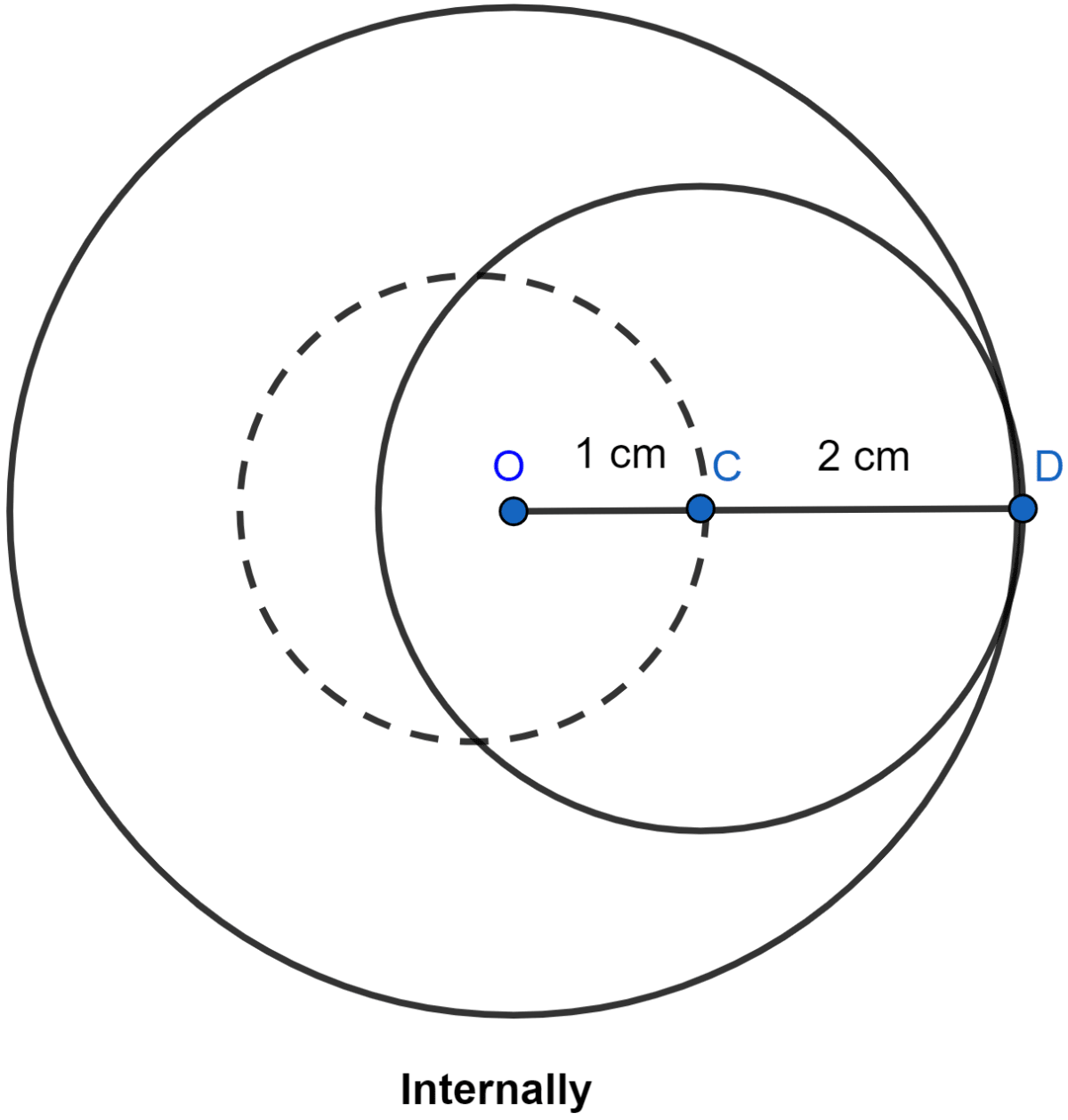
If the circle with 2 cm as radius touches the given circle with 3 cm as radius internally, then the locus of the centre of the circle will be a concentric circle with radius (3 - 2) = 1 cm.
Related Questions
Describe completely the locus of centre of a circle of varying radius and touching two arms of ∠ABC.
Describe completely the locus of point in a plane, at a constant distance of 5 cm from a fixed point (in the plane).
Using ruler and compasses, construct
(i) a triangle ABC in which AB = 5.5 cm, BC = 3.4 cm and CA = 4.9 cm.
(ii) the locus of points equidistant from A and C.
Construct triangle ABC, with AB = 7 cm, BC = 8 cm and ∠ABC = 60°. Locate by construction the point P such that :
(i) P is equidistant from B and C and
(ii) P is equidistant from AB and BC.
(iii) Measure and record the length of PB.