Mathematics
An observer 1.5 m tall is 20.5 meters away from a tower 22 metres high. Determine the angle of elevation of the top of the tower from the eye of the observer.
Heights & Distances
18 Likes
Answer
Let CD be an observer of height 1.5 m which is 20.5 m away from a tower AB of height 22 m.
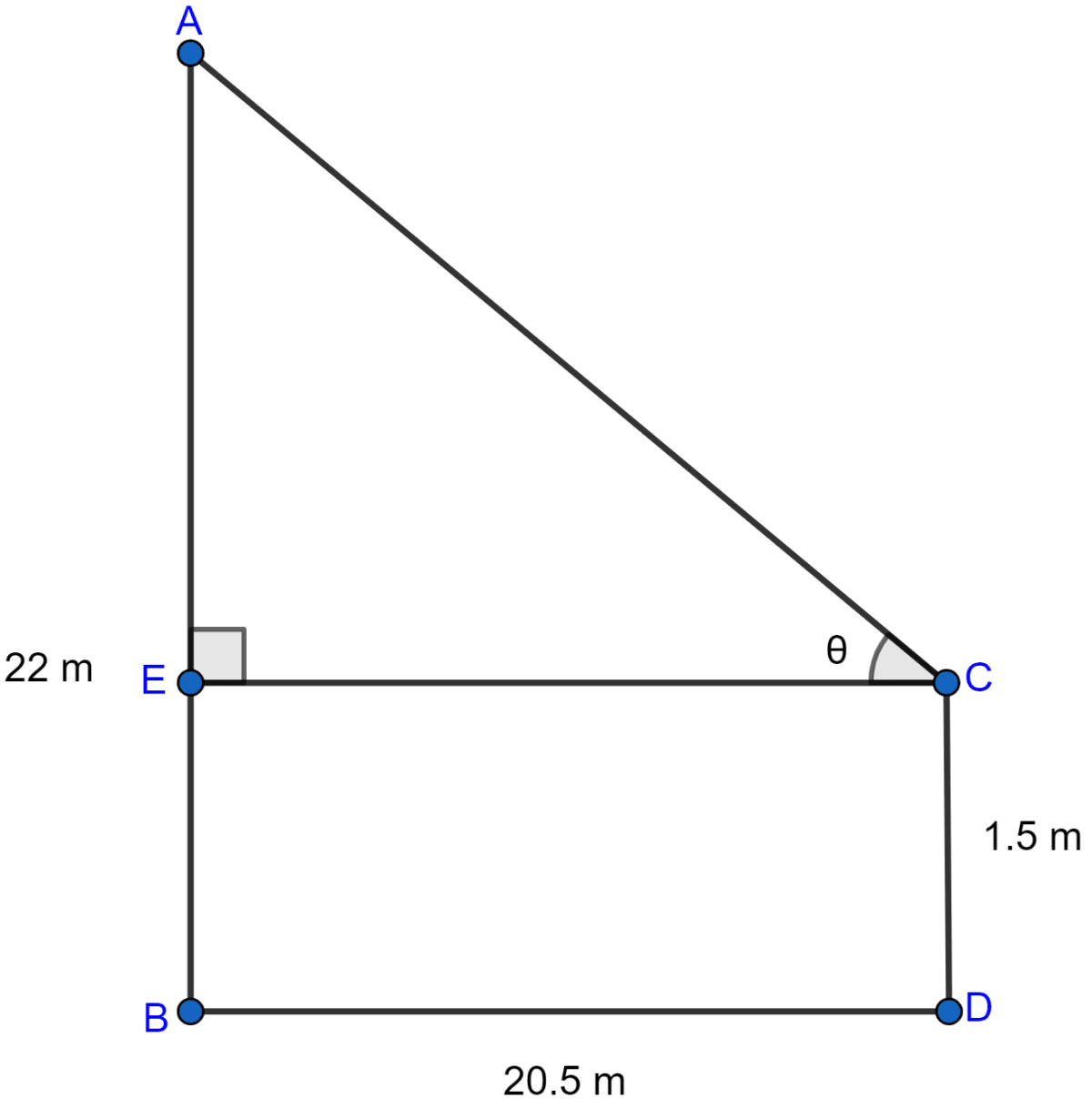
CD = 1.5 m and DB = 20.5 m
From C, draw CE ⊥ AB, then CDBE is a rectangle.
AE = AB - BE = AB - CD = 22 - 1.5 = 20.5 m
CE = DB = 20.5 m.
Let angle of elevation be θ,
From right angled △ACE, we get
Hence, the angle of elevation of the top of the tower from the eye of the observer is 45°.
Answered By
12 Likes
Related Questions
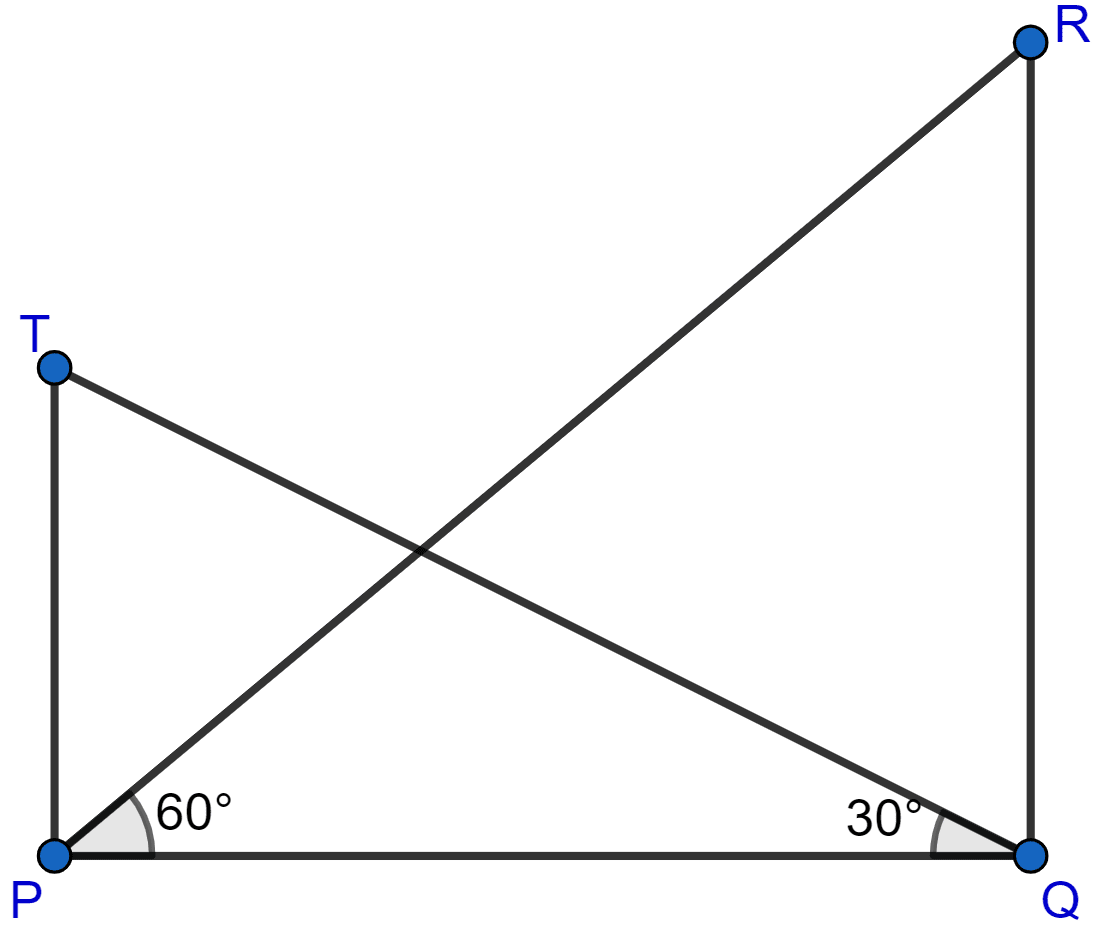
In the adjoining figure, the angle of elevation from a point P of the top of a tower QR, 50 m high is 60° and that of the tower PT from a point Q is 30°. Find the height of the tower PT, correct to the nearest metre.
From a point P on the ground, the angle of elevation of the top of a 10 m tall building and a helicopter, hovering over the top of the building are 30° and 60° respectively. Find the height of the helicopter above the ground.
A vertical tower is 20 m high. A man standing at some distance from the tower knows that the cosine of the angle of elevation of the top of the tower is 0.53. How far is he standing from the foot of the tower ?
The upper part of a tree broken by wind, falls to the ground without being detached. The top of the broken part touches the ground at an angle of 38° 30' at a point 6 m from the foot of the tree. Calculate :
(i) the height at which the tree is broken.
(ii) the original height of the tree correct to two decimal places.