Mathematics
In the adjoining figure, the angle of elevation from a point P of the top of a tower QR, 50 m high is 60° and that of the tower PT from a point Q is 30°. Find the height of the tower PT, correct to the nearest metre.
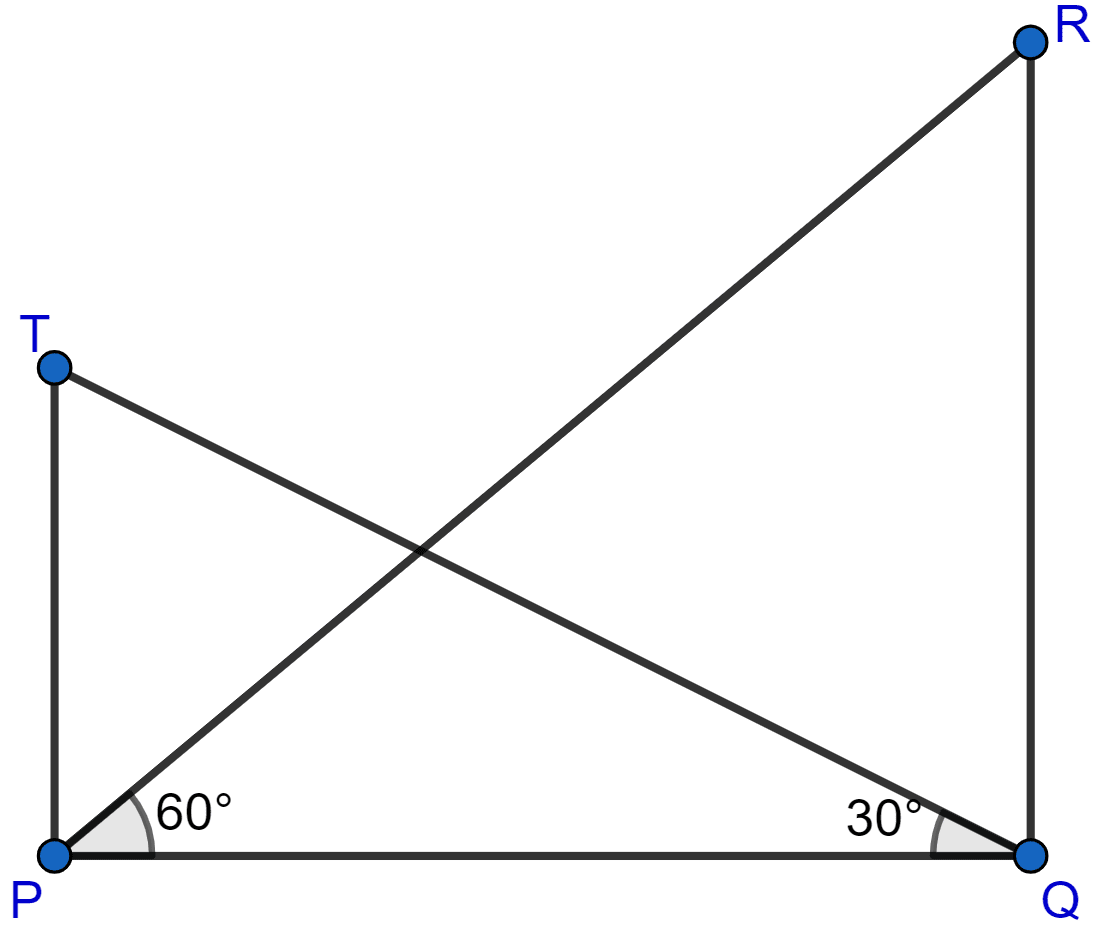
Heights & Distances
49 Likes
Answer
Let height of tower PT be h meters.
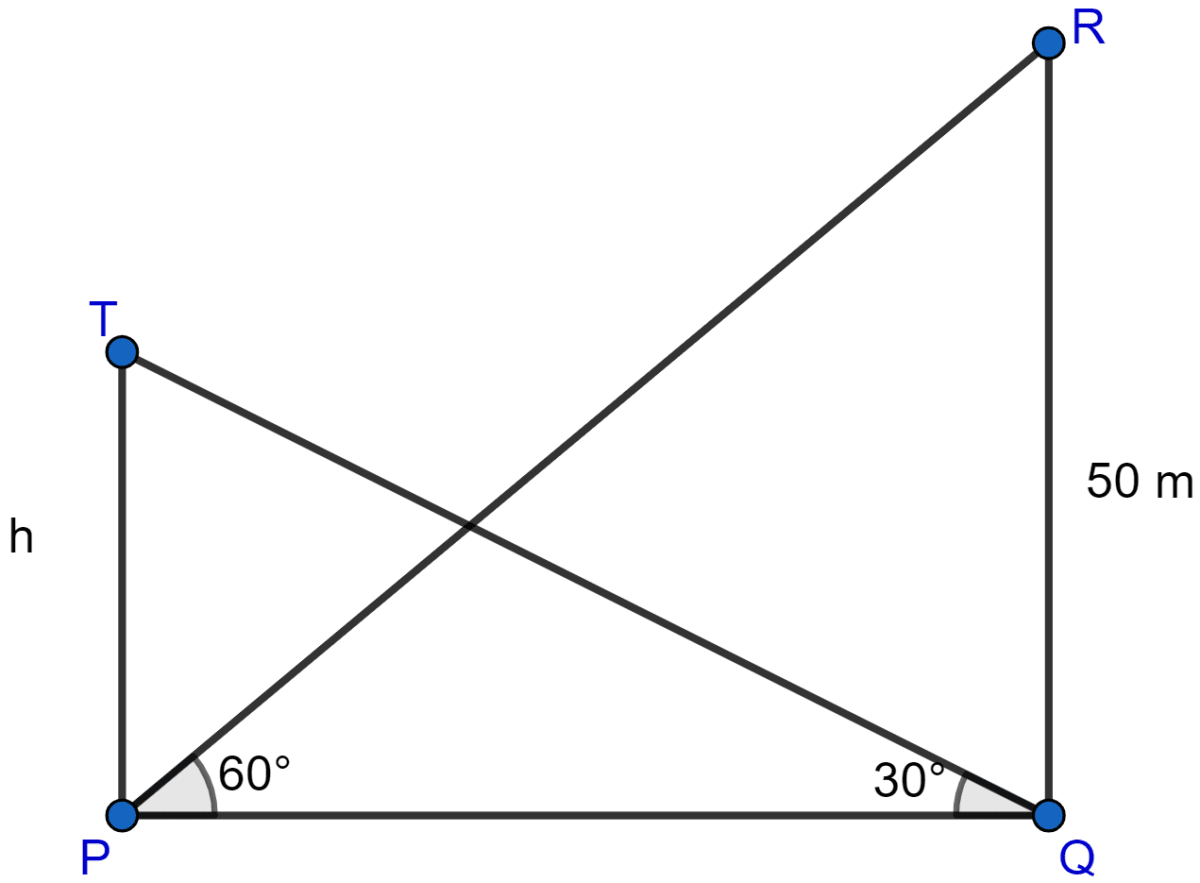
Considering right angled △PQR, we get
Now considering right angled △PQT, we get
On correcting to nearest meter, h = 17 m.
Hence, the height of the tower PT = 17 m.
Answered By
27 Likes
Related Questions
The upper part of a tree broken by wind, falls to the ground without being detached. The top of the broken part touches the ground at an angle of 38° 30' at a point 6 m from the foot of the tree. Calculate :
(i) the height at which the tree is broken.
(ii) the original height of the tree correct to two decimal places.
An observer 1.5 m tall is 20.5 meters away from a tower 22 metres high. Determine the angle of elevation of the top of the tower from the eye of the observer.
From a point P on the ground, the angle of elevation of the top of a 10 m tall building and a helicopter, hovering over the top of the building are 30° and 60° respectively. Find the height of the helicopter above the ground.
An aeroplane when flying at a height of 3125 m from the ground passes vertically below another plane at an instant when the angles of elevation of the two planes from the same point on the ground are 30° and 60° respectively. Find the distance between the two planes at the instant.