Mathematics
AD is perpendicular to the side BC of an equilateral △ABC. Prove that 4AD2 = 3AB2.
Pythagoras Theorem
48 Likes
Answer
Given, AD ⊥ BC and AB = BC = CA (Equilateral triangle).
The perpendicular to base in equilateral triangle bisects the base.
∴ BD = .
From figure,
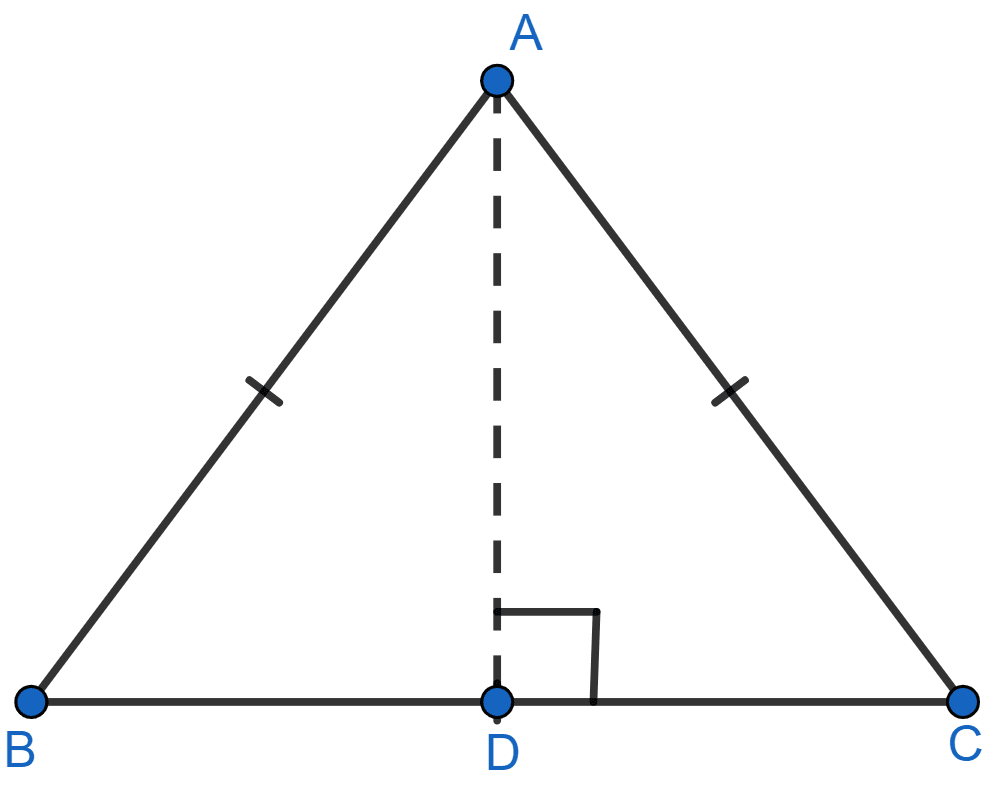
In right triangle ABD,
By pythagoras theorem,
⇒ AB2 = AD2 + BD2
⇒ AB2 = AD2 +
⇒ AB2 = AD2 +
⇒ AB2 =
⇒ 4AB2 = 4AD2 + BC2
⇒ 4AB2 = 4AD2 + AB2 (∵ BC = AB)
⇒ 4AD2 = 3AB2.
Hence, proved that 4AD2 = 3AB2.
Answered By
37 Likes
Related Questions
In figure given below, AB || DC, ∠A = 90°, DC = 7 cm, AB = 17 cm and AC = 25 cm. Calculate BC.

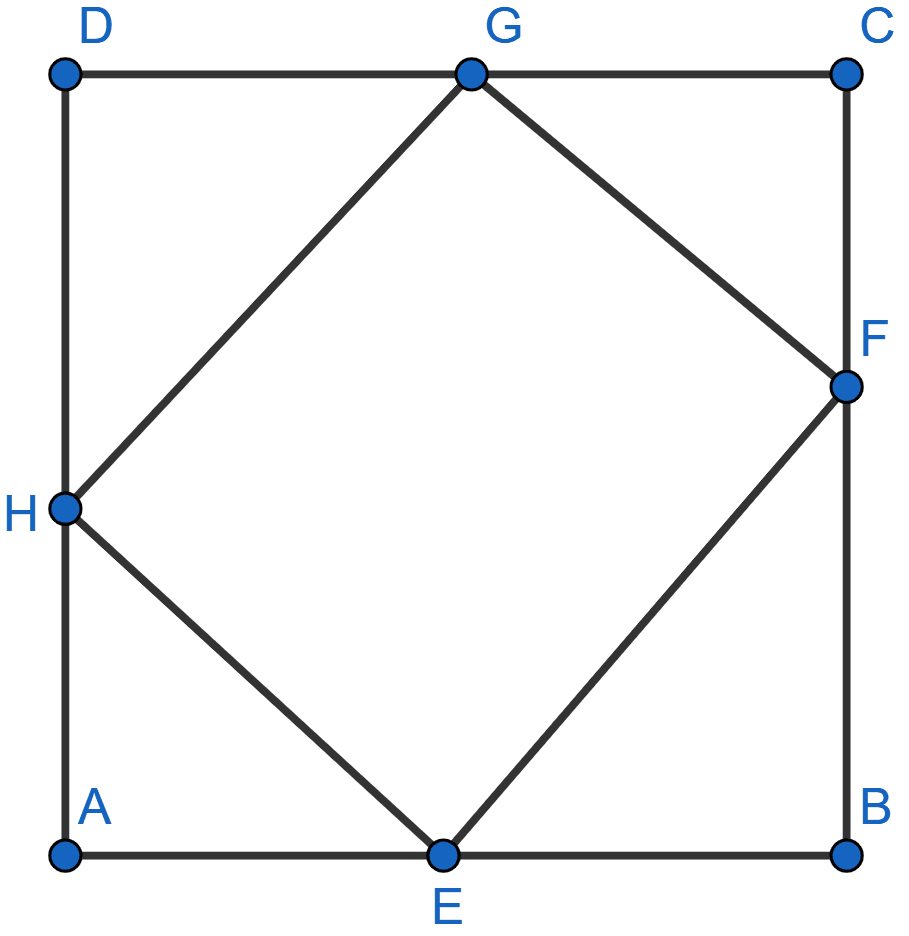
In the figure given below, ABCD is a square of side 7 cm. If
AE = FC = CG = HA = 3 cm,
(i) prove that EFGH is a rectangle.
(ii) find the area and perimeter of EFGH.
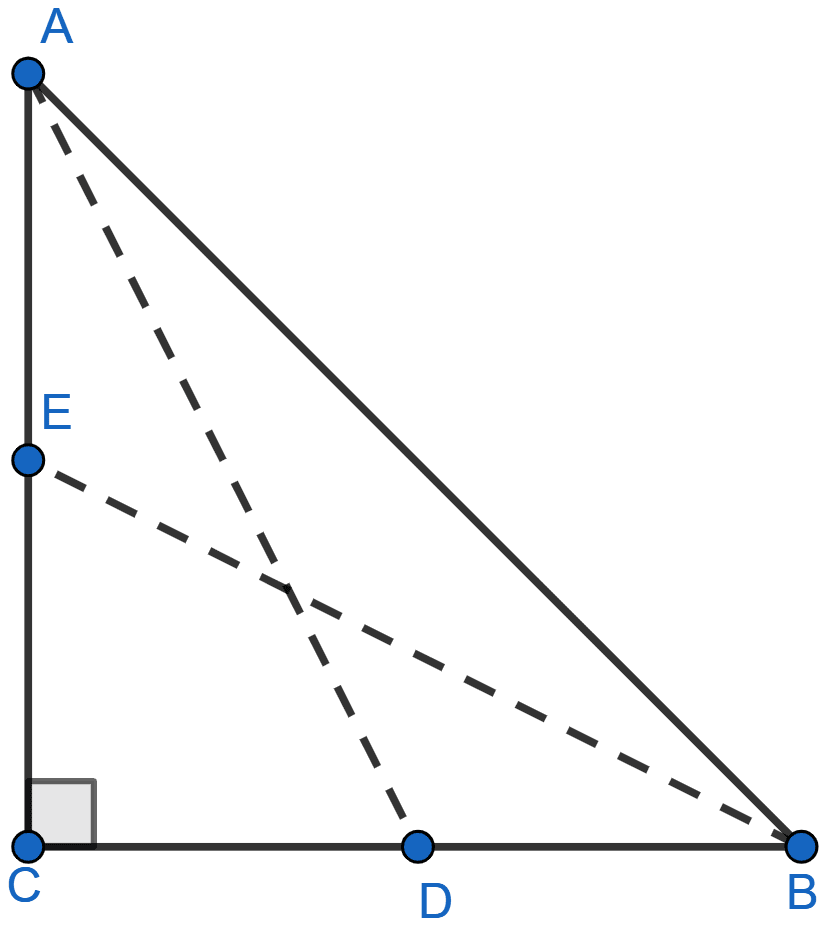
In the adjoining figure, D and E are mid-points of the sides BC and CA respectively of a △ABC, right angled at C. Prove that :
(i) 4AD2 = 4AC2 + BC2
(ii) 4BE2 = 4BC2 + AC2
(iii) 4(AD2 + BE2) = 5AB2
If AD, BE and CF are medians of △ABC, prove that
3(AB2 + BC2 + CA2) = 4(AD2 + BE2 + CF2).