Mathematics
ABCD is a parallelogram where A(x, y), B(5, 8), C(4, 7) and D(2, -4). Find
(i) the coordinates of A.
(ii) the equation of the diagonal BD.
Straight Line Eq
61 Likes
Answer
(i) Let O be the point of intersection of the diagonals.
So, O will be the mid-point of the diagonals so also the mid-point of BD.
By mid-point formula coordinates of O are,
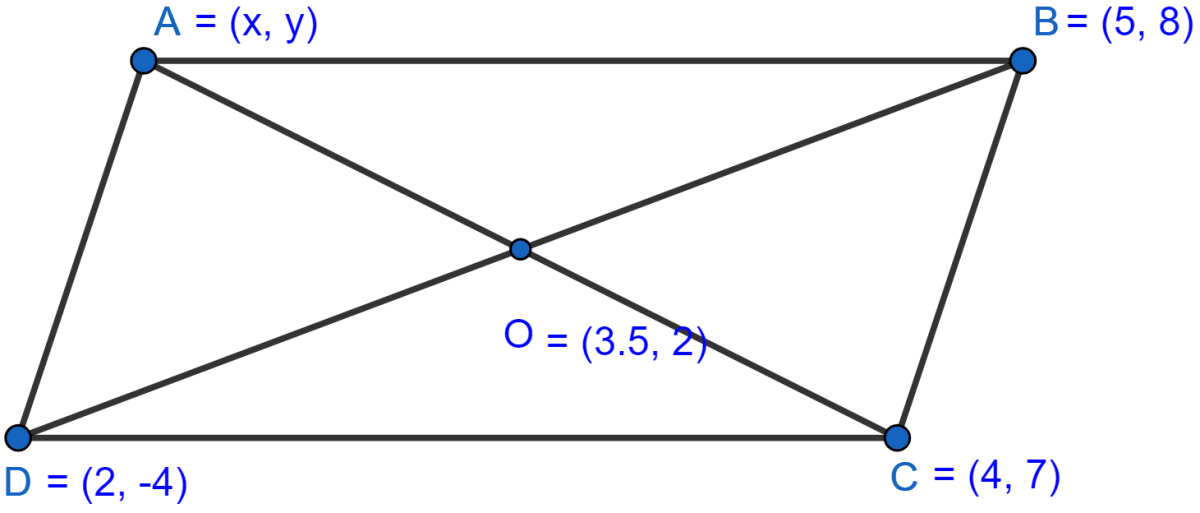
Since, O is also the mid-point of AC so,
Hence, the coordinates of A are (3, -3).
(ii) Equation of diagonal BD can be given by two point formula,
1 = \dfrac{y2 - y1}{x2 - x1}(x - x1)
Putting values in above equation,
Hence, the equation of the diagonal BD is 4x - y - 12 = 0.
Answered By
25 Likes
Related Questions
Find the value of 'a' for which the following points A(a, 3), B(2, 1) and C(5, a) are collinear. Hence, find the equation of the line.
Use a graph paper for this question. The graph of a linear equation in x and y, passes through A (-1, -1) and B (2, 5). From your graph, find the values of h and k, if the line passes through (h, 4) and (, k).
In △ABC, A (3, 5), B (7, 8) and C (1, -10). Find the equation of the median through A.
Find the equation of a line passing through the point (-2, 3) and having x-intercept 4 units.