Mathematics
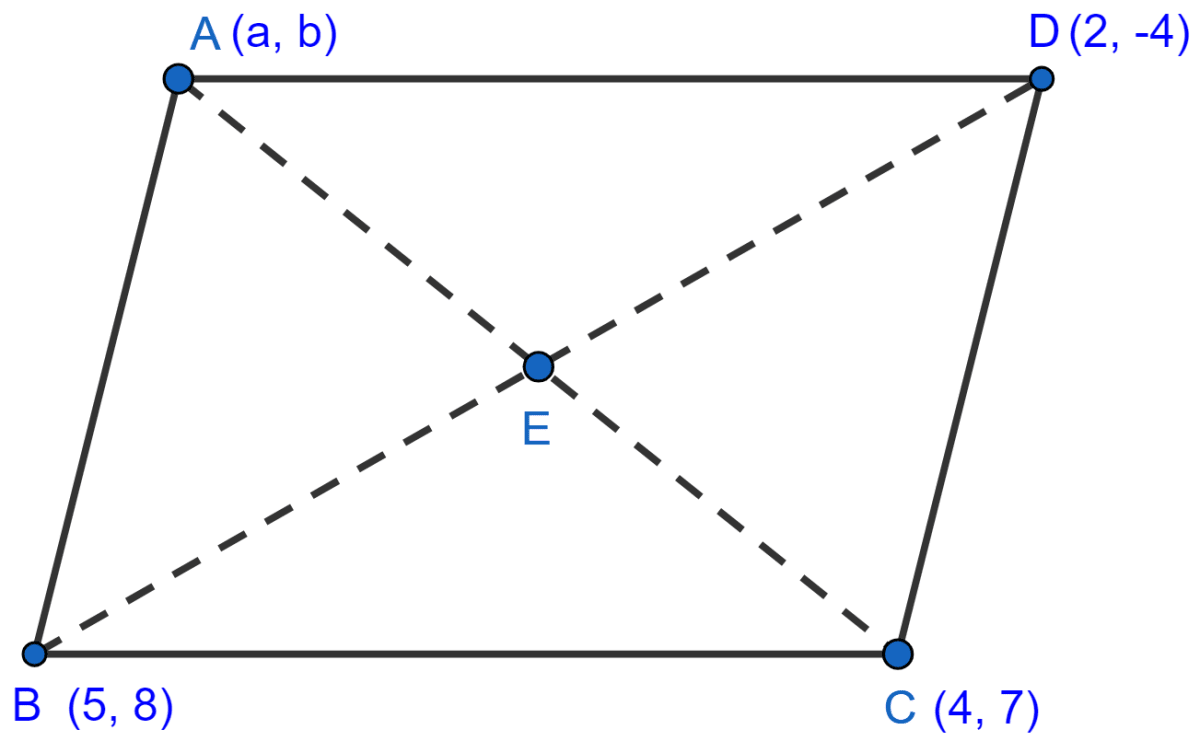
ABCD is a parallelogram in which vertices B = (5, 8), C = (4, 7) and D = (2, -4). Find :
(i) the coordinates of vertex A.
(ii) the equation of diagonal BD.
Straight Line Eq
2 Likes
Answer
(i) We know that,
Diagonals of parallelogram bisect each other.
Let E be the point of intersection of diagonals.
So, E will be the mid-point of BD as well as of AC.
By mid-point formula,
Mid-point (M) = 1 + x2}{2}, \dfrac{y1 + y2}{2}\Big)
So, mid-point of BD = .
E is mid-point of AC as well. Let coordinates of A be (a, b).
A = (a, b) = (3, -3).
Hence, coordinates of A are (3, -3).
(ii) By two point form,
Equation of a line : y - y1 = 2 - y1}{x2 - x1}(x - x_1)
Substituting values we get :
Equation of BD :
Hence, equation of BD is y = 4x - 12.
Answered By
1 Like
Related Questions
Prove that :
.
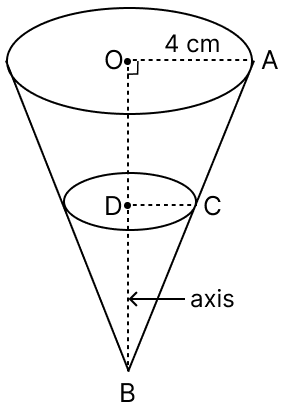
A cone of radius 4 cm is divided into two parts by drawing a plane through the mid-point of its axis and parallel to its base. Compare the volumes of the two parts.
On a graph paper plot the triangle ABC whose vertices are at points A(5, 4), B(7, 5) and C(-3, 6). On the same graph, draw the image of the triangle ABC under reflection in the line y = 3. Mark any two points on the graph paper which are invariant under this reflection. Also, write the co-ordinates of points marked.
Rita went to shop to purchase an article A with MRP = ₹ 850 and rate of GST = 12%. How much will Rita pay for this article?
If instead of article A, Rita purchases some other article B with MRP = ₹ 1200 and rate of GST = 18%, find how much money will she pay to the shopkeeper ?