Mathematics
ABCD is a parallelogram, bisectors of angles A and B meet at E which lies on DC. Prove that AB = 2AD.
Rectilinear Figures
28 Likes
Answer
ABCD is a parallelogram in which bisector of ∠A and ∠B meets DC at E.
To prove: AB = 2AD
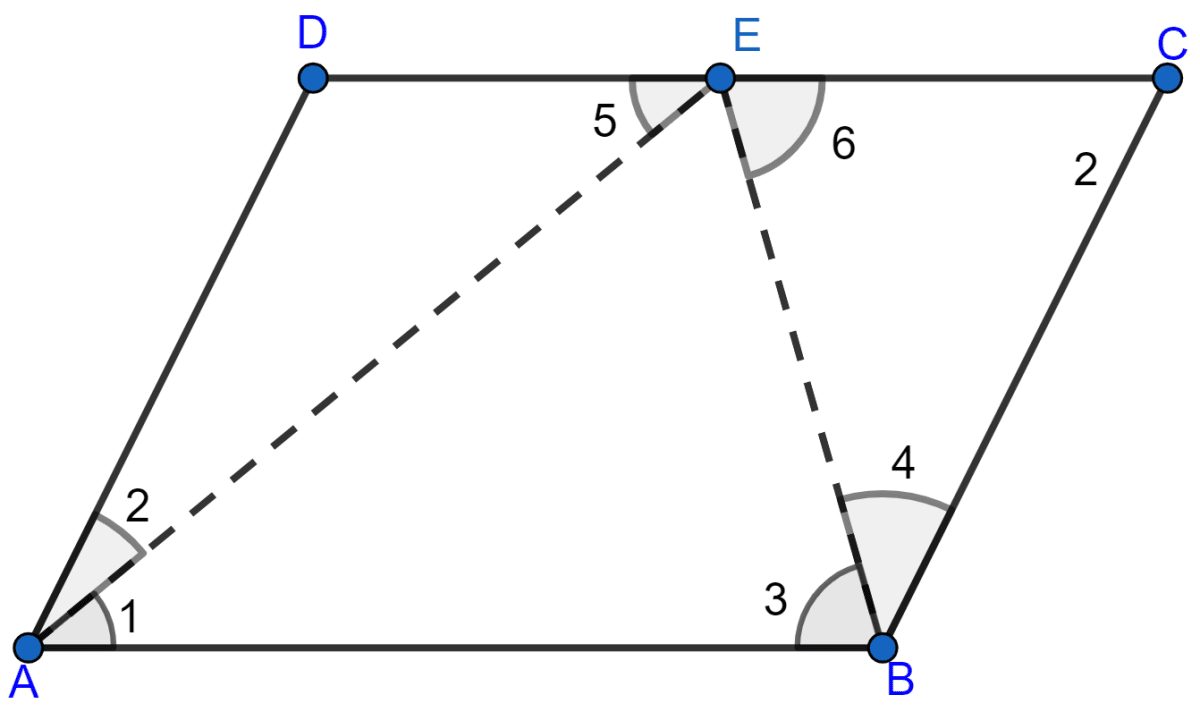
Since, AE and BE are bisector of ∠A and ∠B
∠1 = ∠2 and ∠3 = ∠4
In parallelogram ABCD, we have
AB || DC
∠1 = ∠5 [Alternate angles are equal, AE is transversal]
Thus,
∠2 = ∠5 … (i)
∴ DE = AD [∵ Sides opposite to equal angles in ∆AED]
∠3 = ∠6 [Alternate angles]
∠3 = ∠4 [Since, BE is bisector of ∠B (given)]
Thus, ∠4 = ∠6 … (ii)
∴ BC = EC [∵ Sides opposite to equal angles in ∆BCE]
AD = BC [Opposite sides of || gm are equal]
AD = DE = EC
AB = DC [Opposite sides of a || gm are equal]
⇒ AB = DE + EC
⇒ AB = AD + AD
⇒ AB = 2AD
Hence, proved that AB = 2AD.
Answered By
22 Likes
Related Questions
ABCD is a square and the diagonals intersect at O. If P is a point on AB such that AO = AP, prove that 3∠POB = ∠AOP.
A transversal cuts two parallel lines at A and B. The two interior angles at A are bisected and so are the two interior angles at B; the four bisectors form a quadrilateral ACBD. Prove that
(i) ACBD is a rectangle.
(ii) CD is parallel to the original parallel lines.
In parallelogram ABCD, the bisector of ∠A meets DC in E and AB = 2AD. Prove that
(i) BE bisects ∠B
(ii) ∠AEB = a right angle.
ABCD is a square. E, F, G and H are points on the sides AB, BC, CD and DA respectively such that AE = BF = CG = DH. Prove that EFGH is a square.