Mathematics
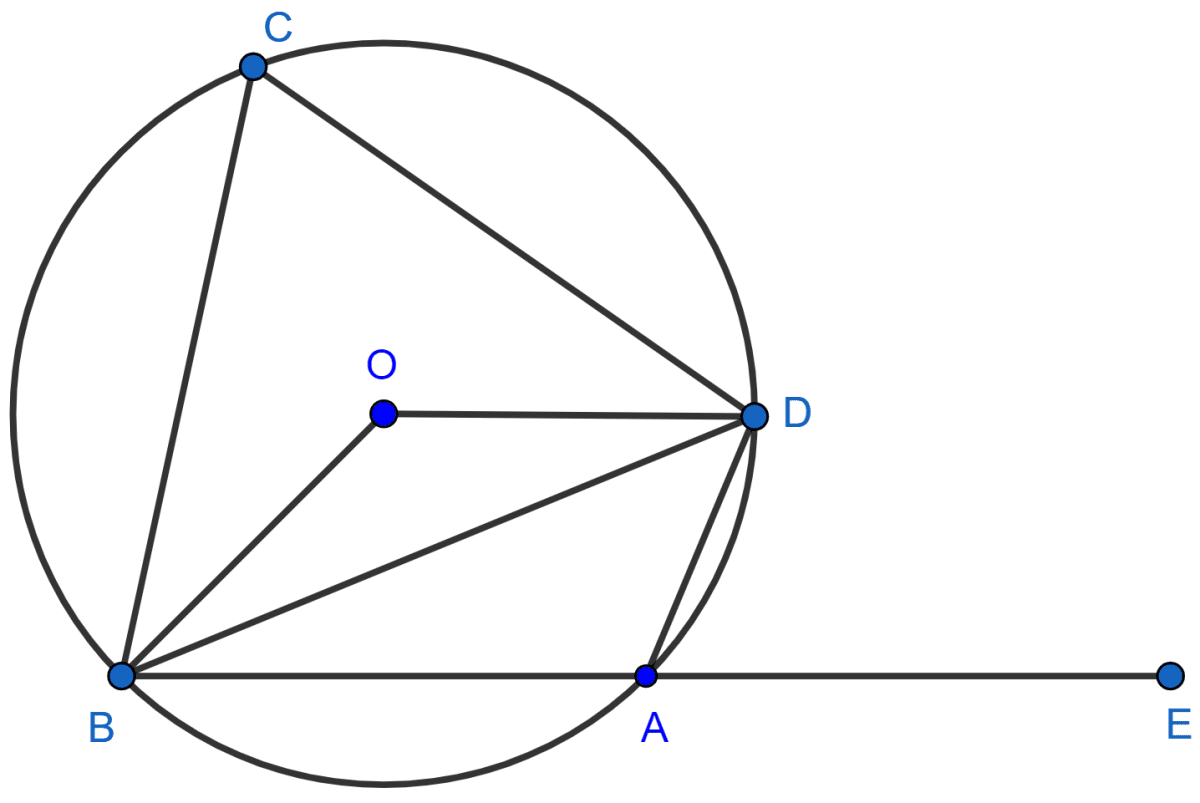
ABCD is a cyclic quadrilateral of a circle with center O such that AB is a diameter of this circle and the length of the chord is equal to the radius of the circle. If AD and BC produced meet at P, show that APB = 60°.
Circles
2 Likes
Answer
From figure,
CO = DO = CD [Radius of circle]
∴ COD is an equilateral triangle.
∴ ∠DOC = ∠ODC = ∠DCO = 60°.
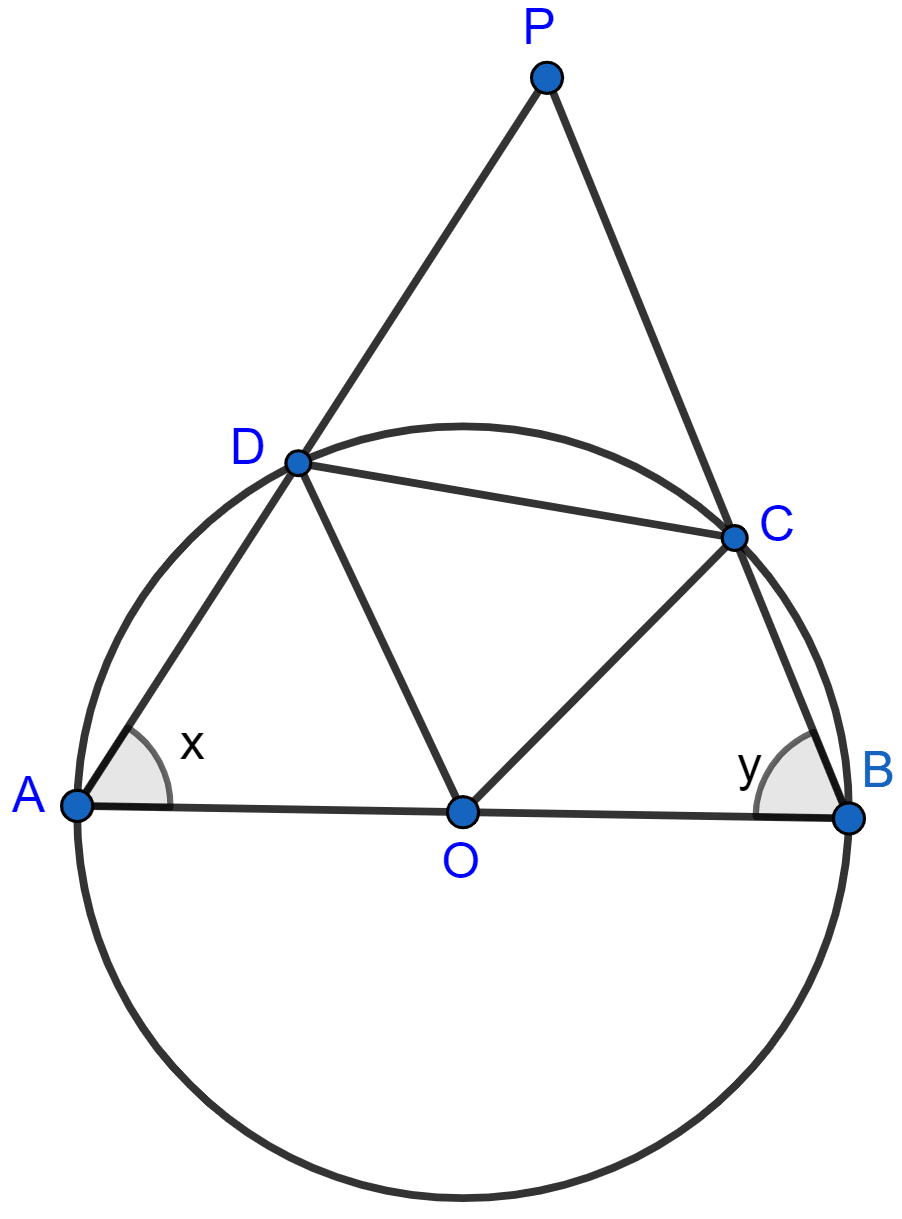
Let ∠A = x and ∠B = y
OA = OB = OC = OD [Radius of circle]
As angles opposite to equal sides are equal.
∴ ∠ODA = ∠OAD = x
and
∠OCB = ∠OBC = y.
In △OAD,
⇒ ∠ODA + ∠OAD + ∠AOD = 180°
⇒ x + x + ∠AOD = 180°
⇒ ∠AOD = 180° - 2x.
In △BOC,
⇒ ∠OBC + ∠OCB + ∠BOC = 180°
⇒ y + y + ∠BOC = 180°
⇒ ∠BOC = 180° - 2y.
Since, AOB is a straight line
⇒ ∠AOD + ∠BOC + ∠DOC = 180°
⇒ 180° - 2x + 180° - 2y + 60° = 180°
⇒ 2x + 2y = 420° - 180°
⇒ 2x + 2y = 240°
⇒ x + y = 120°.
In △ABP,
⇒ ∠A + ∠B + ∠P = 180°
⇒ x + y + ∠P = 180°
⇒ 120° + ∠P = 180°
⇒ ∠P = 180° - 120° = 60°.
From figure,
∠APB = ∠P = 60°.
Hence, ∠APB = 60°.
Answered By
1 Like
Related Questions
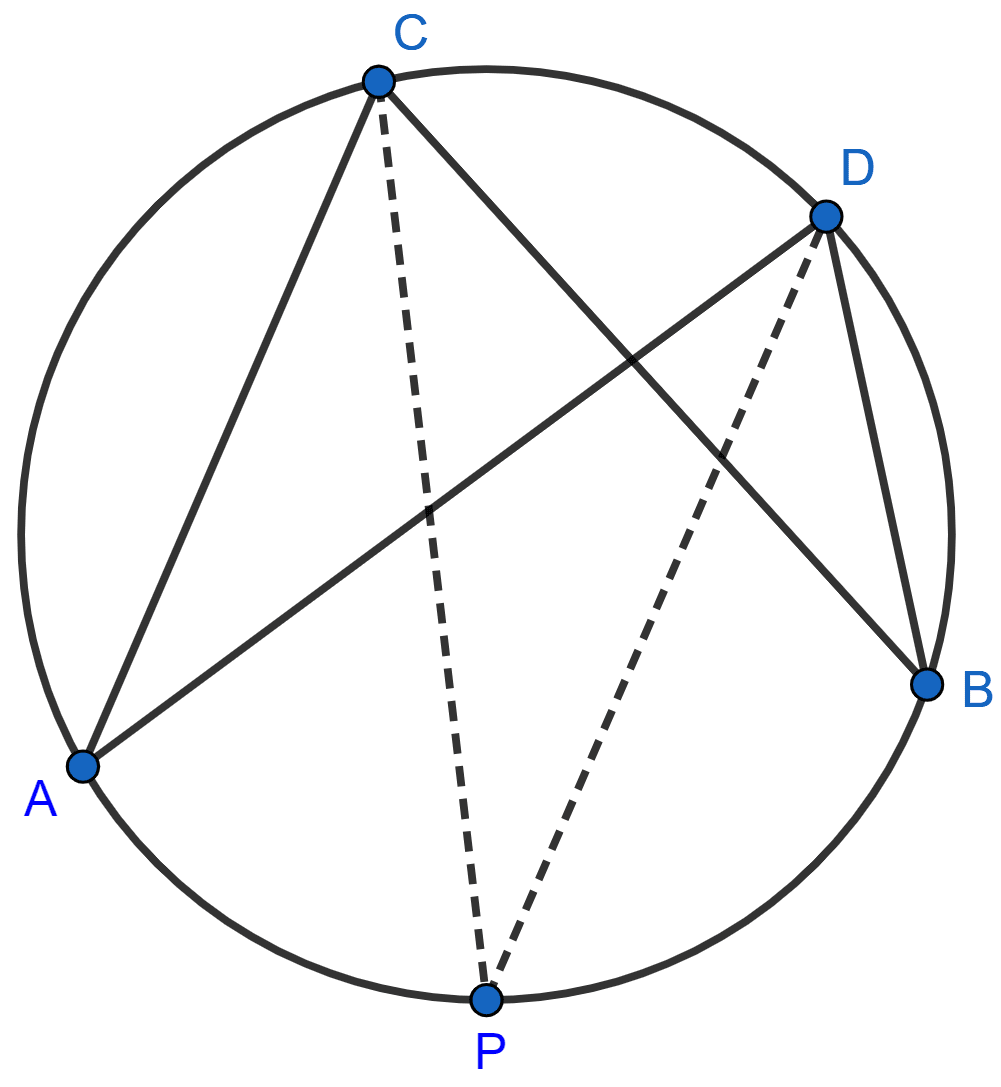
In the figure, given below, CP bisects angle ACB. Show that DP bisects angle ADB.
In the figure, given below, AD = BC, ∠BAC = 30° and ∠CBD = 70°. Find :
(i) ∠BCD
(ii) ∠BCA
(iii) ∠ABC
(iv) ∠ADB

In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°. Find :
(i) ∠OBD
(ii) ∠AOB
(iii) ∠BED

In the figure given, O is the centre of the circle. ∠DAE = 70°. Find giving suitable reasons, the measure of
(i) ∠BCD
(ii) ∠BOD
(iii) ∠OBD